Algebra 2 Volume 1 1st Edition Module 4 Quadratic Equations And System Of Equations
Page 203 Problem 1 Answer
It is given three equations having three variables.
It is required to show that how to find the solutions to the given equation.
To find the solution of the equation find the value of one of the variables in form of the other two.
Then substitute it on the other two-equation to get the equation in two variables. After that use linear algebra to solve the equation in two variables.
Substitute the obtained values in the previous equation to get the value of substituted variables.
The value of all variables is obtained.
Page 203 Problem 2 Answer
Three figures are given in the question.
It is required to determine how many points lie on all three given planes.
For figure one all the plans are parallel to each other hence there is no such points which is common between three planes.
For figure two here two planes are parallel which and one plan intersect other at different line hence plane hence no such points are there.
For figure three plans are not parallel but all each plan intersects at different lines, hence no such point exists in this case.
None of the given has set of points which lies in all the three plans.
Page 203 Problem 3 Answer
A figure is given in the question.
It is required to determine how many points lie on all three given planes.
All the plans are perpendicular to each other and intersect at only one point. Hence there is only one point common in all three plans.
There is only one point common in all three plans.
Page 203 Problem 4 Answer
A figure is given in the question.
It is required to determine how many points lie on all three given planes.
In the given figure arrange in such a manner that they intersect at a common line,
Hence all the points which satisfy the equation of line of intersection will lies in all three planes.
Three plans intersect along a common line of intersection.
All the points which satisfy the line of interaction will be lies on all the three planes.
Page 204 Problem 5 Answer
It is given that the vertical parabola equation is given by y=ax2+bx+c.
It is required to find the parabola that passes through the points (2,1),(−1,4) and (−2,3).
Substitute the coordinates of point into the general equation y=ax2+bx+c to get three equations. Solve the equations for three variables x,y and z.
Put the obtained values back in the general equation to get the required parabola equation.
Substitute (2,1),(−1,4) and (−2,3) in the equation y=ax2+bx+c to obtain three equations.
1=a(2)2+b(2)+c
4=a(−1)2+b(−1)+c
3=a(−2)2+b(−2)+c
Simplify the equations.
1=4a+2b+c
4=a−b+c
3=4a−2b+c
From third equation, c=4−a+b. Put the obtained value in other equations.
3a+3b=−3
3a−b=−1
Solve the two equations by substituting b=3a+1 in 3a+3b=−3.
3a+3(3a+1)=−3
3a+9a+3=−3
12a=−6
a=−1/2
So, the value of b is −3/2+1=−1/2.
The value of c is,
c=4−(−1/2)+(−1/2)
=4+1/2−1/2
=4
Therefore, the equation of parabola is,
y=(−1/2)x2+(−1/2)x+4−2y=x2+x−8
x2+x+2y−8=0
The equation of the vertical parabola passing through the points (2,1),(−1,4) and (−2,3)
is x2+x+2y−8=0.
Page 204 Problem 6 Answer
t is required to give an example of three planes that intersect at exactly one point.
One such combination of the plane is xy−plane,yz−plane and zx−plane which intersect at only one point that is (0,0,0).
So, xy−plane,yz−plane and zx−plane which intersect at only one point that is (0,0,0).
Page 206 Problem 6 Answer
It is given three equations, x+2y+z=8 ……eq(1)
2x+y−z=4 ……eq(2)
x+y+3z=7 ……eq(3)
It is required to find the value of x,y and z for the given equations.
To solve this, substitute the value of y from eq (2) on eq(1) and eq(3) to get the equations in two variables.
Then solve the equations of two variables by using linear algebra.
After that, find the value y with the help of x and z obtained previously.
It is given three equations,
x+2y+z=8 ……eq(1)
2x+y−z=4 ……eq(2)
x+y+3z=7 ……eq(3)
From eq(2),
2x+y−z=4
y=4−2x+z
Substituting the value of y on eq(1) equation become,
x+2y+z=8
x+2(4−2x+z)+z=8
x+8−4x+2z+z=8
−3x+3z=0
−x+z=0
Therefore, x=z ……eq (4)
Substituting the value of y on eq (3) equation become,
x+y+3z=7
x+(4−2x+z)+3z=7
−x+4z=3
From, eq(4)
−z+4z=3
3z=3
z=1
So, x=1
Now substituting the value of x and z on the equation of y,
y=4−2x+z
y=4−2+1
y=3
So, the values are
x=1
y=3
z=1
Therefore, the value of x,y and z for the given equations are,
x=1
y=3
z=1
Page 206 Problem 7 Answer
It is given three equations, 2x−y−3z=1 ……eq(1)
4x+3y+2z=−4 ……eq(2)
−3x+2y+5z=−3 ……eq(3)
It is required to find the value of x,y and z
for the given equations.
To solve this, substitute the value of y from eq(1) on eq(2) and eq(3) to get the equations in two variables.
Then, solve the equations of two variables by using linear algebra.
After that, find the value y with the help of x and z obtained previously.
It is given three equations,
2x−y−3z=1 ……eq(1)
4x+3y+2z=−4 ……eq(2)
−3x+2y+5z=−3 ……eq(3)
From eq(1),
2x−y−3z=1
y=2x−3z−1
Substituting the value of y on eq(2) equation become,
4x+3y+2z=−4
4x+3(2x−3z−1)+2z=−4
4x+6x−9z−3+2z=−4
10x−7z=−4
Thus, 7z−10x=4 ……eq(4)
Substituting the value of y on eq(3) equation become,
−3x+2y+5z=−3
−3x+2(2x−3z−1)+5z=−3
x−z=−1
Thus, x−z=1 ……eq(5)
Adding ten times eq (5) to eq (4)
−3z=14
z=−14/3
From, eq(5)
x=1+z
x=1−14/3
x=−11/3
Now, for y
y=2x−3z−1
y=2⋅(−11/3)−3⋅(−14/3)−1
Simplify it to get the result,
y=−22/3+42/3−1
y=−22+42−3/3
y=17/3
The values of x,y, and z for the given equations are,
x=−11/3
y=17/3
z=−14/3
Page 207 Problem 8 Answer
It is given that the system of equations is
{ x+2y+3z =9 (1)
{ x+3y+2z =5 (2)
{ x+4y−z =−5 (3).
It is required to solve the system for three variables x,y, and z.
Subtract the second equation from the first equation to eliminate x.
Now subtract the third equation from the first equation to eliminate x.
Solve these two equations for y and z.
Now find the value of x by putting the values of y and z.
Start by subtracting equation 2 from equation 1 to eliminate x
x+3y+2z−(x+2y+3z)=5−9
3y+2z−2y−3z=−4
y−z=−4
Subtract equation 3 from 1 to eliminate x.
x+4y−z−(x+2y+3z)=−5−9
4y−z−2y−3z=−14
y−2z=−7
This results in a system of two linear equations.
y−z=−4
y−2z=−7
Solve the two equations for y and z.
y−z−y+2z=−4+7
z=3
Find the value of y.
y=z−4
=3−4
=−1
Find the value of x.
x+2(−1)+3(3)=9
x−2+9=9
x=2
The solution of the system
{ x+2y+3z=9 (1)
{ x+3y+2z =5 (2)
{ x+4y−z =−5 (3)
is the ordered triple (2,−1,3).
Page 209 Problem 9 Answer
It is given three equations,
x+2y+z=8…..eq(1)
2x+y−z=4…..eq(2)
x+y+3z=7…..eq(3)
It is required to solve the equation by the elimination method.
To solve this, add eq(1) with eq(2) then a relation between x and y are obtained. Then substitute the obtained value on eq(3) to get the value of z.
Given equations are,
x+2y+z=8…..eq(1)
2x+y−z=4…..eq(2)
x+y+3z=7…..eq(3)
Add eq(1) with eq(2).
3x+3y=12
3(x+y)=12
x+y=4…..eq(5)
Now, substituting the obtained value in eq(3)
x+y+3z=7
4+3z=7
3z=3
Divide both sides by 3.
z=1
Substituting the value of z on eq(1)
x+2y+1=8
x+2y=7…..eq(6)
Subtracting eq(5) with eq(6)
y=3
Substituting the value of y on eq(5)
x+y=4
x+3=4
Subtract 3 from both sides.
x+3−3=4−3
x=1
Values of the variable after elimination method are,
x=1
y=3
z=1
Page 209 Problem 10 Answer
It is given three equations,
2x−y−3z=1…..eq(1)
4x+3y+2z=−4…..eq(2)
−3x+2y+5z=−3…..eq(3)
It is required to solve the equation by the elimination method.
To solve this, add three times eq(1) with eq(2) then a relation between x and z are obtained.
Again, add two times eq(1) with eq(3) then another relation between x and z are obtained. Now solve the obtained equation to get the value of x and z.
Then substitute the obtained values on eq(3) to get the value of y.
Given equations are,
2x−y−3z=1…..eq(1)
4x+3y+2z=−4…..eq(2)
−3x+2y+5z=−3…..eq(3)
Adding three times eq(1) with eq(2)
10x−7z=−1…..eq(4)
Adding two times eq(1) with eq(3)
x−z=−1…..eq(5)
For, solving subtract 7 times eq(5) from eq(4)
3x=6
x=2
Substituting the value of x on eq(5)
x−z=−1
2−z=−1
z=3
Substituting the obtained values on eq(3) to get the value of y,
−3x+2y+5z=−3
−6+2y+15=−3
2y=−12
y=−6
Values are,
x=2
y=−6
z=3
Page 209 Problem 11 Answer
It is given that the system of equations is
{ x+2y+3z=9
{ x+3y+2z=5
{ x+4y−z=−5.
It is required to solve the system with the matrix method.
Write the system of equations as a matrix. Perform row operations on the matrix till it gets converted into an identity matrix. Find the corresponding triples to the identity matrix.
The system \(\{\begin{array}{c}x+2 y+3 z=9 \\ x+3 y+2 z=5 \\ x+4 y-z=-5\end{array}\) can be written as a matrix as,
\(\left[\begin{array}{cccc}1 & 2 & 3 & 9 \\
1 & 3 & 2 & 5 \\
1 & 4 & -1 & -5
\end{array}\right]\)
Apply \(R 2 \rightarrow-R 1+R 2\) on the matrix.
\(\left[\begin{array}{cccc}1 & 2 & 3 & 9 \\
0 & 1 & -1 & -4 \\
0 & 2 & -4 & -14
\end{array}\right]\)
Apply \(R 3 \rightarrow-R 1+R 3\) on the matrix.
\(\left[\begin{array}{cccc}1 & 2 & 3 & 9 \\
0 & 1 & -1 & -4 \\
0 & 2 & -4 & -14
\end{array}\right]\)
Apply \(R 3 \rightarrow-2 R 2+R 3\) on the matrix.
\(\left[\begin{array}{cccc}1 & 2 & 3 & 9 \\
0 & 1 & -1 & -4 \\
0 & 0 & -2 & -6
\end{array}\right]\)
Apply \(R 3 \rightarrow 0.5 R 3\) on the matrix.
\(\left[\begin{array}{cccc}1 & 2 & 3 & 9 \\
0 & 1 & -1 & -4 \\
0 & 0 & -1 & -3
\end{array}\right]\)
Apply \(R 2 \rightarrow R 3+R 2\) on the matrix.
\(\left[\begin{array}{cccc}1 & 2 & 3 & 9 \\
0 & 1 & 0 & -1 \\
0 & 0 & 1 & 3
\end{array}\right]\)
Apply \(R 1 \rightarrow-3 R 3+R 1\) on the matrix.
\(\left[\begin{array}{cccc}1 & 2 & 0 & 0 \\
0 & 1 & 0 & -1 \\
0 & 0 & 1 & 3
\end{array}\right]\)
Apply \(R 1 \rightarrow-2 R 2+R 1\) on the matrix.
\(\left[\begin{array}{cccc}1 & 0 & 0 & 2 \\
0 & 1 & 0 & -1 \\
0 & 0 & 1 & 3
\end{array}\right]\)
The solution of the system
{ x+2y+3z=9
{ x+3y+2z=5
{ x+4y−z=−5 is the ordered triple (2,−1,3).
Page 212 Problem 12 Answer
It is given three equations,
x+2y+z=8 ……eq(1)
2x+y−z=4 ……eq(2)
x+y+3z=7 ……eq(3)
It is required to find the value of x,y and z for the given equations.
To solve this use gaussian elimination method then use row elimination method, after that simplify to get the solution.
It is given three equations,
x+2y+z=8 ……eq(1)
2x+y−z=4 ……eq(2)
x+y+3z=7 ……eq(3)
Then use gaussian elimination use to convert linear equation into matrix form
\(\left[\begin{array}{cccc}1 & 2 & \overline{1} & 8 \\
2 & 1 & -1 & 4 \\
1 & 1 & 3 & 7
\end{array}\right]\)
Now use certain operation, first \(R 2 \rightarrow R 2-2 R 1\)
\(\left[\begin{array}{cccc}1 & 2 & 1 & 8 \\
0 & -3 & -3 & -12 \\
1 & 1 & 3 & 7
\end{array}\right]\)
Then \(R 3 \rightarrow R 3-R 1\)
\(\left[\begin{array}{cccc}1 & 2 & 1 & 8 \\
0 & -3 & -3 & -12 \\
0 & -1 & 2 & -1
\end{array}\right]\)
Then \(R 3 \rightarrow R 3-\frac{1}{3} R 2\)
\(\left[\begin{array}{cccc}1 & 2 & 1 & 8 \\
0 & -3 & -3 & -12 \\
0 & 0 & -1 & 3
\end{array}\right]\)
Again \(R 1 \rightarrow R 1+\frac{2}{3} R 2\)
\(\left[\begin{array}{cccc}1 & 0 & -1 & 0 \\
0 & -3 & -3 & -12 \\
0 & 0 & -1 & 3
\end{array}\right]\)
Now, \(R 1 \rightarrow R +R 3\); \(R 2 \rightarrow R 2-3 R 3\)
Then, \(\left[\begin{array}{cccc}
1 & 0 & 0 & 3 \\
0 & -3 & 0 & -12 \\
0 & 0 & -1 & 3
\end{array}\right]\)
Then divide \(R 1 \rightarrow \frac{-1}{3} R 1\) and \(R 1 \rightarrow(-1) R 1\)
\(\left[\begin{array}{cccc}1 & 0 & 0 & 3 \\
0 & 1 & 0 & 4 \\
0 & 0 & 1 & -3
\end{array}\right]\)
Hence the solution will be,
x=3
y=4
z=-3
The solution of the system of equations will be,
x=3
y=4
z=−3
Page 213 Problem 13 Answer
It is given that the number of shirts is equal to the number of ties and four times the number of pairs of pants. The cost of shirts is 35, ties are 25, pants are 40 and the total cost is 560.
It is required to tell the number of shirts, pants, and ties.
Form a system of equations from the given data. Solve the equations to get the values of shirts, pants, and ties.
Let the number of shirts, ties, and pants be s,t, and p respectively.
The system of equations from the given data is,
s=t (1)
s=4p (2)
35s+25t+40p=560 (3)
Substitute s/ 4 for p and s for t in equation (3).
35s+25(s)+40(1/4s)=560
35s+25s+10s=560
70s=560
s=8
Find the value of t.
t=s
=8
Find the value of p.
p=s/4
=8/4
=2
The student bought 8 shirts, 8 ties and 2 pairs of pants.
Page 215 Problem 14 Answer
It is required to tell why a system has to have at least as many equations as unknown variables to have a unique solution.
A system must have at least as many numbers of equations as the number of variables.
For instance, if one tries to solve the system of three variables but only two equations are given, then the value of only two variables can be obtained and that too in the terms of the third variable.
This doesn’t solve the system and the system remains unsolved.
That’s why a minimum of three equations are required to solve a system of three variables.
Thus, a system needs to have at least as many equations as unknowns.
It is necessary for a system to have at least as many equations as variables as only then the equations can be solved to find the unknown values.
Page 215 Problem 15 Answer
It is required to find how a system of three linear equations can be solved.
Find the value of one variable from the first equation in the terms of other two variables.
Substitute the obtained value into the other two equations.
Now solve those obtained equation for these two variables.
Then put the values of these two variables in the value of third variable to get its value.
Put all three variable values in any of the equations to verify the answer.
A system of three linear equations can be solved by the method of substitution.
Page 216 Problem 16 Answer
It is given that the system is
{4x+y−2z=−6 (1)
{ 2x−3y+3z=9 (2)
{ x−2y=0 (3).
It is required to solve the system by substitution.
Find the value of x from x−2y=0. Substitute the value in other two equations. Solve for y and z.
Find x from its obtained value.
The value of x is 2y.
Substitute 2y for x in 4x+y−2z=−6 and 2x−3y+3z=9.
4(2y)+y−2z=−6
8y+y−2z=−6
9y−2z=−6
2(2y)−3y+3z=9
y+3z=9
Solve the equations.
9y−2z=−6
y+3z=9
Put z=9−y/3 in 9y−2z=−6.
9y−2(9−y/3)=−6
27y−18+2y=−18
29y=0
y=0
So, the value of x is also 0 as x=2y.
The value of z is,
y+3z=9
3z=9
z=3
The solution of the system
{4x+y−2z=−6 (1)
{ 2x−3y+3z=9 (2)
{ x−2y=0 (3) is, (0,0,3).
Page 216 Problem 17 Answer
Three linear equations in three variables are given.
4x+y−2z=−6 (1)
2x−3y+3z=9 (2)
x−2y=0 (3)
It is required to find the solution to the given system by elimination.
To find the solution to the given system by elimination, select any two equations and eliminate anyone of the variable by multiplying and addition certain factors.
Then, eliminate one more variable with the resultant equation and the third equation.
Thus, find the value of the remaining variable.
Then, by substitution find the values of other two variables.
Eliminate the variable z.
Multiply equation (1) by 3 and multiply equation (2) by 2.
12x+3y−6z=−18 (4)
4x−6y+6z=18 (5)
Add equation (1) and equation (2).
12x+3y−6z=−18
4x−6y+6z=18
16x−3y+0=0 (6)
Find the value of y.
Multiply equation (3) by 16 and subtract it from equation (6).
16x−32y=0
(−)16x−3y=0
0−29y=0
y=0
Therefore, x=0.
Use the value of x and y to find z.
Substitute zero for x,y in the equation 4x+y−2z=−6.
4(0)+(0)−2z=−6
z=3
The solution to the given system of equations is (0,0,3).
Page 217 Problem 18 Answer
Three linear equations in three variables are given.
x+5y+3z=4 (1)
4y−z=3 (2)
6x−2y+4z=0 (3)
It is required to find the solution to the given system by elimination.
To find the solution to the given system by elimination, select any two equations and eliminate anyone of the variable by multiplying and addition certain factors.
Then, eliminate one more variable with the resultant equation and the third equation.
Thus, find the value of the remaining variable. Then, by substitution find the values of other two variables.
Make an equation in terms of y and z.
Multiply equation (1) by −6 and add it to equation (3).
−6x−30y−18z=−24
6x−2y+4z=0
0−32y−14z=−24
16y+7z=12 (4)
Find the value of z.Multiply equation (2) by −4 and add it to equation (4).
−16y+4z=−12
16y+7z=12
11z=0
z=0
Find the value of y.
Substitute zero for z in the equation 4y−z=3.
4y−0=3
y=3/4
Find the value of x.
Substitute zero for z, 3/4 for y in the equation x+5y+3z=4.
x+5(3/4)+3(0)=4
x=4−15/4
x=1/4
The solution to the given system of equations is (1/4,3/4,0).
Page 28 Problem 19 Answer
Three linear equations in three variables are given.
x+5y+3z=4 (1)
4y−z=3 (2)
6x−2y+4z=0 (3)
It is required to find the solution to the given system by elimination.
To find the solution to the given system by matrices, write down the corresponding augmented matrix for the given system.
Then, try to get an identity matrix by performing certain row transformations.
The augmented matrix for the given system is \(\left[\begin{array}{ccc|c}1 & 5 & 3 & 4 \\ 0 & 4 & -1 & 3 \\ 6 & -2 & 4 & 0\end{array}\right]\).
Add \(-6 R_1\) to \(R_3\).
\(\left[\begin{array}{ccc|c}1 & 5 & 3 & 4 \\
0 & 4 & -1 & 3 \\
0 & -32 & -14 & -24
\end{array}\right]\)
Add \(8 R_2\) to \(R_3\) and then divide the row by -22.
\(\left[\begin{array}{ccc|c}1 & 5 & 3 & 4 \\
0 & 4 & -1 & 3 \\
0 & 0 & 1 & 0
\end{array}\right]\)
Add \(R_3\) to \(R_2\) and then divide the row by 4.
\(\left[\begin{array}{lll|l}1 & 5 & 3 & 4 \\
0 & 1 & 0 & \frac{3}{4} \\
0 & 0 & 1 & 0
\end{array}\right]\)
Add \(-5 R_2\) to \(R_1\).
\(\left[\begin{array}{lll|l}1 & 0 & 3 & \frac{1}{4} \\
0 & 1 & 0 & \frac{3}{4} \\
0 & 0 & 1 & 0
\end{array}\right]\)
Add \(-3 R_3\) to \(R_1\).
\(\left[\begin{array}{lll|l}1 & 0 & 0 & \frac{1}{4} \\
0 & 1 & 0 & \frac{3}{4} \\
0 & 0 & 1 & 0
\end{array}\right]\)
This means that,
\(\begin{aligned}& x=\frac{1}{4} \\
& y=\frac{3}{4} \\
& z=0
\end{aligned}\)
The solution to the given system of equations is (1/4,3/4,0).
Page 219 Problem 20 Answer
Three linear equations in three variables are given.
2x−y+3z=5 (1)
−6x+3y−9z=−15 (2)
4x−2y+6z=10 (3)
It is required to find the solution to the given system by elimination.
To find the solution to the given system by matrices, write down the corresponding augmented matrix for the given system.
Then, try to get an identity matrix by performing certain row transformations.
The augmented matrix for the given system is \(\left[\begin{array}{ccc|c}2 & -1 & 3 & 5 \\ -6 & 3 & -9 & -15 \\ 4 & -2 & 6 & 10\end{array}\right]\).
Divide \(R_2\) by 3 and \(R_3\) by 2 .
\(\left[\begin{array}{lll|l}2 & -1 & 3 & 5 \\
2 & -1 & 3 & 5 \\
2 & -1 & 3 & 5
\end{array}\right]\)
Since all given three equations are same, the solution to the given system cannot be determined.
Hence, the given system is inconsistent.
The given system of equation is inconsistent and there is no solution to the system.
Page 219 Problem 21 Answer
It is given that the vertical parabola equation is given by y=ax2+bx+c.
It is required to find the parabola that passes through the points (3,7),(30,−11) and (0,−1).
Substitute the coordinates of point into the general equation y=ax2+bx+c to get three equations.
Solve the equations for three variables x,y and z.
Put the obtained values back in the general equation to get the required parabola equation.
Substitute (3,7),(30,−11) and (0,−1) in the equation y=ax2+bx+c to obtain three equations.
7=a(3)2+b(3)+c
−11=a(30)2+b(30)+c
−1=a(0)2+b(0)+c
Simplify the equations.
7=9a+3b+c
−11=900a+30b+c
−1=c
Substitute −1 for c in the other two equations.
9a+3b−1=7
900a+30b−1=−11
On simplifying the equations,
9a+3b=8
90a+3b=−1
Solve the two equations by substituting 8−9a for 3b in 90a+3b=−1.
90a+8−9a=−1
81a=−9
a=−9
Therefore, b=8−9(−9)/3
b=89/3 Therefore, the equation of parabola is,
y=(−9)x2+(89/2)x−1−2y=19x2−89x+2
19x2−89x+2y+2=0
The equation of the vertical parabola passing through the points (3,7),(30,−11) and (0,−1) is 19x2−89x+2y+2=0.
Page 220 Problem 22 Answer
It is given that in triangle XYZ, the measure of angle X is equal to eight times the sum of the measures of other two angles and the measure of angle Y is equal to three times the measure of angle Z.
It is required to find the measures of all the angles.
The given problem involves, three linear equations in three variables.
Form three different equations by using the given data and the fact that the sum of all three angles of a triangle is 180
degrees. Then, solve the system by substitution method.
Let the measures of the three angles of the triangle be (x,y,z).
Here, x+y+z=180.
Substitute 3z for y,8(y+z) for x in the equation x+y+z=180. Simplify the equation and solve for z.
8(y+z)+3z+z=180
8(3z+z)+3z+z=180
32z+3z+z=180
z=180/36
z=5
Substitute five for z in the equation y=3z.
y=3(5)
y=15
Substitute 5 for z,15 for y in the equation x=8(y+z).
x=8(15+5)
x=8(20)
x=160
The measure of angle X is 160∘.
The measure of angle Y is 15∘.
The measure of angle Z is 5∘.
Page 220 Problem 23 Answer
It is given that the sum of the ages of James, Dan, Paul is 120 years.
James is three times the age of Dan and Paul is two times the sum of the ages of James and Dan.
It is required to find the ages of the three persons.
The given problem involves three linear equations in three variables. Form three different equations by using the given data.
Then, solve the system by substitution method.
Let the age of James be x.
Let the age of Dan be y.
Let the age of Paul be z.
Here, x+y+z=120.
x=3y
z=2(x+y)
Substitute 3y for x,2(x+y) for z in the equation x+y+z=120. Simplify the equation and solve for y.
3y+y+2(x+y)=120
4y+(3y+y)=120
8y=120
y=120/8
y=15
Substitute 15 for y in the equation x=3y.
x=3(15)
x=45
Substitute 15 for y,45 for x in the equation z=2(x+y).
z=2(45+15)
z=2(60)
z=120
The age of James is 45 years.
The age of Dan is 15 years.
The age of Paul is 120 years.
Page 220 Problem 24 Answer
It is given that the total number of shares are 10000 where stock A is four times stock B
and stock C is equal to the sum of A and B.
It is required to find the value of each stock.
Make three equations for the three variables A,B and C
from the given data. Find the value of any two variables in terms of the remaining one variable.
Substitute the values in any equation to get the value of that remaining variable. Now use this value to get the values of the other two variables.
Make three equations for the given data.
A+B+C=10000
A=4B
C=5B
Substitute 4B for A and 5B for C in the equation A+B+C=10000.
4B+B+5B=10000 Solve the equation for B.
10B=10000
B=1000
Find A and C by putting B=1000.
A=4(1000)=4000
C=5(1000)=5000
The shares of each stock A,B and C are 4000,1000 and 5000 respectively.
Page 221 Problem 25 Answer
It is required to find when a system has an infinite number of solutions and when it has zero solutions.
A system has an infinite number of solutions when its equations are parallel to each other or when their graphs are the same.
A system has no solutions when its equations do not intersect at a point.
The system has an infinite number of solutions when its equations are equal algebraically and graphically.
The system has zero solutions when its equations do not have an intersection point.
Page 221 Problem 26 Answer
It is given that the student tried to solve the system \(\left\{\begin{array}{l}5 x+7 y+9 x=0 \\ x-y+z=-3 \\ 8 x+y=12\end{array}\right.\) and wrote the determinant as \(\left[\begin{array}{lll}5 & 7 & 9 \\ 1 & -1 & 1 \\ 8 & 1 & 0\end{array}\right]\)
It is required to find and then correct the error done by the student.
The first equation of the system 5x+7y+9 x=0 can be further simplified as,
(5 x+9 x)+7 y=0
14 x+7 y=0
2 x+y=0
The student misread 9x as 9z and wrote the determinant as \(\left[\begin{array}{ccc}5 & 7 & 9 \\ 1 & -1 & 1 \\ 8 & 1 & 0\end{array}\right]\) which is incorrect.
However, the correct determinant should be \(\left[\begin{array}{ccc}2 & 1 & 0 \\ 1 & -1 & 1 \\ 8 & 1 & 0\end{array}\right]\) as the coefficient of z in the first equation is 0 .
So, the correct determinant is \(\left[\begin{array}{ccc}2 & 1 & 0 \\ 1 & -1 & 1 \\ 8 & 1 & 0\end{array}\right]\).
The student committed an error as he took \(9 \mathrm{x}\) as \(9 \mathrm{z}\) and the correct determinant is \(\left[\begin{array}{ccc}
2 & 1 & 0 \\
1 & -1 & 1 \\
8 & 1 & 0
\end{array}\right]\)
Page 221 Problem 27 Answer
It is given that the system of equations is
{7x+y+6z=1
{ −x−4y+8z=9.
It is required to find why the given system of equations cannot be solved.
The given system consists of three variables x,y and z .
To solve the values of three variables, at least three equations are required.
But the given system has only two equations.
Therefore, the given system cannot be solved.
The system of equations
{ 7x+y+6z=1
{ −x−4y+8z=9
cannot be solved as its equations have three variables but it has only two equations.
Page 222 Exercise 1 Answer
It is given that the part B is four times that of C, the part A is one-fifth of B+C and the number of parts is 6000.
The previous and new costs are 0.25,0.50,0.75 and 0.60,0.40,0.60 for parts A,B and C respectively.
It is required to find if the company would be able to buy the same quantity of parts at the price of 3000 or not.
Find the value of A,B and C from the given data. Find the cost for the values 0.60,0.40,0.60 by substituting for A,B and C. Check if it is equal to 3000 or not.
Make three equations for the given data.
0.25A+0.50B+0.75C=3000
B=4C
A=1/5(B+C)
Solve for the value of C.
Substitute 4C for B and 1/5(B+C) for A in the equation 0.25A+0.50B+0.75C=3000.
0.25(1/5(B+C))+0.50(4C)+0.75C=3000
0.25(1/5(4C+C))+0.50(4C)+0.75C=3000
3C=3000
C=1000
Find the value of B and A.
Substitute 1000 for C in B=4C to find B.
B=4(1000)
=4000
Substitute 4C for B and 1000 for C in A=1/5(B+C) to find B.
A=1/5(4C+C)
=1/5(5C)
=C
=1000
Find the new cost for the parts.
0.60A+0.40B+0.60C=0.60(1000)+0.40(4000)+0.60(1000)
=600+1600+600
=2800
The new price is $200 less than the previous cost of $3000.
The company will not be able to buy the same quantity of parts at the previous price as the new price is less than the previous price of $3000.
Page 223 Exercise 2 Answer
It is required to find how the systems of equations can be used to solve real-world problems.
Take the important data from the problem that may help in writing some equations.
Define variables for the unknown quantities.
Write the equations by using the defined variables.
Use substitution or graphing to solve the system of equations.
Verify the evaluated values of variables by putting the ordered pair in the original equations.
Now answer the questions according to the real-world problems.
A real world problem can be solved easily by taking some variables, making equations from the data and then solving them to get the answer.
Page 224 Exercise 3 Answer
It is given that the center of the circle is at (−7.5,15) and its radius is 1.5.
It is required to find the equation of a circle.
Substitute (−7.5,15) for (h,k) and 1.5 for r in the general equation (x−h)2+(y−k)2=r2 and simplify it to get the equation for the given circle.
Put (−7.5,15) for (h,k) and 1.5 for r in (x−h)2+(y−k)2=r2.
(x−(−7.5))2+(y−15)2=(1.5)2
(x+7.5)2+(y−15)2=(1.5)2
Simplify the expression.
(x+7.5)2+(y−15)2=(1.5)2
x2+56.25+15x+y2+225−30y=2.25
x2+y2+15x−30y+279=0
The equation of the circle with center at (−7.5,15) and radius 1.5 is x2+y2+15x−30y+279=0.
Page 224 Exercise 4 Answer
It is given that the equation of the circle is (x−5)2+(y−8)2=144.
It is required to find the center and the radius of the circle.
Compare the given equation of circle with the general equation to get the coordinates of the center and length of its radius.
The centre of the circle is, (5,8).
The radius of the circle is given by,
r2=144
r=√144
r=±12
Ignore the value −12 as the length of radius cannot be negative.
Hence, the length of the radius is 12.
The center and radius of the circle with the equation (x−5)2+(y−8)2=144 are (5,8) and 12 respectively.
Page 224 Exercise 5 Answer
It is given that the equation of the circle is x2+(y+6)2=50.
It is required to find the center and the radius of the circle.
Compare the given equation of circle with the general equation to get the coordinates of the center and length of its radius.
The centre of the circle is, (0,−6).
The radius of the circle is given by,
r2=50
r=√50
r=±5√2
Ignore the value −5√2 as the length of radius cannot be negative.
Hence, the length of the radius is 5√2.
The center and radius of the circle with the equation x2+(y+6)2=50 are (0,−6) and 5√2 respectively.
Page 224 Exercise 6 Answer
It is given that the system of equations is,
{ x−3y=2
{ y=x2+2x−34.
It is required to find the solutions of the given system.
Plot the given system of equations. Check if the given curve and the line meet at any point or not.
Find the coordinates of such points. These intersection points are the solutions of the system.
Draw the graph of the system
{ 4x+3y=1
{ y=x2
{ −x−1 on an online geometry calculator.
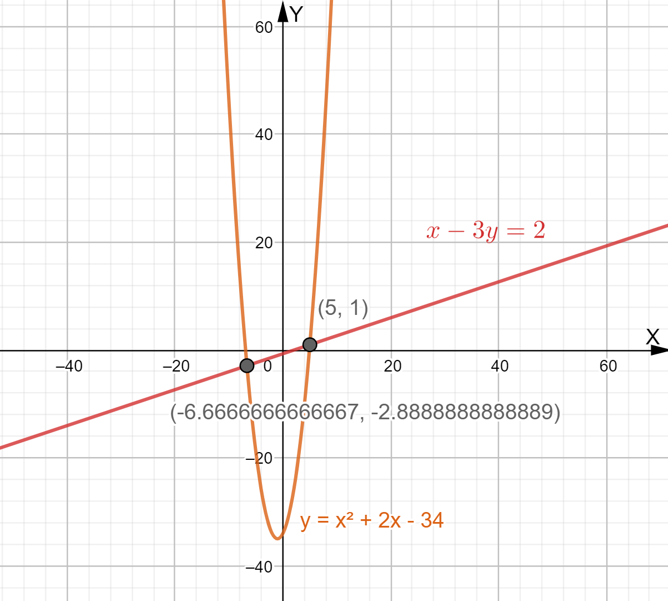
The curve y=x2−x−1 and the line 4x+3y=1 meet at two points on the graph.
The intersection points of the system are (−6.7,−2.9) and (5,1).
The solution of the given system of equations
{ 4x+3y=1
{ y=x2−x−1 are (−6.7,−2.9) and (5,1).
Page 224 Exercise 7 Answer
It is given that the system of equations is,
{ 3x+5y−2z=−7
{ −2x+7y+6z=−3
{ 8x+3y−10z=−11.
It is required to find the solutions of the given system.
Find the value of z from any one of the equations. Substitute its value in the other two equations to get them in terms of x and y.
Solve for the two variables and find the value of z from these.
The value of z from the first equation is given by,
3x+5y−2z=−7
2z=3x+5y+7
z=3x+5y+7/2
Put this value of z in the equation −2x+7y+6z=−3.
−2x+7y+6(3x+5y+7/2)=−3
−2x+7y+9x+15y+21=−3
7x+22y=−24
Put this value of z in the equation 8x+3y−10z=−11.
8x+3y−10(3x+5y+7/2)=−11
8x+3y−15x−25y−35=−11
7x+22y=−24
As both the equations obtained are equal, the number of solutions is infinite.
There are infinite solutions of the given system of equations
{ 3x+5y−2z=−7
{ −2x+7y+6z=−3
{ 8x+3y−10z=−11.
Page 225 Exercise 8 Answer
It is given a circle with center C(0,−2) and radius r=1.
It is required to find the equation of a given circle.
To solve this, substitute the point of centre in place of (h,k) and value of radius in place of r in the standard equation of circle.
Given circle have center C(0,−2) and radius r=1.
Substitute the value of center C(0,−2) and radius r=1 on equation,
(x−h)2+(y−k)2=r2
(x−0)2+(y−(−2))2=12
x2+(y+2)2=1
So, the equation of circle is,x2+(y+2)2=1
The equation of the circle is,x2+(y+2)2=1
Page 225 Exercise 9 Answer
It is given equation of a circle,x2+y2=25
It is required to find the center and radius of the given circle.
To solve this, compare the given equation to the standard equation to get center and radius.
Given equation of circle is,x2+y2=25
Now, standard equation of circle having centre C(h,k) and radius r is (x−h)2+(y−k)2=r2.
Then by comparing, center is C(0,0) and radius is r=5.
For the given circle center is C(0,0) and radius is r=5.
Page 225 Exercise 10 Answer
It is given equation of a circle,(x−18)2+(y+18)2=70
It is required to find the center and radius of the given circle.
To solve this, compare the given equation to the standard equation to get center and radius.
Given equation of circle is,(x−18)2+(y+18)2=70
Now, standard equation of circle having centre C(h,k) and radius r is (x−h)2+(y−k)2=r2.
Then by comparing, center is C(18,−18) and radius is r=√70.
For the given circle center is C(18,−18) and radius is r=√70.
Page 225 Exercise 11 Answer
It is given two equations
y+12=4x
y−20=x2−8x
It is required to find the value of x and y for given equations.
To solve this put the value of y from first equation in second equation.
Then solve the obtained equations by using basic calculations to get the value of x and y.
It is given two equations
y+12=4x…..eq(1)
y−20=x2−8x…..eq(2)
Substituting the value of y from eq(1) on eq(2),
y−20=x2−8x
4x−12−20=x2−8x
4x−32=x2−8x
So, the equation becomes x2−12x+32=0
Solving the equation,
x2−12x+32=0
x2−8x−4x+32=0
x(x−8)−4(x−8)=0
(x−4)(x−8)=0
So, values of x=4,8
For these values of x the values of y=4,20 respectively.
Solution of the given equations are (4,4) and (8,20).
Page 225 Exercise 12 Answer
It is given two equations
y=x+2
2y−12=2x2−8x
It is required to find the value of x and y for given equations.
To solve this put the value of y from first equation in second equation.
Then solve the obtained equations by using basic calculations to get the value of x and y.
It is given two equations
y=x+2…..eq(1)
2y−12=2x2−8x…..eq(2)
Substituting the value of y from eq(1) on eq(2),
2y−12=2x2−8x
2(x+2)−12=2x2−8x
2x+4−12=2x2−8x
2x−8=2x2−8x
So, the equation becomes
2x2−10x+8=0
x2−5x+4=0
Solving the equation,
x2−5x+4=0
x2−x−4x+4=0
x(x−1)−4(x−1)=0
(x−4)(x−1)=0
So, values of x=4,1
For these values of x the values of y=6,3 respectively.
Solution of the given equations are (4,6) and (1,3).
Page 225 Exercise 13 Answer
It is given three equations, −3x−12y−3z=0 ……eq(1)
x+4y+z=10 ……eq(2)
−2x−8y−2z=−34 ……eq(3)
It is required to find the value of x,y and z for the given equations.
To solve this use a gaussian elimination method then use row elimination method, after that simplify to get the solution.
It is given three equations, −3x−12y−3z=0 ……eq(1)
x+4y+z=10 ……eq(2)
−2x−8y−2z=−34 ……eq(3)
\(\left[\begin{array}{cccc}-3 & -12 & -3 & 0 \\
1 & 4 & 1 & 10 \\
-2 & -8 & -2 & -34
\end{array}\right]\)
Now use certain operations, first \(R 2 \rightarrow R 2+\frac{1}{3} R 1\); \(R 3 \rightarrow R 3-\frac{2}{3} R 1\)
\({\left[\begin{array}{cccc}-3 & -12 & -3 & 0 \\
0 & 0 & 0 & 10 \\
0 & 0 & 0 & -34
\end{array}\right]}\)
Here all the coefficient terms of R 2, R 3 is zero hence the system of equation has no solution.
Then use gaussian elimination use to convert linear equation into matrix form
The given system of equations has no solution.
Page 225 Exercise 14 Answer
It is required to discuss real life world situation that might involve three linear equation variables.
To solve this let consider a student shopping for clothes. He needs to buy four times of shirts of pants.
And he also needs to buy five times as many shirts as ties.
Here shirts costs $80, tie cost $50 and cost of pant is $70 how many shirts and pants and tiles did he got if he will spend only a sum of $1000.
In this problem there are three variables that are number of pants number of ties and number of shirts.
And equation will be,
4s=p
s=4t
80s+50t+70p=1000
This can be solved by the method of gaussian elimination method.
The real-life problem could be to solve this let consider a student shopping for clothes.
He needs to buy four times of shirts of pants. And he also needs to buy five times as many shirts as ties.
Here shirts costs $80, tie cost $50 and cost of pant is $700 how many shirts and pants and tiles did he got if he will spend only a sum of $1000.
Page 226 Exercise 15 Answer
It is given the focus and the directrix for three cases.
It is required to determine whether the parabola is horizontal or not in each case.
This can be done by using the equation of directrix, if the directrix is vertical then the parabola must be horizontal.
For part A, the focus is located at (−5,0) and the equation of its directrix is x=5.
So, in this case the directrix is vertical. Thus, the parabola in this case is horizontal.
For part B, the focus is located at (4,0) and the equation of its directrix is x=−4.
So, in this case the directrix is vertical. Thus, the parabola in this case is horizontal.
For part C, the focus is located at (0,−3) and the equation of its directrix is y=3.
So, in this case the directrix is horizontal. Thus, the parabola in this case is vertical.
The parabola is horizontal for part A and B but the parabola in part C is not horizontal.
Page 226 Exercise 16 Answer
It is given a system of equations y=x2+6x+10 and y+6=2x.
It is required to determine whether the following statements are correct or not.
This can be done by rewriting the equation then try to solve this system graphically by making a graph of the equations.
For part A: Yes, it is possible to rearranged the given system of equations to write the linear equation in terms of x making y the subject of the formula.
Thus, the equation y+6=2x can also be written asy=2x−6.
For part B: No, the given system of equations can be solved by both graphically as well as algebraically, by substituting the value of y from the linear equation into the quadratic equation.
For part C: No, the given system of equations has no solutions as shown by its graphical solution below.
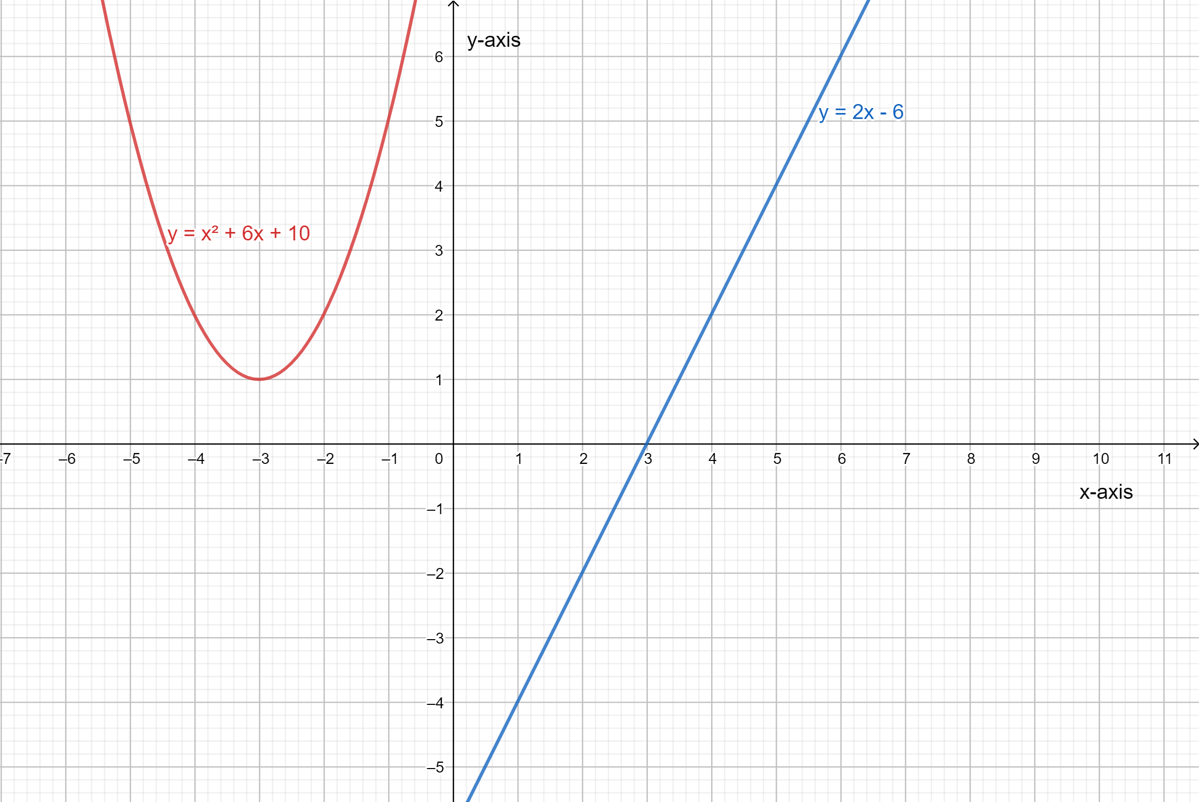
Statement in part A is correct while the statements in other two parts are wrong. This can be shown with the help of the diagram drawn below.
Page 226 Exercise 17 Answer
It is given a system of three equations.
It is required to explain how a system of three equations does in three variables can have infinitely many solutions.
An infinite number of solutions can result from several situations.
The three planes could be same, so that a solution will be the solution to the other two equations.
All three equations could be different, but they intersect on a line, which has infinite solutions.
A system of three equations in three variables have infinitely many solutions.
Page 226 Exercise 18 Answer
It is given that Robin solved a quadratic equation.
It is required to describe and correct her mistake.
This can be done by finding the mistake and then write the correct solution.
The mistake is in the third step where she has incorrectly written −4 on simplifying (−2)2.
This should be equal to .4 Therefore, the correct solution is as follows:
x=−(−2)±√(−2)2−4(1/4)(7)/2(1/4)
x=2±√4−7/1/2
x=4±2√−3
x=4±2i√3
The mistake is in the third step, (−2)2≠−4 and the correct solution is x=4±2i√3.
Page 227 Exercise 19 Answer
It is given a function f(x)=3(x−7)2+2.
It is required to determine whether the following statements are true or false.
This can be done by plotting the graph of the function then checking every part separately.
Draw the graph of the function.
Clearly, the axis of symmetry for f(x) is x=7 as shown in the diagram above.
As the minimum value of f(x) is 2 and maximum value is infinity then the range of f(x) is {2,∞}.
The vertex of the function f(x)=3(x−7)2+2 is (7,2) as shown in the diagram.
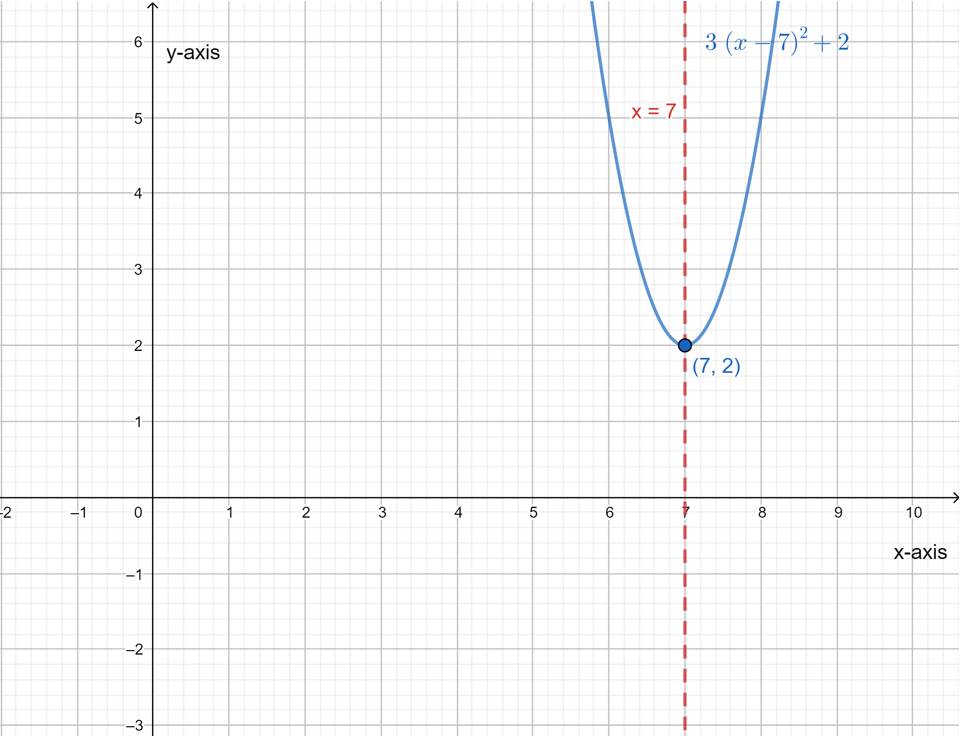
The statement in part A is true as the axis of symmetry is x=7 but the statements in part B
and C are false as the range of f(x) is {2,∞} and its vertex is (7,2).
Page 227 Exercise 20 Answer
It is given the following equations.It is required to determine whether the equations have real roots or not.
This can be done by checking the signs of the numbers on both side of the equality.
For part A: In equation x2−25=0.
This can also be written as x2=25. Thus, both sides have positive numbers therefore, this equation has real roots.
For part B: In equation −1/2x2−3=0.
This can also be written as x2=−6.
Thus, left hand side contains a negative number and the right-hand side contain a negative number which cannot be possible therefore, this equation does not have real roots.
For part C: In equation 3x2−4=2.
This can also be written as x2=2.
Thus, both sides have positive numbers therefore, this equation has real roots.
The equations x2−25=0 and 3x2−4=2
have real roots while the equation −1/2x2−3=0 does not have real roots.
Page 227 Exercise 21 Answer
It is given an equation 3x2−12x+15=0.
It is required to determine whether the following statements are true or false.
This can be done by solving this equation to calculate its roots and then solve it by completing the square method.
Divide the equation by 3.
x2−4x+5=0
Now this quadratic equation cannot be split so that it can make two factors.
So, the equation x2−4x+5=0 cannot be equal to (x−5)(x+1)=0.
Now find its discriminant.
D=(−4)2−4⋅1⋅5
D=16−20
D=−4
Thus,x=−b±√D/2a
x=4±2i/2
x=2±i
Now for solving the equation x2−4x+5=0
by completing the square method, add (−4/2)2
=4 on both side of the equality. Therefore,
x2−4x+4=−5+4
(x−2)2=−1
x=2±i
The statements in part A and B are false while the statement in part C is true.
Page 227 Exercise 22 Answer
It is given a system of equations y=2x2−3x+5 and y−3=x.
It is required to determine whether the following statements are correct or not.
This can be done by rewriting the equation then try to solve this system graphically by making a graph of the equations.
For part A: No, the given system of equations can be solved by both graphically as well as algebraically.
For part B: Yes, it is possible to rearrange the given system of equations to write the linear equation in terms of x making y the subject of the formula.
Thus, the equation y−3=x can also be written as y=x+3.
For part C: No, the given system of equations has only one solution as shown by its graphical solution below.
Statement in part B is correct while the statements in other two parts are wrong. This can be shown with the help of the diagram drawn below.
Page 228 Exercise 23 Answer
A quadratic equation is given as −4x2+x=3.
It is required to explain which method can be used to solve the equation and then solve it using that method.
The methods of factoring and completing squares cannot be used to solve the given equation as the discriminant is negative.
Therefore, only the quadratic formula can be used to solve the equation.
The given equation can be written as 4x2−x+3=0.
Substitute 4 for a, −1 for b, 3 for c in the formula x=−b±√b2−4ac/2a.
x=−(−1)±√(−1)2−4(4)(3)/2(4)
x=1±√1−48/8
x=1±i√47/8
To solve the given equation, only the quadratic formula can be used as the roots are complex.
The solution to the given equation is 1±i√47/8.
Page 228 Exercise 24 Answer
It is given that Ronald says that f(x)=0.5x+1.5 is an inverse of g(x)=−1.5x+4
It is required to check whether Ronald is correct with explanation.
Writing the inverse of g(x) means writing x as a function of g(x).
Then, replacing x by f(x) and g(x) by x.
Find the inverse and check whether it is the same as Ronald’s answer.
Write x as a function of g(x) from equation g(x)=−1.5x+4.
g(x)=−1.5x+4
1.5x=4−g(x)
x=4−g(x)/1.5
x=2.66−0.66g(x)
Replace x by f(x) and g(x) by x in equation x=2.66−0.66g(x).
f(x)=2.66−0.66x.Therefore, the statement of Ronald is incorrect.
The inverse of the given equation is f(x)=2.66−0.66x.
Page 228 Exercise 25 Answer
It is given that Keille wants to build a wire fence. A 80 feet roll provides an area of 100 feet squares and a 40 feet roll provides an area of 400 feet squares.
It is required to write a relation between the areas provided by the two rolls.
With the 80 ft. roll, Keille can build a fence of 100 feet squares.
With the 40ft. roll, Keille can build a fence of 400 feet squares.
This means that the area with 80ft. roll is four times larger than the area with 40 ft. roll.
If the area with 80 ft. roll is y and the area with 40ft. roll is x, then the relation between the two rolls can be written as y=4x.
Page 228 Exercise 26 Answer
It is given that Keille wants to build a wire fence. A 80 feet roll provides an area of 100 feet squares and a 40 feet roll provides an area of 400 feet squares.
It is required to show that the largest pen built from a 80 feet roll is two times the largest pen built from a 40 feet roll.
From the given figure, the largest pen built from 80 feet roll is of width 40 feet and the largest pen built from 40 feet of roll is 20 feet.
Therefore, the largest pen built from 80 feet roll is two times the largest pen built from 40 feet roll.
The largest pen built from a 80 feet roll is two times the largest pen built from a 40 feet roll.
Page 229 Exercise 27 Answer
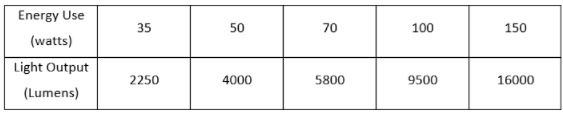
The table for the light produced by high-pressure sodium vapour streetlamps for different energy usage is given below.
It is required to find a quadratic model for the light output with respect to energy use.
To find a quadratic model for the light output with respect to energy usage, use Microsoft Excel and the plot the point by considering the energy usage values as x-coordinates and the light output values as y-coordinates. Select the trend-line to be quadratic and paste the equation.
Consider the energy usage values as x-coordinates and the light output values as y-coordinates.
Plot the given points in Microsoft Excel and choose the trend-line as polynomial of order two.
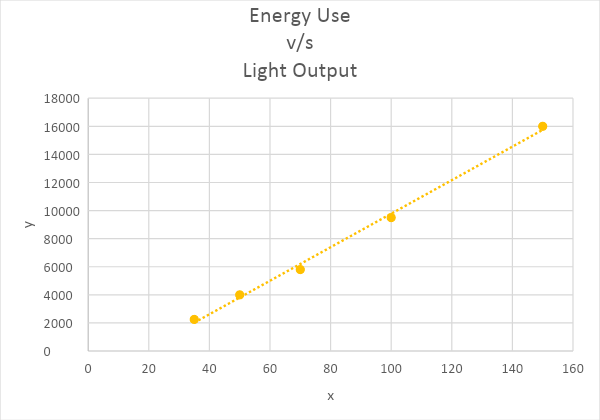
From the graph, the equation of the quadratic model of the given data is y=0.1875x2+84.339x−863.55.
The equation of the quadratic model of the given data is y=0.1875x2+84.339x−863.55.
Page 229 Exercise 28 Answer
The table for the light produced by high-pressure sodium vapour streetlamps for different energy usage is given below.
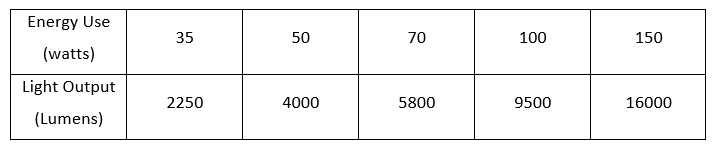
It is required to find a linear model for the light output with respect to energy use.
To find a linear model for the light output with respect to energy usage, use Microsoft Excel and the plot the point by considering the energy usage values as x-coordinates and the light output values as y-coordinates. Select the trend-line to be linear and paste the equation.
Consider the energy usage values as x-coordinates and the light output values as y-coordinates.
Plot the given points in Microsoft Excel and choose the linear trend-line.
From the graph, the equation of the linear model of the given data is y=119.38x−2159.4.
The equation of the linear model of the given data is y=119.38x−2159.4.
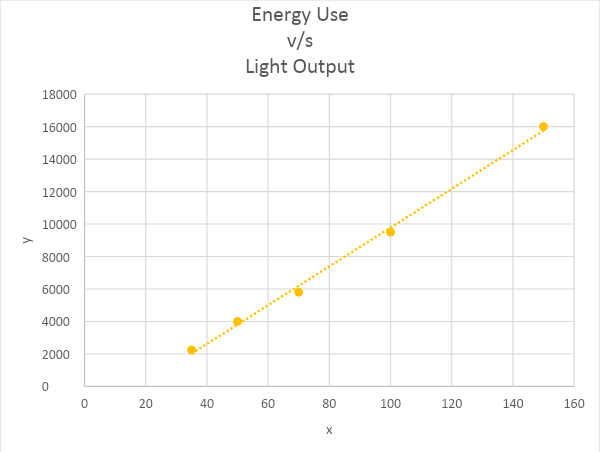
Page 229 Exercise 29 Answer
The table for the light produced by high-pressure sodium vapour street lamps for different energy usage is given below.
It is required to estimate the light output for a 200-watt bulb in both the model, linear as well as quadratic.
To estimate light output for a 200-watt bulb, from the quadratic model, substitute 200
for x in the equation y=0.1875x2+84.339x−863.55 and simplify.
To estimate light output for a 200-watt bulb, from the linear model, substitute 200
for x in the equation y=119.38x−2159.4 and simplify.
Substitute 200 for x in the equation y=0.1875x2+84.339x−863.55 and simplify.
y=0.1875(200)2+84.339(200)−863.55
y=23504.25
Therefore, the estimated value of the light output for a 200-watt bulb, from the quadratic model is 23504.25 lumens.
Substitute 200 for x in the equation y=119.38x−2159.4 and simplify.
y=119.38(200)−2159.4
y=21716.6
Therefore, the estimated value of the light output for a 200 watt bulb from the linear model, is 1716.6 lumens.
The estimated value of the light output for a 200-watt bulb, from the quadratic model is 23504.25 lumens.
The estimated value of the light output for a 200-watt bulb, from the linear model, is 21716.6 lumens.
Page 229 Exercise 30 Answer
The table for the light produced by high-pressure sodium vapor streetlamps for different energy usage is given below.
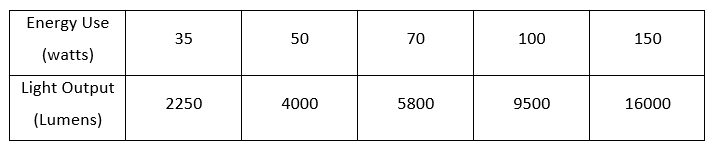
It is required to determine which model of the two- linear and quadratic gives a better estimate.
The quadratic model gives a better estimate as all the points on the graph almost lie on the curve.
The quadratic model gives a better estimate as all the points on the graph almost lie on the curve.