Financial Algebra 1st Edition Chapter 2 Modeling a Business
Page 99 Problem 1 Answer
Given: The revenue and expense functions are R=-350p2+18,000p and E=−1,500p+199,000.
To find The profit equation. For doing so, we will use the fact that the difference between the revenue and total cost functions is the profit function.
We know that the difference between the revenue and total cost functions is the profit function.
P=R−E
=(−350p2+18,000p)−(−1,500p+199,000)
=−350p2+19500p−199000
∴Our required profit equation isP=−350p2+19500p−199000.
Page 100 Problem 2 Answer
Given: Must maximum profit occur at the same price as the maximum revenue?
To find The explanation for the given.
We know that the profit equation is different from the revenue equation.
So, profit and revenue will have different parabolas with different points of maxima.
So, it is not necessary that maximum profit occurs at the same price as the maximum revenue.
No, it is not necessary that maximum profit occurs at the same price as the maximum revenue.
Page 101 Problem 3 Answer
Given: How might the quote apply to what has been outlined in this lesson?
To find: Summarize the lesson’s outline using a quote.
When you create a profit, your revenue exceeds your expenditures.
When you lose money, your costs outnumber your earnings.
Because your income exceeds your costs, your expenses are not more than your revenue, implying that you cannot lose money if you earn a profit.
∴ You cannot lose money if you earn a profit.
Page 101 Problem 4 Answer
Given:
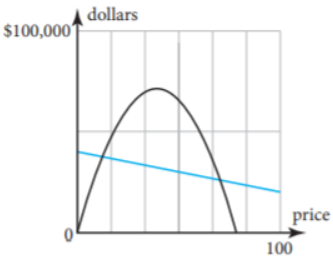
To find The range of prices when the profit is made.
For doing so, we will refer to the fact that when the income exceeds the costs, the black curve rises over the blue line, indicating a profit.
When the income exceeds the costs, the black curve rises over the blue line, indicating a profit.
We can see that the horizontal axis is split into six regions between0 and 100, therefore the width between successive tick marks on the horizontal axis is:100/6≈16.67.
The initial crossing of the black curve and the blue line is about two-thirds of the way between the vertical axis and the first tick mark, which corresponds to11.11.
2/3⋅100/6
=100/9
≈11.11
The second crossing of the black curve and blue line occurs about one-third of the way between the fourth and fifth tickmarks, corresponding to72.22.
(4+1/3)⋅100/6
=650/9
≈72.22
When the price is generally between$11.11 and $72.22, we profit.
Page 101 Problem 5 Answer
We have given a graph where the blue graph represents the expense function and the black graph represents the revenue function.
We have to describe the profit situation in terms of the expense and revenue functions.
We will use above information.
Here,We can see that between 0 and 100 on the horizontal axis is divided into 6 areas.
So, the width between the consecutive tick-marks on the horizontal axis is: 100/6
≈16.67
Now, The first intersection between the black curve and blue line is about one third between the vertical axis and the first tick-mark, which corresponds with roughly 11.11.
i.e.1/3⋅100/6
=50/9
≈5.56
The second intersection between the black curve and blue line is about two thirds between the fourth and fifth tick-mark, which corresponds with roughly 72.22
i.e.(4+2/3)⋅100/6
=700/9
≈77.78
Hence,
We make a profit when the price is roughly between $5.56 and $77.78.
Therefore, Price is roughly between $5.56 and $77.78
Page 101 Problem 6 Answer
Given :
E=−20,000p+90,000
R=−2,170p2+87,000p
We have to write the profit function for the given expense and revenue functions.
We will use above information.
Here, We have,
E=−20,000p+90,000
R=−2,170p2+87,000p
So,P=−2,170p2+87,000p−(−20,000p+90,000)
=−2,170p2+107,000p−90,000
Therefore,
P=−2,170p2+107,000p−90,000
Page 101 Problem 7 Answer
Given :
E=−6,500p+300,000
R=−720p2+19,000p
We have to write the profit function for the given expense and revenue functions.
We will use above information.
Here,We have,
E=−6,500p+300,000
R=−720p2+19,000p
So, P=−720p2+19,000p−(−6,500p+300,000)
=−720p2+25,500p−300,000
Therefore,
P=−720p2+25,500p−300,000
Page 101 Problem 8 Answer
Given :
E=−2,500p+80,000
R=−330p2+9,000p
We have to write the profit function for the given expense and revenue functions.
We will use above information.
Here, We have,
E=−2,500p+80,000
R=−330p2+9,000p
so,P=−330p2+9,000p−(−2,500p+80,000)
=−330p2+11,500p−80,000
Therefore,
P=−330p2+11,500p−80,000
Page 101 Problem 9 Answer
Given :
E=−12,500p+78,000
R=−1,450p2+55,000p
We have to write the profit function for the given expense and revenue functions.
We will use above information.
Here, We have,
E=−12,500p+78,000
R=−1,450p2+55,000p
So,P=−1,450p2+55,000p−(−12,500p+78,000)
=−1,450p2+67,500p−78,000
Therefore,
P=−1,450p2+67,500p−78,000
Page 102 Problem 10 Answer
Given: The revenue (black) and expense (blue) functions.
We have to examine the revenue (black) and expense (blue) functions.
And also estimate the price at the maximum profit and explain our reasoning.
We will use above information.
Here, As we know that, A profit is made when the revenue is larger than the expenses.
The maximum profit is then obtained when the difference between the revenue and expenses is largest.
The largest profit then appears to occur slightly to the right of the peak of the black curve.
Hence, The largest profit appears to occur at $25.00.
Therefore, The largest profit appears to occur at $25.00.
Page 102 Problem 11 Answer
Given : Profit functions of Exercises 6−9 models .
We have to determine which profit function models a no profit situation.
We will use above information.
Here, We have, The profit function is the difference between the revenue function and the expense function.
P=−2,170p2+107,000p−90,000
P=−720p2+25,500p−300,000
P=−330p2+11,500p−80,000
P=−1,450p2+67,500p−78,000
Now, the graph of all functions:
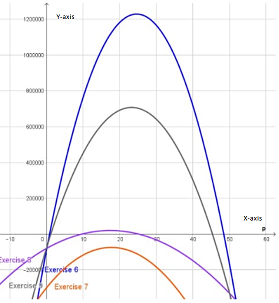
As the profit functions models a no profit situation if the profit is always negative (which indicates that we always make a loss and thus never make a profit).
We see that the profit function of exercise 7 models a no profit situation, as the graph lies completely below the horizontal axis, which indicates that the profit is always negative.
Therefore,
Problem 7 model is a no profit situation.
Page 102 Problem 12 Answer
Given: Profit functions of Exercises 6−9 models.
We have to show that how a profit function look like when no profit can be made.
We will use above information.
Here,The profit functions models a no profit situation if the profit is always negative (which indicates that we always make a loss and thus never make a profit).
As in part (a), we saw that the profit function of problem 7 models a no profit situation, as the graph lies completely below the horizontal axis, which indicates that the profit is always negative.
Thus a profit function lies completely below the horizontal axis when no profit can be made.
Therefore,
Page 102 Problem 13 Answer
Given : P=−400p2+12,400p−50,000
We have to determine the maximum profit and the price that would yield the maximum profit.
We will use above information.
Here,We have, P=−400p2+12,400p−50,000,
a=−400
b=12,400
c=−50,000
As we know that the maximum value occurs on the axis of symmetry.
The x-intercept of the axis of symmetry is determined −b/2a=−12,400/2×−400
⇒15.5
So, at p=15.5 the profit will be maximum.
Now, The maximum profit ,P=−400(15.5)2+12,400(15.5)−50,000,
P=−96,100+192,200−50,000
=46,100
Therefore, The maximum profit is 46,100
Page 102 Problem 14 Answer
Given:P=−370p2+8,800p−25,000
We have to determine the maximum profit and the price that would yield the maximum profit.
We will use above information.
Here,
We have, P=−370p2+8,800p−25,000
a=−370
b=8,800
c=−25,000
As we know that the maximum value occurs on the axis of symmetry.
The x-intercept of the axis of symmetry is determined −b/2a
=−8,800
2×−370
⇒11.89
So, at p=11.89 the profit will be maximum.
Now,The maximum profit ,P=−370(11.89)2+8,800(11.89)−25,000
P=−52,307.68+104,632−25,000
=27,324.32
Therefore,
The maximum profit is, P=27,324.32
Page 102 Problem 15 Answer
Given: P=−170p2+88,800p−55,000
To Determine the maximum profit and the price that would yield the maximum profit for each.
To determine the maximum profit algebraically, recall that the maximum value occurs on the axis of symmetry.
For the profit function given on left side The x – intercept of the axis of symmetry is determined −b/2a
P=−170p2+88,800p−55,000,a=−170,b=88,800, and c=−55,000.
Calculate −b/2a
=−88,800/2×−170
=261.18
Hence at p=261.18, the profit will be maximum now to find maximum profit substitute this value of p
P=−170(261.18)2+88,800(261.18)−55,000,
P=−11,596,548.71+23,192,784−55,000=11,541,235.29
At 261.18 the maximum proft would be 11,541,235.29.
Page 102 Problem 16 Answer
Given: The expense and revenue functions are
E=−1,850p+800,000 and R=−100p2+20,000p.
To Determine the profit function.
Using the formula P=R−E
we get
P=−100p2+20,000p−(−1,850p+800,000)
P=−100p2+21,850p−800,000
Therefore profit function is P=−100p2+21,850p−800,000
Page 102 Problem 17 Answer
Given: The expense and revenue functions are
E=−1,850p+800,000 and R=−100p2+20,000p.
To Determine the price, to the nearest cent, that yields the maximum profit.
To determine the maximum profit algebraically, recall that the maximum value occurs on the axis of symmetry. For the profit function given on left side The x – intercept of the axis
of symmetry is determined −b/2a
P=−100p2+21,850p−800,000
a=−100,b=21,850,c=−800,000
Calculate −b/2a
=−21,850/2×−100
=109.25
Therefore the price, to the nearest cent, that yields the maximum profit at $109.25
Page 102 Problem 18 Answer
Given: The expense and revenue functions are
E=−1,850p+800,000 and R=−100p2+20,000p
Also From previous exercise we know that p=$109.25
To Determine: the maximum profit, to the nearest cent.
Hence at p=109.25
The profit will be maximum now to find maximum profit substitute this value of p
P=−100(109.25)2+21,850(109.25)−800,000,
P=−1,193,556.25+2,387,112.5−800,000=393,556.25
The maximum profit, to the nearest cent is $393,556.25
Page 102 Problem 19 Answer
Given: The expense and revenue functions are E=−250p+50,000 and R=−225p2+7,200p
To Determine the profit function.
p=−225p2+7,200p-(250p+50,000)
p=−225p2+7.450p−50.000
Therefore the profit function is P=−225p{2}+7.450p−50.000
Page 102 Problem 20 Answer
Given: expense and revenue functions are E=−250p+50,000 and R=−225p2+7,200p
To Determine: the price, to the nearest cent, that yields the maximum profit.
To determine the maximum profit algebraically, recall that the maximum value occurs on the axis of symmetry.
For the profit function given on left side The x – intercept of the axis of symmetry is determined −b/2a
P=−225p2+7,450p−50,000
a=−225,b=7,450,c=−50,000
Calculate −b/2a
=−7,450/2×−225
=16.56
Therefore the price, to the nearest cent, that yields the maximum profit is at $16.56
Page 102 Problem 21 Answer
Given : The expense and revenue functions are E=−250p+50,000 and R=−225p2+7,200p
Also we know that p=$16.56
To Determine the maximum profit, to the nearest cent.
Hence at p=25.54 the profit will be maximum now to find maximum profit substitute this value of p
P=−225(16.56)2+7,450(16.56)−50,000
P=−61,702.56+123,372−50,000=11,669.44
Therefore the maximum profit, to the nearest cent is at $11,669.44
Chapter 2 Solving Linear Inequalities
-
- Cengage Financial Algebra 1st Edition Chapter 2 Assessment Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.1 Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.2 Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.3 Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.4 Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.5 Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.6 Modeling a Business
- Cengage Financial Algebra 1st Edition Chapter 2 Exercise 2.8 Modeling a Business