Algebra 1 Student Journal 1st Edition Chapter 2 Solving Linear Inequalities
Page 43 Essential Question Answer
Clear parenthesis on both sides of the inequality and collect-like terms.
Adding or subtracting terms so the variable is on one side and the constant is on the other side of the inequality sign.
And by multiplying and dividing by whatever constants are attached to the variable.
Also, remember to change the direction of the inequality if you multiply or divide by a negative number.
We can solve a multi-step inequality by solving the equation so that the variable are on one side and the constant are on the other side and then solving the equation and also to change the direction of the inequality if you multiply or divide by a negative number.
Read and Learn More Big Ideas Math Algebra 1 Student Journal 1st Edition Solutions
Page 43 Exploration 1 Answer
Given: The inequality is 2x+3≤x+5
To Find Solve the multi-step inequality.
Evaluate the question to get the answer
From the given we get
2x+3≤x+5
2x+3−3≤x+5−3
2x≤x+2
2x−x≤x+2−x
x≤2
After solving 2x+3≤x+5 we get x≤2.

Given: The inequality is −2x+3>x+9
To Find Solve the multi-step inequality.
Evaluate the question to get the answer.
From the given, we get that,
−2x+3>x+9
−2x+3−9>x+9−9
−2x−6>x
−2x+2x−6>x+2x
−6>3x
\(\frac{-6}{3}>\frac{3 x}{3}\)
−2>x
After solving −2x+3>x+9 we get x<−2.
Given: The inequality is 27≥5x+4x
To Find: Solve the multi-step inequality.
Evaluate the question to get the answer.
From the given we get
27≥5x+4x
27≥ 9x
\(\frac{27}{9} \geq \frac{9 x}{9}\)
3≥x
After solving 27≥5x+4x we get x≤3.
Given: The inequality is−8x+2x−16<−5x+7x
To Find Solve the multi-step inequality.
Evaluate the question to get the answer.
From the given we get
−8x+2x−16<−5x+7x
−6x−16<2x
−6x−16+6x<2x+6x
−16<8x
\(\frac{-16}{8}<\frac{8 x}{8}\)
−2<x
After solving−8x+2x−16<−5x+7x we get x>−2.
Given: The inequality is 3(x−3)−5x>−3x−6
To Find Solve the multi-step inequality.
Evaluate the question to get the answer.
From the given we get
3(x−3)−5x>−3x−6
3x−9−5x>−3x−6
−2x−9>−3x−6
−9>−x−6
−3>−x
3<x
After solving3(x−3)−5x>−3x−6 we get x>3.
Given The inequality is −5x−6x≤8−8x−x
To Find: Solve the multi-step inequality.
Evaluate the question to get the answer.
From the given we get
−5x−6x≤8−8x−x
−11x≤8−9x
−11x+9x≤8−9x+9x
−2x≤8
x≥−4
After solving−5x−6x≤8−8x−x we get x≥−4.
Big Ideas Math Algebra 1 Chapter 2 Exercise 2.4 Solution
Big Ideas Math Algebra 1 Student Journal 1st Edition Chapter 2 Solving Linear Inequalities Exercise 2.4 Page 44 Exercise 3 Answer
Given: The graph is
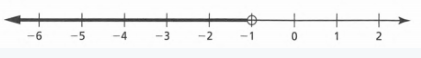
To Find Write two different multi-step inequalities whose solutions are represented by the graph.
Evaluate the question to get the answer.
From the given graph we get the inequality to be
x<−1
Now, adding 3x to both sides we get
x+3x<−1+3x
4x<−1+3x
Again adding 2 to both sides we get
4x+2<−1+3x+2
4x+2<3x+1
The two multi-step inequalities whose solutions are represented by the graph are, 4x<−1+3x
and 4x+2<3x+1.
Page 46 Exercise 1 Answer
Given: The value is 3x−2<10.
The graph is

To solve the inequality and Graph the solution.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for x:
3x−2<10 ( Given)
3x−2+2<10+2 (Add 2 to both sides)
3x<12 (Simplify )
\(\frac{3 x}{3}<\frac{12}{3}\) (Divide both sides by 3)
x<4
Plot x<4 as follows

The required solution is plot x<4 and graph is

Algebra 1 Student Journal Chapter 2 Exercise 2.4 Answers
Page 46 Exercise 2 Answer
Given: The value is 4a+8≥0.
The graph is

To find To solve the inequality, Graph the solution.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for a:
4a+8≥0 (Given)
4a+8−8≥0−8 ( Subtract 8 from both sides )
4a≥−8
\(\frac{4 a}{4} \geq \frac{-8}{4}\) ( Divide both sides by 4)
Plot a≥−2 as follows
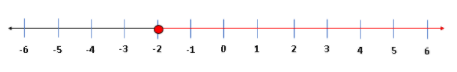
The required solution is plot x≥−2 and the graph is
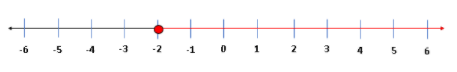
Big Ideas Math Algebra 1 Student Journal 1st Edition Chapter 2 Solving Linear Inequalities Exercise 2.4 Page 46 Exercise 3 Answer
Given: The value is \(2+\frac{b}{-3} \leq 3\).
The graph is
![]()
To solve the inequality, Graph the solution.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for b:
2+\(\frac{b}{-3} \leq 3\) ( Given)
2+\(\frac{b}{-3}-2 \leq 3\) (Subtract 2 to both sides )
\(\frac{b}{-3} \leq 1\)\(\frac{b}{-3} \cdot(-3) \leq 1 \cdot(-3)\) (Multiply both sides by −3)
b≤−3
b≥−3 ( Inequality symbol reverse)
b≥−3
Plot b≥−3 as follows

The required solution is Plot b≥−3 and the graph is
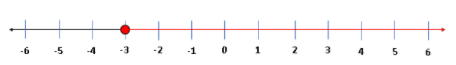
Page 46 Exercise 4 Answer
Given : The value is −\(-\frac{c}{2}-6>-8\).The graph is
![]()
To find To solve the inequality, Graph the solution.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for c:
\(-\frac{c}{2}-6>-8\) (Given)
\(-\frac{c}{2}\) −6+6>−8+6 (Add 6 to both sides)
\(-\frac{c}{2}>-2\) (Inequality symbol reverses)
c<−4 (Multiply both sides by −2)
c<−4
Plot c <−4 as follows
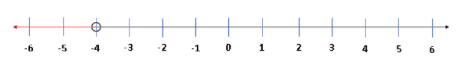
The required solution is c<−4 graph is
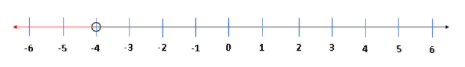
Page 46 Exercise 5 Answer
Given: The value is 8≤−4(d+1).
The graph is
![]()
To solve the inequality, Graph the solution.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for d :
8≤−4(d+1) (Given)
8≤−4d−4 ( Distribute )
8+4≤−4d−4+4 ( Add 4 to both sides)
12≤−4d
\(\frac{12}{-4} \leq \frac{-4 d}{-4}\) (Divide both sides by −4)
−3≤d (Inequality symbol reverses )
−3≥d d≤−3
Plot d≤−3 as follows

The required solution is plot d≤−3 and graph is

Chapter 2 Exercise 2.4 Step-By-Step Solutions Big Ideas Math
Big Ideas Math Algebra 1 Student Journal 1st Edition Chapter 2 Solving Linear Inequalities Exercise 2.4 Page 47 Exercise 6 Answer
Given: The value is 5−2n>8−4n.
To find: To solve the inequality.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for n :
5−2n>8−4n (Given)
5−2n−5>8−4n−5 (Subtract 5 to both sides )
−2n>3−4n
−2n+4n>3−4n+4n (Add 4n to both sides )
2n>3
\(\frac{2 n}{2}>\frac{3}{2}\)
\(n>\frac{3}{2}\) (Divide both sides by 2)
Plot \(n>\frac{3}{2}\) as follows
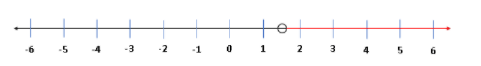
The required solution is\(n>\frac{3}{2}\) and graph is
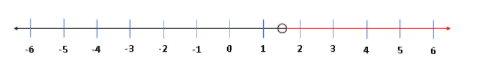
Page 47 Exercise 7 Answer
Given: The value is 6h−18<6h+1
To solve the inequality.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for h:
6h−18<6h+1 ( Given)
6h−18+18<6h+1+18 (Add 18 to both sides)
6h<6h+19
6h−6h<6h+19−6h (Subtract oh to both sides )
0<19
There is no solution to this inequality
The result is 0<19 there is no solution to this inequality.
Page 47 Exercise 8 Answer
Given: The value is 3p+4≥−4p+25
To solve the inequality.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for p:
3p+4≥−4p+25 (Given)
3p+4+4p≥−4p+25+4p (Add 4p to both sides)
7p+4≥25
7p+4−4≥25−4 ( Subtract 4 to both sides )
7p≥21
\(\frac{7 p}{7} \geq \frac{21}{7}\) (Divide both sides by 7)
p≥3
Hence the solution of the given inequality 3p+4≥−4p+25 is p≥3.
Big Ideas Math Chapter 2 Exercise 2.4 Walkthrough
Big Ideas Math Algebra 1 Student Journal 1st Edition Chapter 2 Solving Linear Inequalities Exercise 2.4 Page 47 Exercise 9 Answer
Given: The value is 7j−4j+6<−2+3j.
To solve the inequality.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for j :
7j−4j+6<−2+3j ( Given)
3j+6<−2+3j (Simplify)
3j+6−6<−2+3j−6 ( Subtract 6 to both sides)
3j<3j−8
3j−3j<3j−8−3j (Subtract 3j to both sides )
0<−8
There is no solution to this inequality
The required solution is 0<−8, there is no solution to the inequality.
Page 47 Exercise 10 Answer
Given : The value is 12\(12\left(\frac{1}{4} w+3\right) \leq 3(w-4)\).
To find: To solve the inequality.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for w:
12\(12\left(\frac{1}{4} w+3\right) \leq 3(w-4)\) (Given)
\(\frac{12}{4} w\)+12⋅3≤3w−3⋅4 (Distribute)
3w+36≤3w−12 (Simplify)
3w+36−36≤3w−12−36 (Subtract 36 to both sides)
3w≤3w−48
3w−3w≤3w−48−3w (Subtract 3w to both sides )
0≤−48
There is no solution to this inequality
The required solution is 0≤−48, there is no solution to the inequality.
Big Ideas Math Algebra 1 Student Journal 1st Edition Chapter 2 Solving Linear Inequalities Page 47 Exercise 11 Answer
Given : The value is k(4r−5)≥−12r−9
To find the value of k.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for k:
To have all real numbers as a solution for the inequality, we must find a value of k that is independent of r and the other constants.
We solve for as
k(4r−5)≥−12r−9
Given remove constants and inequality symbol
k4r=−12r
\(\frac{k 4 r}{4 r}=\frac{-12 r}{4 r}\) ( Divide both sides by 4r)
Given: k(4r−5)≥−12r−9 ,k=−3
k(4r−5)≥−12r−9 (Given )
−3(4r−5)≥−12r−9 (k=−3 distribute)
−3⋅4r−3⋅(−5)≥−12r−9
Test 1:
−12r+15≥−12r−9 (Set r=0)
−12(0)+15≥−12(0)−9 (Simplify)
15≥−9 (This is true )
Test 2:
−12r+15≥−12r−9 (Set r=1)
−12(1)+15≥−12(1)−9 (Simplify)
3≥−21 (This is also true)
Hence, if k=−3, the solution of r is the set of all real numbers
The required solution is k=−3 the solution of r is the set of all real numbers.
Page 47 Exercise 12 Answer
Given: The value is 2kx−3k<2x+4+3kx.
To find: To find the value of k.
Evaluate to get the solution.
Let us consider the given value and simplify
Solve for k:
Given, 3kx−3k<2x+4+3kx,
find the value of k such that this inequality has no solution. We only consider the values that are dependent on x, such that
3kx−3k<2x+4+3kx (Given)
2kx=2x+3kx ( Constants and inequality symbol are removed)
\(\frac{2 k}{x}\)=\(\frac{2 x}{x}+\frac{3 k x}{x}\) (Divide all terms by x)
2k=2+3k
2k−3k=2+3k−3k
−k=2
−k(−1)=2(−1)
k=−3 ( Subtract 3k to both sides)
Checking:
3kx−3k<2x+4+3kx ( Given )
3(−2)x−3(−2)<2x+4+3(−2)x ( Set k=−2)
Test:1
3(−2)x−3(−2)<2x+4+3(−2)x (Set x=0)
3(−2)(0)−3(−2)<2(0)+4+3(−2)(0) ( Simplify )
0+6<0+4+0
6<4 ( This is not true )
Test 2:
3(−2)x−3(−2)<2x+4+3(−2)x (Set x=1)
3(−2)(1)−3(−2)<2(1)+4+3(−2)(1) ( Simplify )
−6+6<2+4+−6
0<0 ( This is not true )
Hence, if k=−2, any value of r is not a solution to this inequality
The required solution is k=−2 which makes the inequality 2kx−3k<2x+4+3kx to have no solution.
Algebra 1 Exercise 2.4 Explanation Big Ideas Math
