Algebra 2 Volume 1 1st Edition Module 3 Quadratic Equations
Page 127 Problem 1 Answer
A complex number is a number that is of the form a+bi, where a and b are real numbers, and i is the imaginary unit equal to √−1.
Consider the two arbitrary complex numbers a+bi and c+di.The sum of the two complex numbers can be written as (a+bi)+(c+di)
. In the sum, the terms a and c, and the terms bi and di are like terms.By grouping the like terms, the sum can be written as (a+bi)+(c+di)=(a+c)+(bi+di).
The right-hand side of the sum can be simplified by combining the like terms in the parentheses to find the simplified sum of the two complex numbers.
The difference of the two complex numbers can be written as (a+bi)−(c+di). In the difference, the terms a and c, and the terms bi and di are like terms.
By grouping the like terms, the difference can be written as (a+bi)−(c+di)=(a−c)+(bi−di).
The right-hand side of the difference can be simplified by combining the like terms in the parentheses to find the simplified difference of the two complex numbers.
Thus, the sum and difference of two complex numbers can be determined by grouping the like terms, that is the real parts and the imaginary parts, and then combining the like terms.
When a+bi and c+di are multiplied using the distributive property of multiplication, the product is (a+bi)(c+di)=ac+adi+bci+bdi2.Using the value of i2 and simplifying the expression, the resulting expression is (a+bi)(c+di)=ac+(ad+bc)i−bd.
The right-hand side of the equation can be grouped as (ac−bd)+(ad+bc)i.
Thus, the product of two complex numbers can be determined by using the distributive property of multiplication, combining the like terms, and then using the value of i2 to simplify the product.
A number is called a complex number if it can be written in the form a+bi, where a and b are real numbers, and i is the imaginary unit equal to √−1.
Two complex numbers can be added or subtracted by writing the expression for the sum or difference, grouping the like terms, that is the real parts and the imaginary parts, and then combining the like terms.
Two complex numbers can be multiplied by using the distributive property of multiplication, combining the like terms, and then using the value of i2 to simplify the product.
Page 127 Problem 2 Answer
The given binomials are 3+4x and 2−x.
The question requires to add the binomials 3+4x and 2−x.
To add the binomials, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Write the sum of 3+4x and 2−x, group the like terms in parentheses, and combine the like terms in the parentheses.
(3+4x)+(2−x)=(3+2)+(4x+(−x))
=(3+2)+(4x−x)
=5+3x
Thus, the required sum is 5+3x.
The required sum is 5+3x.
Page 127 Problem 3 Answer
The given binomials are 3+4x and 2−x.
The question requires to subtract 2−x from 3+4x.
To subtract the binomials, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Write the difference of 2−x from 3+4x, and rewrite the subtraction as addition.
Then, group the like terms in parentheses, and combine the like terms in the parentheses.
(3+4x)−(2−x)=(3+4x)+(−2+x)
=(3+(−2))+(4x+x)
=(3−2)+(4x+x)
=1+5x
Thus, the required difference is 1+5x.
The required difference is 1+5x.
Page 127 Problem 4 Answer
The given binomials are 3+4x and 2−x.
The question requires to multiply the binomials 3+4x and 2−x.
To multiply the binomials, use the FOIL method, and then combine the like terms.
Write the product of 3+4x and 2−x, use the FOIL method, and combine the like terms.
(3+4x)(2−x)=6+(−3x)+8x+(−4x2)
=6−3x+8x−4x2
=6+5x−4x2
Thus, the required product is 6+5x−4x2.
The required product is 6+5x−4x2.
Page 127 Problem 5 Answer
The given equation is (3+4x)+(2−x)=5+3x.
The question requires to write the equation obtained if x is equal to the imaginary unit i.
To obtain the required equation, replace the variable x by the imaginary unit i on both sides of the given equation.
Substitute i for x on both sides of (3+4x)+(2−x)=5+3x.
(3+4i)+(2−i)=5+3i
Thus, the required equation is (3+4i)+(2−i)=5+3i.
The required equation is (3+4i)+(2−i)=5+3i.
Page 127 Problem 6 Answer
The given equation is (3+4x)(2−x)=6+5x−4x2.
The question requires to write the equation obtained if x is equal to the imaginary unit i.
Also, it is required to explain how the right side of the obtained equation can be simplified further.
To obtain the required equation, replace the variable x by the imaginary unit i on both sides of the given equation.
Then, use the value of i2 to simplify the right side of the equation further.
Substitute i for x on both sides of (3+4x)(2−x)=6+5x−4x2.
(3+4i)(2−i)=6+5i−4i2
Thus, the required equation is (3+4i)(2−i)=6+5i−4i2.
The right side of the equation contains the square i2, which is equal to −1.
Substitute −1 for i2 on the right side of (3+4i)(2−i)=6+5i−4i2, and simplify the equation.
(3+4i)(2−i)=6+5i−4(−1)
(3+4i)(2−i)=6+5i+4
(3+4i)(2−i)=10+5i
Thus, the right side of the equation can be simplified as 10+5i.
The required equation is (3+4i)(2−i)=6+5i−4i2.
The right side of the obtained equation is 6+5i−4i2, which contains the square i2.
The right side can be further simplified by substituting −1 for i2, and then combining the like terms to simplify the right side as 10+5i.
Page 128 Problem 7 Answer
The given number is −7i, which can be written as the complex number 0−7i.
Therefore, the real part of −7i is 0 and the imaginary part of −7i is −7.
Since the given number can be written as the complex number 0−7i having the real part 0, it belongs to the set of imaginary numbers and the set of complex numbers.
The real part and imaginary part of −7i are 0 and −7 respectively.
The given number −7i belongs to the set of imaginary numbers and the set of complex numbers.
Page 129 Problem 8 Answer
The given number is −1+i, which can be written as the complex number −1+1⋅i.
Therefore, the real part of −1+i is −1 and the imaginary part of −1+i is 1.
Since the given number can be written as the complex number −1+1⋅i having nonzero real and imaginary parts, it belongs to only the set of complex numbers.
The real part and imaginary part of −1+i are −1 and 1 respectively.
The given number −1+i belongs to only the set of complex numbers.
Page 129 Problem 9 Answer
The given sum is (a+bi)+(a−bi). The numbers a and b are real.
The question requires to determine and explain whether the sum (a+bi)+(a−bi) is a real number or an imaginary number.
To answer the question, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Then, observe the real and imaginary parts of the sum, and determine whether the number is real or imaginary.
Group the real and imaginary parts in the sum (a+bi)+(a−bi), and then combine the like terms.
(a+bi)+(a−bi)=(a+a)+(bi−bi)
=2a+0
=2a
Thus, the required sum is 2a.
The sum 2a can be written as the complex number 2a+0i.
Therefore, the real and imaginary part of 2a are 2a and 0 respectively.
Since the imaginary part of the sum 2a is 0, the sum 2a is a real number.
The sum (a+bi)+(a−bi) is a real number because the sum is equal to 2a, and the imaginary part of 2a is equal to 0.
Page 129 Problem 10 Answer
The given difference is (17−6i)−(9+10i).
The question requires to subtract the complex numbers in the given difference.
To subtract the complex numbers, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Rewrite the subtraction of the complex numbers in (17−6i)−(9+10i) as addition.
Then, group the real and imaginary parts, and combine the like terms.
(17−6i)−(9+10i)=(17−6i)+(−9−10i)
=(17−9)+(−6i−10i)
=8−16i
Thus, the required difference is 8−16i.
The required difference is 8−16i.
Page 129 Problem 11 Answer
The given difference is (18+27i)−(2+3i).
The question requires to subtract the complex numbers in the given difference.
To subtract the complex numbers, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Rewrite the subtraction of the complex numbers in (18+27i)−(2+3i) as addition. Then, group the real and imaginary parts, and combine the like terms.
(18+27i)−(2+3i)=(18+27i)+(−2−3i)
=(18−2)+(27i−3i)
=16+24i
Thus, the required difference is 16+24i.
The required difference is 16+24i.
Page 130 Problem 12 Answer
The given product is (6−5i)(3−10i).
The question requires to multiply the complex numbers in the given product.
To multiply the complex numbers, use the distributive property of multiplication and the value of i2, and simplify the expression.
Apply the distributive property of multiplication in the given product (6−5i)(3−10i), and then combine the like terms.
(6−5i)(3−10i)=18−60i−15i+50i2
=18−75i+50i2
Substitute −1 for i2, and then simplify the expression.
(6−5i)(3−10i)=18−75i+50(−1)
=18−75i−50
=−32−75i
Thus, the required product is −32−75i.
The required product is −32−75i.
Page 130 Problem 13 Answer
The given product is (8+15i)(11+i).
The question requires to multiply the complex numbers in the given product.
To multiply the complex numbers, use the distributive property of multiplication and the value of i2, and simplify the expression.
Apply the distributive property of multiplication in the given product (8+15i)(11+i), and then combine the like terms.
(8+15i)(11+i)=88+8i+165i+15i2
=88+173i+15i2
Substitute −1 for i2, and then simplify the expression.
(8+15i)(11+i)=88+173i+15(−1)
=88+173i−15
=73+173i
Thus, the required product is 73+173i.
The required product is 73+173i.
Page 130 Problem 14 Answer
The given product is (−3+12i)(7+4i).
The question requires to multiply the complex numbers in the given product.
To multiply the complex numbers, use the distributive property of multiplication and the value of i2, and simplify the expression.
Apply the distributive property of multiplication in the given product (−3+12i)(7+4i), and then combine the like terms.
(−3+12i)(7+4i)=−21−12i+84i+48i2
=−21+72i+48i2
Substitute −1 for i2, and then simplify the expression.
(−3+12i)(7+4i)=−21+72i+48(−1)
=−21+72i−48
=−69+72i
Thus, the required product is −69+72i.he required product is −69+72i.
Page 131 Problem 15 Answer
The given diagram shows the components in a circuit. The given current I is 24+12i amps.
The question requires to determine the voltage of each component of the circuit.
To determine the voltage of each component, use the diagram to write the impedance of each component.
Then, use the impedance and current in the Ohm’s law to determine the required voltage of each component.
From the circuit diagram, it can be observed that the resistor is labelled 4 ohms.
Therefore, the impedance of the resistor can be represented by the complex number 4.
The inductor is labelled 3 ohms. Therefore, the impedance of the inductor can be represented by the complex number 3i.
The capacitor is labelled 5 ohms. Therefore, the impedance of the capacitor can be represented by the complex number −5i.
Substitute 24+12i for I and 4 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(24+12i)(4)
=96+48i
Thus, the voltage of the resistor is 96+48i volts.
Substitute 24+12i for I and 3i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(24+12i)(3i)
=72i+36i2
=72i+36(−1)
=−36+72i
Thus, the voltage of the inductor is −36+72i volts.
Substitute 24+12i for I and −5i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute 1 for i2.
V=(24+12i)(−5i)
=−120i−60i2
=−120i−60(−1)
=60−120i
Thus, the voltage of the capacitor is 60−120i volts.
The voltage of the resistor, inductor, and capacitor are 96+48i volts, −36+72i volts, and 60−120i volts respectively.
Page 132 Problem 16 Answer
The given diagram shows the components in a circuit. The given current I is 24+12i amps.
The question requires to determine the sum of the voltages for the three components, and write what is noticed by the result.
Given that the voltage of the resistor, inductor, and capacitor are 96+48i volts, −36+72i volts, and 60−120i volts respectively, as determined in part B of example 4.
To determine the sum of voltages, add the voltages by grouping the like terms and combining them.
Then, compare the sum to the voltage across the power source and write what is noticed.
Write the sum of the voltages of the three components, group the real and imaginary parts in the sum, and then combine the like terms.
(96+48i)+(−36+72i)+(60−120i)=(96−36+60)+(48i+72i−120i)
=120+0i
=120
Thus, the sum of the voltages for the three components is 120 volts.
From the diagram, it can be observed that the power source is labelled 120 V.
This means that the voltage across the power source is 120 volts.
Thus, the sum of the voltages for the three components is equal to the voltage across the power source, that is 120 volts.
The sum of the voltages for the three components is 120 volts.
The sum of the voltages for the three components is equal to the voltage across the power source, that is 120 volts.
Page 132 Problem 17 Answer
It is given that a second resistor with impedance equal to 2 ohms is added to the circuit diagram given in example 4. The given current I is 18+6i amps.
The question requires to determine the total impedance, and then determine the voltage of each component of the circuit.
To determine the total impedance and voltage of each component, use the diagram to write the impedance of each component as a complex number.
Then, add the complex numbers to determine the total impedance.
Next, use the impedance and current in the Ohm’s law to determine the required voltage of each component.
From the circuit diagram, it can be observed that the first resistor has the impedance 4 ohms.
Therefore, the impedance of the first resistor can be represented by the complex number 4.
The inductor has the impedance 3 ohms. Therefore, the impedance of the inductor can be represented by the complex number 3i.
The capacitor has the impedance 5 ohms. Therefore, the impedance of the capacitor can be represented by the complex number −5i.
The second resistor has the impedance 2 ohms. Therefore, the impedance of the second resistor can be represented by the complex number 2.
The total impedance of the circuit is equal to the sum of the impedances of the four components.
Write the sum of the impedances of the four components, group the real and imaginary parts in the sum, and then combine the like terms.
4+3i+(−5i)+2=(4+2)+(3i−5i)
=6−2i
Thus, the total impedance is 6−2i ohms.
Substitute 18+6i for I and 4 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(18+6i)(4)
=72+24i
Thus, the voltage of the first resistor is 72+24i volts.
Substitute 18+6i for I and 2 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(18+6i)(2)
=36+12i
Thus, the voltage of the second resistor is 36+12i volts.
Substitute 18+6i for I and 3i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(18+6i)(3i)
=54i+18i2
=54i+18(−1)
=−18+54i
Thus, the voltage of the inductor is −18+54i volts.
Substitute 18+6i for I and −5i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(18+6i)(−5i)
=−90i−30i2
=−90i−30(−1)
=30−90i
Thus, the voltage of the capacitor is 30−90i volts.
The required total impedance is 6−2i ohms.
The voltages of the first resistor, the second resistor, the inductor, and the capacitor are 72+24i volts, 36+12i volts, −18+54i volts, and 30−90i volts respectively.
Page 132 Problem 18 Answer
Take the two arbitrary complex numbers a+bi and c+di.When a+bi and c+di are added, the sum is (a+bi)+(c+di)=(a+c)+(b+d)i, which is a complex number.
The sum (a+c)+(b+d)i is a real number only if the imaginary part (b+d) is equal to 0.
The imaginary part (b+d) is the sum of the imaginary parts of the complex numbers a+bi and c+di.
Therefore, the sum of two complex numbers is a real number only when the sum of their imaginary parts is equal to 0.
The sum (a+c)+(b+d)i is an imaginary number only if the real part (a+c) is equal to 0.
The real part (a+c) is the sum of the real parts of the complex numbers a+bi and c+di.
Therefore, the sum of two complex numbers is an imaginary number only when the sum of their real parts is equal to 0.
The sum of two complex numbers is a real number only when the sum of the imaginary parts of the two complex numbers is equal to 0.
The sum of two complex numbers is an imaginary number only when the sum of the real parts of the two complex numbers is equal to 0.
Page 133 Problem 19 Answer
Consider the two arbitrary binomial linear expressions a+bx and c+dx.
When a+bx and c+dx are multiplied using the distributive property of multiplication, the product is(a+bx)(c+dx)=ac+adx+bcx+bdx2.
Grouping the like terms, the resulting product is (a+bx)(c+dx)=ac+(ad+bc)x+bdx2.
Consider the two arbitrary complex numbers a+bi and c+di.When a+bi and c+di are multiplied using the distributive property of multiplication, the product is (a+bi)(c+di)=ac+adi+bci+bdi2.
Using the value of i2 and simplifying the expression, the resulting expression is (a+bi)(c+di)=ac+(ad+bc)i−bd.
The right-hand side of the equation can be grouped as (ac−bd)+(ad+bc)i, which is a complex number.
It can be observed that in the multiplication of two binomial linear expressions in the same variable, and in the multiplication of two complex numbers, first the distributive property of multiplication is used, and then the like terms are grouped and simplified.
Also, it can be observed that in the multiplication of two complex numbers, the result obtained after using the distributive property of multiplication and grouping the terms can be simplified further using the value i2=−1.
The product of two binomial linear expressions lacks the square of the imaginary unit, that is i2, and thus, it cannot be simplified further.
The similarities between multiplying two complex numbers and multiplying two binomial linear expressions in the same variable are:
Both involve the application of the distributive property of multiplication (a+b)(c+d)=ac+ad+bc+bd
.Both involve grouping and combining the like terms after using the distributive property of multiplication.
The difference between multiplying two complex numbers and multiplying two binomial linear expressions in the same variable is that the result obtained after using the distributive property of multiplication and grouping the terms in the multiplication of two complex numbers can be simplified further using the value i2=−1.
This is not possible when multiplying two binomial linear expressions because their product does not contain the square i2.
Page 133 Exercise 1 Answer
The given binomials are 3+2x and 4−5x.
The question requires to determine the sum of the given binomials, and then explain how the sum can be used to find the sum of the complex numbers 3+2i and 4−5i.
To add the binomials, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Then, replace the variable x by the imaginary unit i on both sides of the given equation to find the sum of the complex numbers 3+2i and 4−5i.
Write the sum of 3+2x and 4−5x, group the like terms in parentheses, and combine the like terms in the parentheses.
(3+2x)+(4−5x)=(3+4)+(2x+(−5x))
(3+2x)+(4−5x)=(3+4)+(2x−5x)
(3+2x)+(4−5x)=7−3x
Thus, the required sum of the given binomials is (3+2x)+(4−5x)=7−3x.
The sum of the complex numbers 3+2i and 4−5i can be obtained by substituting the variable x by the imaginary unit i in the equation (3+2x)+(4−5x)=7−3x.
Substitute i for x on both sides of (3+2x)+(4−5x)=7−3x.
(3+2i)+(4−5i)=7−3i
Thus, the sum of the complex numbers is (3+2i)+(4−5i)=7−3i.
The required sum of the given binomials is (3+2x)+(4−5x)=7−3x.
The sum of the complex numbers 3+2i and 4−5i can be determined by substituting i
for x on both sides of the equation for the sum of the given binomials, that is in the equation (3+2x)+(4−5x)=7−3x.
The resulting sum of the complex numbers 3+2i and 4−5i is (3+2i)+(4−5i)=7−3i.
Page 133 Exercise 2 Answer
The given number is 5+i, which can be written as the complex number 5+1⋅i.Therefore, the real part of 5+i is 5 and the imaginary part of 5+i is 1.
Since the given number can be written as the complex number 5+1⋅i having nonzero real and imaginary parts, it belongs only to the set of complex numbers.
The real part and imaginary part of 5+i are 5 and 1 respectively.
The given number 5+i belongs to only the set of complex numbers.
Page 133 Exercise 3 Answer
The given number is 7−6i, which can be written as the complex number 7+(−6)i.
Therefore, the real part of 7−6i is 7 and the imaginary part of 7−6i is −6.
Since the given number can be written as the complex number 7+(−6)i having nonzero real and imaginary parts, it belongs only to the set of complex numbers.
The real part and imaginary part of 7−6i are 7 and −6 respectively.
The given number 7−6i belongs to only the set of complex numbers.
Page 134 Exercise 4 Answer
The given number is 25, which can be written as the complex number 25+0i.Therefore, the real part of 25 is 25 and the imaginary part of 25 is 0.
Since the given number can be written as the complex number 25+0i having the imaginary part 0, it belongs to the set of real numbers and the set of complex numbers.
The real part and imaginary part of 25 are 25 and 0 respectively.
The given number 25 belongs to the set of real numbers and the set of complex numbers.
Page 134 Exercise 5 Answer
The given number is i√21, which can be written as the complex number 0+(√21)i
.Therefore, the real part of i√21 is 0 and the imaginary part of i√21 is √21
.Since the given number can be written as the complex number 0+(√21)i
having the real part 0, it belongs to the set of imaginary numbers and the set of complex numbers.
The real part and imaginary part of i√21 are 0 and √21 respectively.
The given number i√21 belongs to the set of imaginary numbers and the set of complex numbers.
Page 134 Exercise 6 Answer
The given sum is (3+4i)+(7+11i).
The question requires to add the complex numbers in the given sum.
To add the complex numbers, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Group the real and imaginary parts in the sum (3+4i)+(7+11i), and then combine the like terms.
(3+4i)+(7+11i)=(3+7)+(4i+11i)
=10+15i
Thus, the required sum is 10+15i.
The required sum is 10+15i.
Page 134 Exercise 7 Answer
The given sum is (−1−i)+(−10+3i).
The question requires to add the complex numbers in the given sum.
To add the complex numbers, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Group the real and imaginary parts in the sum (−1−i)+(−10+3i), and then combine the like terms.
(−1−i)+(−10+3i)=(−1+(−10))+(−i+3i)
=(−1−10)+(−i+3i)
=−11+2i
Thus, the required sum is −11+2i.
The required sum is −11+2i.
Page 133 Exercise 8 Answer
The given sum is (−9−7i)+(6+5i).
The question requires to add the complex numbers in the given sum.
To add the complex numbers, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Group the real and imaginary parts in the sum (−9−7i)+(6+5i), and then combine the like terms.
(−9−7i)+(6+5i)=(−9+6)+(−7i+5i)
=−3−2i
Thus, the required sum is −3−2i.
The required sum is −3−2i.
Page 134 Exercise 9 Answer
The given difference is (2+3i)−(7+6i).
The question requires to subtract the complex numbers in the given difference.
To subtract the complex numbers, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Rewrite the subtraction of the complex numbers in (2+3i)−(7+6i) as addition.
Then, group the real and imaginary parts, and combine the like terms.
(2+3i)−(7+6i)=(2+3i)+(−7−6i)
=(2−7)+(3i−6i)
=−5−3i
Thus, the required difference is −5−3i.
The required difference is −5−3i.
Page 134 Exercise 10 Answer
The given difference is (4+5i)−(14−i).
The question requires to subtract the complex numbers in the given difference.
To subtract the complex numbers, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Rewrite the subtraction of the complex numbers in (4+5i)−(14−i) as addition.
Then, group the real and imaginary parts, and combine the like terms.
(4+5i)−(14−i)=(4+5i)+(−14+i)
=(4−14)+(5i+i)
=−10+6i
Thus, the required difference is −10+6i.
The required difference is −10+6i.
Page 134 Exercise 11 Answer
The given difference is (−8−3i)−(−9−5i).The question requires to subtract the complex numbers in the given difference.
To subtract the complex numbers, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Rewrite the subtraction of the complex numbers in (−8−3i)−(−9−5i)
as addition. Then, group the real and imaginary parts, and combine the like terms.
(−8−3i)−(−9−5i)=(−8−3i)+(9+5i)
=(−8+9)+(−3i+5i)
=1+2i
Thus, the required difference is 1+2i.
The required difference is 1+2i.
Page 134 Exercise 12 Answer
The given difference is (5+2i)−(5−2i).
The question requires to subtract the complex numbers in the given difference.
To subtract the complex numbers, rewrite the difference as a sum, group the like terms in the sum using parentheses, and then combine the like terms in the expression.
Rewrite the subtraction of the complex numbers in (5+2i)−(5−2i) as addition.
Then, group the real and imaginary parts, and combine the like terms.
(5+2i)−(5−2i)=(5+2i)+(−5+2i)
=(5−5)+(2i+2i)
=0+4i
=4i
Thus, the required difference is 4i.
The required difference is 4i.
Page 134 Exercise 13 Answer
The given product is (2+3i)(3+5i).
The question requires to multiply the complex numbers in the given product.
To multiply the complex numbers, use the distributive property of multiplication and the value of i2, and simplify the expression.
Apply the distributive property of multiplication in the given product (2+3i)(3+5i), and then combine the like terms.
(2+3i)(3+5i)=6+10i+9i+15i2
=6+19i+15i2
Substitute −1 for i2, and then simplify the expression.
(2+3i)(3+5i)=6+19i+15(−1)
=6+19i−15
=−9+19i
Thus, the required product is −9+19i.
The required product is −9+19i.
Page 134 Exercise 14 Answer
The given product is (7+i)(6−9i).
The question requires to multiply the complex numbers in the given product.
To multiply the complex numbers, use the distributive property of multiplication and the value of i2, and simplify the expression.
Apply the distributive property of multiplication in the given product (7+i)(6−9i), and then combine the like terms.
(7+i)(6−9i)=42−63i+6i−9i2
=42−57i−9i2
Substitute −1 for i2, and then simplify the expression.
(7+i)(6−9i)=42−57i−9(−1)
=42−57i+9
=51−57i
Thus, the required product is 51−57i.
The required product is 51−57i.
Page 134 Exercise 15 Answer
The given product is (4−i)(4+i).
The question requires to multiply the complex numbers in the given product.
To multiply the complex numbers, use the distributive property of multiplication and the value of i2, and simplify the expression.
Apply the distributive property of multiplication in the given product (4−i)(4+i), and then combine the like terms.
(4−i)(4+i)=16+4i−4i−i2=16−i2
Substitute −1 for i2, and then simplify the expression.
(4−i)(4+i)=16−(−1)
=16+1
=17
Thus, the required product is 17.
The required product is 17.
Page 135 Exercise 16 Answer
The given diagram shows the components in a circuit. The given current is 12+36i amps.
The question requires to determine the total impedance, and then determine the voltage for each component of the circuit.
To determine the total impedance and voltage of each component, use the diagram to write the impedance for each component as a complex number.
Then, add the complex numbers to determine the total impedance.
Next, use the impedance and current in the Ohm’s law to determine the required voltage for each component.
From the circuit diagram, it can be observed that there is one resistor and one capacitor.
The resistor has the impedance 1 ohm. Therefore, the impedance for the resistor can be represented by the complex number 1.
The capacitor has the impedance 3 ohms. Therefore, the impedance for the capacitor can be represented by the complex number −3i.
The total impedance for the circuit is equal to the sum of the impedances for the two components.
Calculate the sum of the impedances for the two components.
1+(−3i)=1−3i
Thus, the total impedance is 1−3i ohms.
Substitute 12+36i for I and 1 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(12+36i)(1)
=12+36i
Thus, the voltage for the resistor is 12+36i volts.
Substitute 12+36i for I and −3i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(12+36i)(−3i)
=−36i−108i2
=−36i−108(−1)
=108−36i
Thus, the voltage for the capacitor is 108−36i volts.
The required total impedance is 1−3i ohms.
The voltages for the resistor and the capacitor are 12+36i volts and 108−36i volts respectively.
Page 135 Exercise 17 Answer
The given diagram shows the components in a circuit. The given current is 19.2−14.4i amps.
The question requires to determine the total impedance, and then determine the voltage for each component of the circuit.
To determine the total impedance and voltage of each component, use the diagram to write the impedance for each component as a complex number.
Then, add the complex numbers to determine the total impedance.
Next, use the impedance and current in the Ohm’s law to determine the required voltage for each component.
From the circuit diagram, it can be observed that there is one resistor and one inductor.
The resistor has the impedance 4 ohms. Therefore, the impedance for the resistor can be represented by the complex number 4.
The inductor has the impedance 3 ohms. Therefore, the impedance for the inductor can be represented by the complex number 3i.
The total impedance for the circuit is equal to the sum of the impedances for the two components.
Write the sum of the impedances for the two components.
(4)+(3i)=4+3i
Thus, the total impedance is 4+3i ohms.
Substitute 19.2−14.4i for I and 4 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(19.2−14.4i)(4)
=76.8−57.6i
Thus, the voltage for the resistor is 76.8−57.6i volts.
Substitute 19.2−14.4i for I and 3i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(19.2−14.4i)(3i)
=57.6i−43.2i2
=57.6i−43.2(−1)
=43.2+57.6i
Thus, the voltage for the inductor is 43.2+57.6i volts.
The required total impedance is 4+3i ohms.
The voltages for the resistor and the inductor are 76.8−57.6i volts and 43.2+57.6i
volts respectively.
Page 135 Exercise 18 Answer
The given diagram shows the components in a circuit. The given current is 7.2+9.6i amps.
The question requires to determine the total impedance, and then determine the voltage for each component of the circuit.
To determine the total impedance and voltage of each component, use the diagram to write the impedance for each component as a complex number.
Then, add the complex numbers to determine the total impedance.
Next, use the impedance and current in the Ohm’s law to determine the required voltage for each component.
From the circuit diagram, it can be observed that there is one resistor, one inductor, and one capacitor.
The resistor has the impedance 6 ohms. Therefore, the impedance for the resistor can be represented by the complex number 6.
The inductor has the impedance 2 ohms. Therefore, the impedance for the inductor can be represented by the complex number 2i.
The capacitor has the impedance 10 ohms. Therefore, the impedance for the capacitor can be represented by the complex number −10i.
The total impedance for the circuit is equal to the sum of the impedances for the three components.
Calculate the sum of the impedances for the three components.
6+2i+(−10i)=6+(2i−10i)
=6−8i
Thus, the total impedance is 6−8i ohms.
Substitute 7.2+9.6i for I and 6 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(7.2+9.6i)(6)
=43.2+57.6i
Thus, the voltage for the resistor is 43.2+57.6i volts.
Substitute 7.2+9.6i for I and 2i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(7.2+9.6i)(2i)
=14.4i+19.2i2
=14.4i+19.2(−1)
=−19.2+14.4i
Thus, the voltage for the inductor is −19.2+14.4i volts.
Substitute 7.2+9.6i for I and −10i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(7.2+9.6i)(−10i)
=−72i−96i2
=−72i−96(−1)
=96−72i
Thus, the voltage for the capacitor is 96−72i volts.
The required total impedance is 6−8i ohms.
The voltages for the resistor, the inductor, and the capacitor are 43.2+57.6i volts, −19.2+14.4i
volts, and 96−72i volts respectively.
Page 135 Exercise 19 Answer
The given diagram shows the components in a circuit. The given current is 16.8+2.4i amps.
The question requires to determine the total impedance, and then determine the voltage for each component of the circuit.
To determine the total impedance and voltage of each component, use the diagram to write the impedance for each component as a complex number.
Then, add the complex numbers to determine the total impedance.
Next, use the impedance and current in the Ohm’s law to determine the required voltage for each component.
From the circuit diagram, it can be observed that there is one resistor, one inductor, and one capacitor.
The resistor has the impedance 7 ohms. Therefore, the impedance for the resistor can be represented by the complex number 7.
The inductor has the impedance 3 ohms. Therefore, the impedance for the inductor can be represented by the complex number 3i.
The capacitor has the impedance 4 ohms. Therefore, the impedance for the capacitor can be represented by the complex number −4i.
The total impedance for the circuit is equal to the sum of the impedances for the three components.
Calculate the sum of the impedances for the three components.
7+3i+(−4i)=7+(3i−4i)
=7−i
Thus, the total impedance is 7−i ohms.
Substitute 16.8+2.4i for I and 7 for Z in the Ohm’s law V=I⋅Z, and multiply the terms.
V=(16.8+2.4i)(7)
=117.6+16.8i
Thus, the voltage for the resistor is 117.6+16.8i volts.
Substitute 16.8+2.4i for I and 3i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(16.8+2.4i)(3i)
=50.4i+7.2i2
=50.4i+7.2(−1)
=−7.2+50.4i
Thus, the voltage for the inductor is −7.2+50.4i volts.
Substitute 16.8+2.4i for I and −4i for Z in the Ohm’s law V=I⋅Z, and multiply the terms, and substitute −1 for i2.
V=(16.8+2.4i)(−4i)
=−67.2i−9.6i2
=−67.2i−9.6(−1)
=9.6−67.2i
Thus, the voltage for the capacitor is 9.6−67.2i volts.
The required total impedance is 7−i ohms.
The voltages for the resistor, the inductor, and the capacitor are 117.6+16.8i volts, −7.2+50.4i volts, and 9.6−67.2i volts respectively.
Page 136 Exercise 20 Answer
The given expressions are (A) (3−5i)(3+5i), (B) (3+5i)(3+5i), (C) (−3−5i)(3+5i), and (D) (3−5i)(−3−5i).
The given products are −16+30i, −34, 34, and 16−30i.
The question requires to match the given products on the right with their corresponding expressions on the left.
To match the products with the expressions, simplify the expressions using the distributive property of multiplication and the value of i2.
Then, match the obtained products with the expressions.
Apply the distributive property of multiplication in the product (3−5i)(3+5i), combine the like terms, substitute −1 for i2, and then simplify the expression.
(3−5i)(3+5i)=9+15i−15i−25i2
=9−25i2
=9−25(−1)
=9+25
=34
Thus, the correct match for the product 34 is the expression (A) (3−5i)(3+5i).
Apply the distributive property of multiplication in the product (3+5i)(3+5i), combine the like terms, substitute −1 for i2, and then simplify the expression.
(3+5i)(3+5i)=9+15i+15i+25i2
=9+30i+25i2
=9+30i+25(−1)
=9+30i−25
=−16+30i
Thus, the correct match for the product −16+30i is the expression (B) (3+5i)(3+5i).
Apply the distributive property of multiplication in the product (−3−5i)(3+5i), combine the like terms, substitute −1 for i2, and then simplify the expression.
(−3−5i)(3+5i)=−9−15i−15i−25i2
=−9−30i−25i2
=−9−30i−25(−1)
=−9−30i+25
=16−30i
Thus, the correct match for the product 16−30i is the expression (C) (−3−5i)(3+5i).
Apply the distributive property of multiplication in the product (3−5i)(−3−5i), combine the like terms, substitute −1 for i2, and then simplify the expression.
(3−5i)(−3−5i)=−9−15i+15i+25i2
=−9+25i2
=−9+25(−1)
=−9−25
=−34
Thus, the correct match for the product −34 is the expression (D) (3−5i)(−3−5i).
The correct match for the product −16+30i is the expression (B) (3+5i)(3+5i).
The correct match for the product −34 is the expression (D) (3−5i)(−3−5i).
The correct match for the product 34 is the expression (A) (3−5i)(3+5i).
The correct match for the product 16−30i is the expression (C) (−3−5i)(3+5i).
Page 137 Exercise 21 Answer
The given complex numbers are √3+i√3 and −√3−i√3.
The question requires to prove that √3+i√3 and −√3−i√3 are the square roots of 6i.
To prove the equation, calculate the square of √3+i√3 using the distributive property of multiplication and the value of i2.
Then, use the definition of square root to prove that √3+i√3
is the square root of 6i. Similarly, calculate the square of −√3−i√3 and use the definition of square root to prove that −√3−i√3 is the square root of 6i.
Calculate the square of √3+i√3 using the distributive property of multiplication.
Then, combine the like terms, substitute −1 for i2, and then simplify the expression.
(√3+i√3)2
=(√3+i√3)(√3+i√3)
=3+3i+3i+3i2
=3+6i+3(−1)
=3+6i−3
=6i
According to the definition of square root, if (√3+i√3)2=6i, then the expression √3+i√3 is the square root of 6i.
Hence, it is proved that √3+i√3 is the square root of 6i.
Calculate the square of −√3−i√3 using the distributive property of multiplication.
Then, combine the like terms, substitute −1 for i2, and then simplify the expression.
(−√3−i√3)2
=(−√3−i√3)(−√3−i√3)
=3+3i+3i+3i2
=3+6i+3(−1)
=3+6i−3 =6i
According to the definition of square root, if (−√3−i√3)2=6i, then the expression −√3−i√3 is the square root of 6i.
Hence, it is proved that −√3−i√3 is the square root of 6i.
It is proved that √3+i√3 and −√3−i√3 are the square roots of 6i.
Page 137 Exercise 22 Answer
Two complex numbers are given which differ only in the sign of their imaginary parts.
The question requires to determine what type of number is the product of two complex numbers that differ only in the sign of their imaginary parts.
Also, it is required to prove the written conjecture.
To answer the question, take two arbitrary complex numbers that differ only in the sign of their imaginary parts.
Then, write their product as a difference of squares, and use the value of i2 to determine what type of number the product is.
Next, calculate the product of the two complex numbers using the distributive property of multiplication and the value of i2 to prove the conjecture.
Let the two complex numbers be a+bi and a−bi which differ only in the sign of their imaginary parts.
The product of a+bi and a−bi can be written as the difference of squares a2−(bi)2, which can be simplified as a2−b2i .
The expressions a2 and b2i2 are real because a and b are real, and i2 is equal to −1. This means that the difference of squares a2−(bi)2 is a real number.
Therefore, the product of a+bi and a−bi is a real number.
Thus, the product of two complex numbers that differ only in the sign of their imaginary parts is a real number.
Write the product of a+bi and a−bi, and apply the distributive property of multiplication. Then, combine the like terms, substitute −1 for i2, and then simplify the expression.
(a+bi)(a−bi)=a2−abi+abi−b2i2
=a2−b2i2
=a2−b2(−1)
=a2+b2
The product a2+b2 is real because a and b are real.
Therefore, the product of the complex numbers a+bi and a−bi is a real number.
Hence, it is proved that the product of two complex numbers that differ only in the sign of their imaginary parts is a real number.
The product of two complex numbers that differ only in the sign of their imaginary parts is a real number.
The conjecture ‘the product of two complex numbers that differ only in the sign of their imaginary parts is a real number’ is proved.
Page 138 Exercise 23 Answer
The given table shows a sequence defined by the recursive rule f(n+1)=(f(n))2+i, where f(0) is equal to 1.
The question requires to generate the first few numbers of the sequence and record the results in the given table.
To determine the few numbers and record the results, substitute 0 for n in the recursive rule, and use the value of f(0) to simplify and obtain the number f(1).
Similarly, substitute n as 1, 2, and 3, and solve the recursive rule using the value of f(n) to find the next three numbers of the sequence.
Finally, record the calculations and results in the given table.
The value of f(0) is equal to 1.
Substitute 0 for n in the recursive rule f(n+1)=(f(n))2+i. Then, substitute 1 for f(0), and simplify the expression.
f(0+1)=(f(0))2+i
f(1)=(1)2+i
f(1)=1+i
Substitute 1 for n in the recursive rule f(n+1)=(f(n))2+i. Then, substitute 1+i for f(1), −1 for i2, and simplify the expression.
f(1+1)=(f(1))2+i
f(2)=(1+i)2+i
f(2)=1+2i+i2+i
f(2)=1+2i+(−1)+i
f(2)=3i
Substitute 2 for n in the recursive rule f(n+1)=(f(n))2+i. Then, substitute 3i for f(2), −1 for i2, and simplify the expression.
f(2+1)=(f(2))2+i
f(3)=(3i)2+i
f(3)=9i2+i
f(3)=9(−1)+i
f(3)=−9+i
Substitute 3 for n in the recursive rule f(n+1)=(f(n))2+i. Then, substitute −9+i for f(3), −1 for i2, and simplify the expression.
f(3+1)=(f(3))2+i
f(4)=(−9+i)2+i
f(4)=81−18i+i2+i
f(4)=81−18i+(−1)+i
f(4)=80−17i
Record the results by completing the given table using the calculations performed and the num
The first few numbers of the sequence are recorded in the given table as shown:
Page 138 Exercise 24 Answer
The given sequence is defined by f(0)=1 and the recursive rule f(n+1)=(f(n))2+i. It is given that if the magnitudes of the numbers increase without bound, the number f(0) equal to 1
does not belong to the “filled-in” Julia set.
The question requires to use the completed table for f(0)=1 to determine whether the number belongs to the “filled-in” Julia set corresponding to c equal to i or not.
Given the completed table for f(0)=1, as calculated in the previous part of this exercise.
To answer the question, write the number f(0)=1 and the next four numbers in the sequence from the table, and calculate their magnitudes.
Then, observe the magnitudes to determine whether the magnitudes increase without bound, and use the observation to determine if the number f(0)=1
belongs to the “filled-in” Julia set corresponding to c equal to i or not.
The value of f(0) is equal to 1, which can be written as the complex number 1+0i.
Calculate the magnitude of f(0) using the formula √a2+b2, and simplify the expression.
√a2+b2=√12+02
=√1+0
=√1
=±1
The magnitude is equal to 1 because it cannot be negative.
The value of f(1) is equal to 1+i.
Calculate the magnitude of f(1) using the formula √a2+b2, and simplify the expression.
√a2+b2=√12+12
=√1+1
=√2
The value of f(2) is equal to 3i, which can be written as the complex number 0+3i.
Calculate the magnitude of f(2) using the formula √a2+b2, and simplify the expression.
√a2+b2=√02+32
=√0+9
=√9
=±3
The magnitude is equal to 3 because it cannot be negative.
The value of f(4) is equal to 80−17i.
Calculate the magnitude of f(4) using the formula √a2+b2, and simplify the expression.
√a2+b2=√802+(−17)2
=√6400+289
=√6689 ≈ ±81.8
The magnitude is equal to 81.8 because it cannot be negative.
From the magnitudes of the first few generated numbers, it can be observed that there is no bound on the value of the magnitudes as n increases.
Since the magnitudes increase without bound, the number f(0)=1 does not belong to the “filled-in” Julia set corresponding to c equal to i.
No, the number f(0)=1 does not belong to the “filled-in” Julia set corresponding to c
equal to i because for the numbers of the sequence determined in the table, the magnitudes increase without bound.
Page 138 Exercise 25 Answer
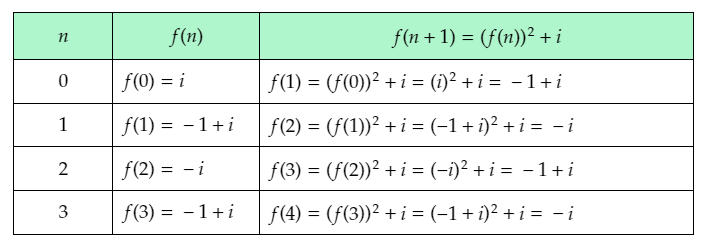
The given sequence is defined by f(0)=i and the recursive rule f(n+1)=(f(n))2+i.
The question requires to determine whether the number f(0)=i belongs to the “filled-in” Julia set corresponding to c equal to i or not.
To determine the first few numbers of the sequence, substitute 0 for n in the recursive rule, and use the value of f(0) to simplify and obtain the number f(1).
Similarly, substitute n as 1, 2, and 3, and solve the recursive rule using the value of f(n) to find the next three numbers of the sequence.
Finally, record the calculations and results in a table.
To answer the question, calculate the magnitudes of the number f(0)=i and the next four numbers in the sequence from the table.
Then, observe the magnitudes to determine whether the magnitudes increase without bound, and use the observation to determine if the number f(0)=i belongs to the “filled-in” Julia set corresponding to c equal to i or not.
The value of f(0) is equal to i.Substitute 0 for n in the recursive rule f(n+1)=(f(n))2+i.
Then, substitute i for f(0), and −1 for i2.
f(0+1)=(f(0))2+i
f(1)=(i)2+i
f(1)=i2+i
f(1)=−1+i
Substitute 1 for n in the recursive rule f(n+1)=(f(n))2+i.
Then, substitute −1+i for f(1), −1 for i2, and simplify the expression.
f(1+1)=(f(1))2+i
f(2)=(−1+i)2+i
f(2)=1−2i+i2+i
f(2)=1−2i+(−1)+i
f(2)=−i
Substitute 2 for n in the recursive rule f(n+1)=(f(n))2+i.
Then, substitute −i for f(2), and −1 for i2.
f(2+1)=(f(2))2+i
f(3)=(−i)2+i
f(3)=i2+i
f(3)=−1+i
Substitute 3 for n in the recursive rule f(n+1)=(f(n))2+i.
Then, substitute −1+i for f(3), −1 for i2, and simplify the expression.
f(3+1)=(f(3))2+i
f(4)=(−1+i)2+i
f(4)=1−2i+i2+i
f(4)=1−2i+(−1)+i
f(4)=−i
Record the results by constructing a table with the columns for values of n, f(n), and f(n+1).
The value of f(0) is equal to i, which can be written as the complex number 0+1⋅i.
Calculate the magnitude of f(0) using the formula √a2+b2, and simplify the expression.
√a2+b2=√02+12
=√0+1
=√1
=±1
The magnitude is equal to 1 because it cannot be negative.
The value of f(1) is equal to −1+i.
Calculate the magnitude of f(1) using the formula √a2+b2, and simplify the expression.
√a2+b2=√(−1)2+12
=√1+1
=√2
The value of f(2) is equal to −i, which can be written as the complex number 0+(−1)i.
Calculate the magnitude of f(2) using the formula √a2+b2, and simplify the expression.
√a2+b2=√02+(−1)2
=√0+1
=√1
=±1
The magnitude is equal to 1 because it cannot be negative.
The value of f(3) is equal to −1+i.
Calculate the magnitude of f(3) using the formula √a2+b2, and simplify the expression.
√a2+b2=√(−1)2+12
=√1+1
=√2
The value of f(4) is equal to −i, which can be written as the complex number 0+(−1)i.
Calculate the magnitude of f(4) using the formula √a2+b2, and simplify the expression.
√a2+b2=√02+(−1)2
=√0+1
=√1
=±1
The magnitude is equal to 1 because it cannot be negative.
From the magnitudes of the first few generated numbers, it can be observed that the magnitudes are bounded.
The values of the magnitudes are either 1 or √2. Therefore, the magnitudes are bounded at √2.
Since the magnitudes remain bounded, the number f(0)=i belongs to the “filled-in” Julia set corresponding to c equal to i.
Yes, the number f(0)=i belongs to the “filled-in” Julia set corresponding to c equal to i because the magnitudes for the numbers of the sequence remain bounded at √2.
The values of the magnitudes are either 1 or √2.