Envision Math Grade 8 Volume 1 Chapter 6 Congruence And Similarity
Page 309 Focus On Math Practices Answer
We can describe Maria’s change in position, when car returns to the position at which she began the ride, with rotation.
Because, a rotation is a transformation that turns a figure around a fixed point, called the center of rotation. And as we can see in the picture, each car turns around central part of the Ferris wheel.
If we want to come back to the start position, this means that we have to go through an entire drive, or an entire circle.
This transformation can be described by rotation for 360∘.
This transformation can be described by rotation for 360∘
Page 310 Essential Question Answer
Given:
The two dimensional figure.
To find:
How does a rotation affect the properties of a two-dimensional figure?
A rotation is a transformation that turns a figure around a fixed point, called the center of rotation.
There are not change in shape, side lengths, angle measures and orientation between resulting image and original figure by rotation.
The only difference, after rotation, is in x and y values in ordered pairs that represent the coordinates of vertices.
There are not change in shape, side lengths, angle measures and orientation between resulting image and original figure by rotation.
The only difference, after rotation, is in x and y values in ordered pairs that represent the coordinates of vertices.

Envision Math Grade 8 Volume 1 Chapter 6.4 Solutions
Page 310 Try It Answer
Given:
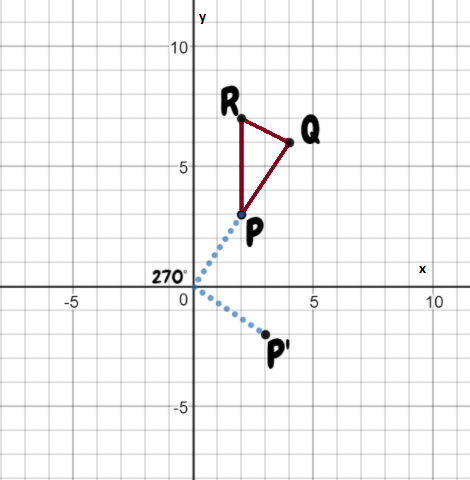
The architect continues to rotate the umbrella in a counter clockwise direction.
To find:
What is the angle of this rotation?
Rotation for 360∘ (one complete circle around) means turning around until we point in the same direction again.
If we want to determined the measure of angle of rotation to the original position, we have to find the value r
that is true for the equation:
90∘ + r = 360∘
Notice that, rotation for 360∘ (one complete circle around) means turning around until we point in the same direction again.
And in the equation we add 90∘, because we do not rotate from the start position. The umbrella has been rotated already for 90∘.
90∘ + r = 360∘
r = 270∘
Subtract from the both sides by 90∘
Therefore, the angle of this rotation is 270∘
Therefore, the angle of this rotation is 270∘.
Congruence And Similarity Envision Math Exercise 6.4 Answers
Page 311 Try It Answer
Given:
The coordinates of the vertices of quadrilateral HIJK are H(1,4), I(3,2), J(−1,−4), and K(−3,−2)).
To find:
If quadrilateral is rotated 270∘ about the origin, what are the vertices of the resulting image, quadrilateral H′I′J′K′
We use the concept that:
The rule for a rotation by 270∘ about the origin is
(x, y) → (y,−x).
There are some rules for x – and y – coordinates of a point at rotation for 270∘
In another words, when we rotate for 270∘, the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before second coordinate:
(x, y)→(y,−x)
Therefore, the vertices of quadrilateral H′I′J′K′ are:
H(1,4) → H′(4,−1)
I(3,2) → I′(2,−3)
J(−1,−4) → J′(−4,1)
K(−3,−2) → K′(−2,3)
The vertices of quadrilateral H′I′J′K′ are
H′(4,−1)
I′(2,−3)
J′(−4,1)
K′(−2,3)
Page 311 Try It Answer
Given:
A figure with two triangles.
To find:
The description of the rotation that maps ΔFGH to ΔF′G′H′
We find the number of degrees that figure rotates.
To describe the rotation that maps triangle FGH to triangle F′G′H′, we have to find the angle of rotation. In another words, we have to find the number of degrees that figure rotates.
The procedure is simple:
1. Draw rays from the origin through point G and point G′.(Notice that, we can choose any point of the figure, because all point are rotated for the same angle.)
2. Measure the angle formed by rays.
(Use a protractor to determine the number of degrees.)
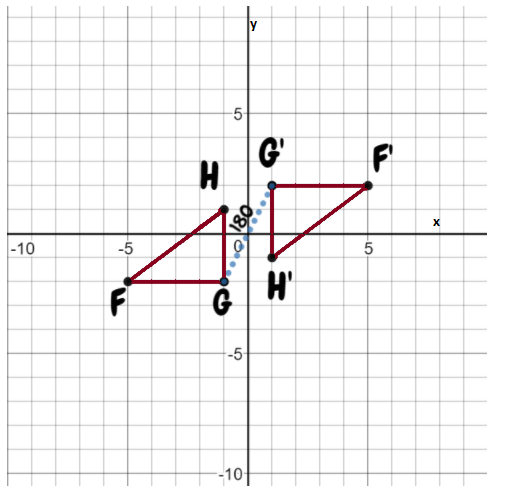
Hence, a 180∘ rotation about origin maps ΔFGH to ΔF′G′H′.
Envision Math Grade 8 Chapter 6.4 Explained
Envision Math Grade 8 Volume 1 Student Edition Solutions Chapter 6 Congruence And Similarity Exercise 6.3 Page 312 Exercise 1 Answer
Given:
A rotation of two dimensional figure.
To find:
How does a rotation affect the properties of a two-dimensional figure?
A rotation is a transformation that turns a figure around a fixed point, called the center of rotation.
There are not change in shape, side lengths, angle measures and orientation between resulting image and original figure by rotation.
The only difference ,after rotation, is in x and y values in ordered pairs that represent the coordinates of vertices.
The only difference, after rotation, is in x and y values in ordered pairs that represent the coordinates of vertices.
Page 312 Exercise 2 Answer
Given:
If a preimage is rotated 360 degrees about the origin
To find:
How can you describe its image?
We use the fact that 360 degrees about the origin means turning around until you point in the same direction again.
So, when we rotated some figure 360∘ about the origin, we actually put the figure on the same position. Like we did not even change it.
Therefore, the image will be the same as the preimage.
Therefore, the image will be the same as the preimage.
Page 312 Exercise 3 Answer
Given: AB is parallel to DC
To find:
How are sides A′B′ related to C′D′.
There are not change in shape, side lengths, angle measures and orientation between resulting image and the final original figure by rotation.
The only difference, after rotation, is in x and y values in ordered pairs that represent the coordinates of vertices.
Therefore, if side AB is parallel to side CD on the preimage, the side A′B′ and C′D′ will also be parallel.
Because side lengths and shape remain the same after rotation.
Therefore, if side AB is parallel to side CD on the preimage, the side A′B′ and C′D′ will also be parallel. Because side lengths and shape remain the same after rotation.
Page 312 Exercise 4 Answer
Given:
The coordinates of the vertices of rectangle ABCD are
A(3,−2), B(3,2), C(−3,2), and D(−3,−2).
To find:
The coordinates of the vertices A′B′C′D′.
when we rotate for 90∘, the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before first coordinate:
There are some rules for x – and y – coordinates of a point at rotation for 90∘. In another words, when we rotate for 90∘, the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before first coordinate:
(x, y) → (−y, x)
Therefore, the vertices of rectangle A′B′C′D′ are:
A(3,−2)→A′(2,3)
B(3,2)→B′(−2,3)
C(−3,2)→C′(−2,−3)
D(−3,−2)→D′(2,−3)
The vertices of rectangle A′B′C′D′
A′(2,3)
B′(−2,3)
C′(−2,−3)
D′(2,−3)
Given:
The coordinates of the vertices of rectangle ABCD are A(3,−2), B(3,2), C(−3,2), and D(−3,−2).
To find:
The measures of angles of A′B′C′D′
As we already said, the rotation is a transformation that turns a figure around a fixed point, called the center of rotation. There are not change in shape, side lengths, angle measures and orientation between resulting image and original figure by rotation.
The only difference, after rotation, is in x and y values in ordered pairs that represent the coordinates of vertices.
Therefore, the measures of the angles of A′B′C′D′ will be the same as the measures of the angles of A′B′C′D′.
To be precise, we know that figure A′B′C′D′ is also rectangle, because the rotation does not change the shape of figures.
So, as rectangle has four right angles, the measures of all angles will be 90∘.
So, as rectangle has four right angles, the measures of all angles will be 90∘.
Solutions For Envision Math Grade 8 Exercise 6.4
Envision Math Grade 8 Volume 1 Student Edition Solutions Chapter 6 Congruence And Similarity Exercise 6.3 Page 312 Exercise 5 Answer
Given:
Graph with two triangles.
To find:
Counterclockwise rotation of ΔQRS.
We use the concept of rotation to solve the question.
To describe the rotation that maps triangle QRS to triangle Q′R′S′, we have to find the angle of rotation. In another words, we have to find the number of degrees that figure rotates.
The procedure is simple:
1. Draw rays from the origin through point Q and point Q′.(Notice that, we can choose any point of the figure, because all point are rotated for the same angle.)
2. Measure the angle formed by rays.
(Use a protractor to determine the number of degrees.)
Hence, 270∘ rotation about origin maps QRS to Q′R′S′.
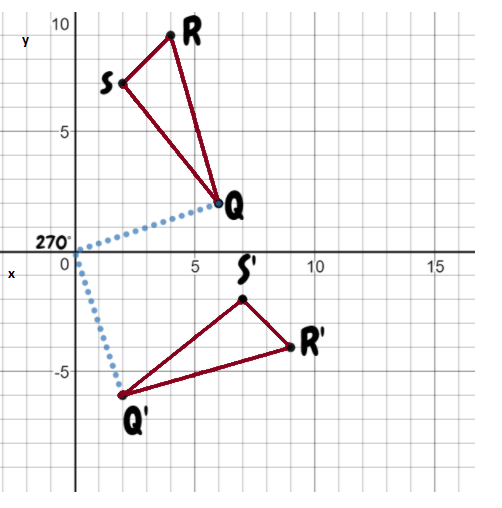
Hence, 270∘ rotation about origin maps QRS to Q′R′S′.
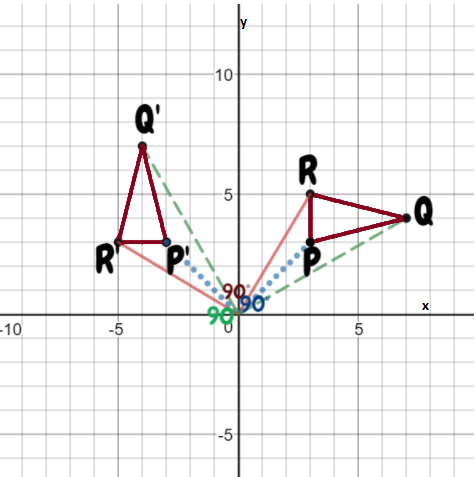
Page 313 Exercise 6 Answer
Given:
Graph with two triangles.
To find:
The angle of rotation of the given maps about origin.
A rotation is a transformation that turns a figure around a fixed point, called the center of rotation.
To describe the rotation that maps triangle PQR to triangle P′Q′R′, we have to find the angle of rotation. In another words, we have to find the number of degrees that figure rotates. The procedure is simple:
1. Draw rays from the origin through point Q and point Q′.(Notice that, we can choose any point of the figure because all point are rotated for the same angle.)
2. Measure the angle formed by rays.
(Use a protractor to determine the number of degrees.)
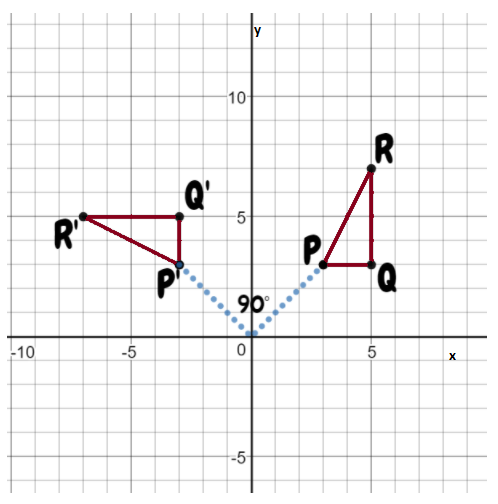
The angle of rotation about origin maps triangle PQR to triangle P′Q′R is 90∘
The angle of rotation about origin maps triangle PQR to triangle P′Q′R is 90∘.
Envision Math Grade 8 Volume 1 Chapter 6.4 Practice Problems
Page 313 Exercise 7 Answer
Given:
Graph with two triangles.
To find:
Is ΔXYZ rotation of ΔX′Y′Z′.
A rotation is a transformation that turns a figure around a fixed point, called the center of rotation.
If triangle X′Y′Z′ is a rotation of triangle XYZ, each vertex of triangle X′Y′Z′
must be rotated around the origin for the same angle.
Therefore, we have to check angle measures for each corresponding pair of vertices.
To find out the angle measures, draw rays from the origin through each vertex of triangle XYZ and its corresponding vertex of triangle X′Y′Z′, then measure the angle formed by the rays.
Hence, yes X′Y′Z′ is rotation of XYZ.
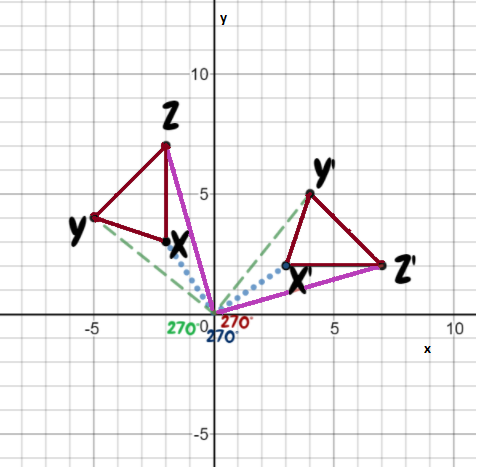
Hence, yes X′Y′Z′ is rotation of XYZ.
Page 313 Exercise 8 Answer
Given:
ΔPQR is rotated 270∘.
To find:
graph and label the coordinates.
We use the concept of rotation to find the graph.
To graph
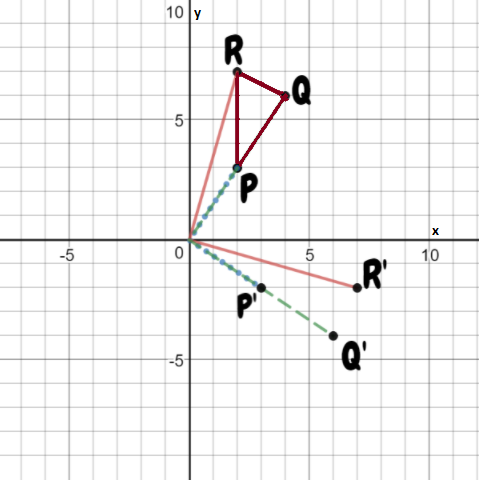
The rotation for 270∘ of triangle 270∘ about origin we have to:
Draw the ray from the origin to vertex P.
Use a protractor to draw a 270∘ angle in counterclockwise direction.
Plot vertex P′, the same distance from the origin as vertex.
We repeat the process to get it for other vertices.
There are some rules for x – and y – coordinates of a point at rotation for , the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before second coordinate:
In another words, when we rotate for 270∘ the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before second coordinate:
(x,y)→(y,−x)
Therefore ,the vertices of triangle,P′Q′R′are:
P(2,3) → P′(3,−2)
Q(4,6) → Q′(6,−4)
R(2,7) → R′(7,−2)
But despite of that, we could also read the coordinates of vertices from the graph.
Therefore, the vertices of rectangle P′Q′R′ are:
P(2,3) → P′(3,−2)
Q(4,6) → Q′(6,−4)
R(2,7) → R′(7,−2)
Page 313 Exercise 9 Answer
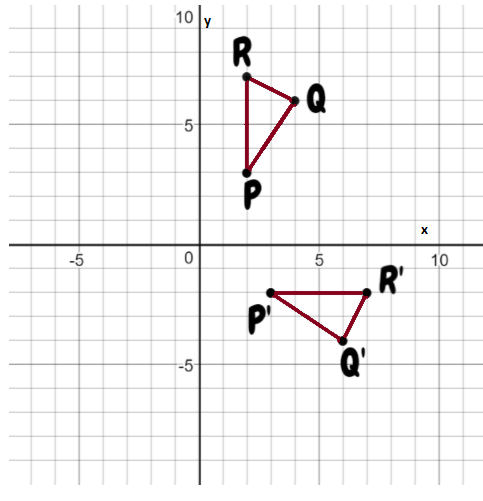
Given:
Graph with two triangles.
To find:
Is P′Q′R′ 270∘ rotation of ΔPQR
A rotation is a transformation that turns a figure around a fixed point, called the center of rotation.
If triangle P′Q′R′ is a 270∘ rotation of triangle PQR, each vertex of triangle PQR must be rotated around the origin for 270∘
Therefore, we have to check angle measures for each corresponding pair of vertices.
To find out the angle measures, draw rays from the origin through each vertex of triangle PQR and its corresponding vertex of triangle P′Q′R′, then measure the angle formed by the rays in the graph given below.
Therefore, we notice that we could also find out, if triangle P′Q′R′ is a 270∘ rotation of triangle PQR, checking the relations between coordinates. Because, there are some rules for x – and y – coordinates of a point at rotation for 270∘.
In another words, when we rotate for 270∘, the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before second coordinate:
(x,y) → (y,−x)
But that is not the case with these vertices:
P (3,3) → P′(−3,3)
Q (7,4) → Q′(−4,7)
R (3,5) → R′(−5,3)
So, we can conclude that triangle P′Q′R′ is not a 270∘ rotation of triangle PQR.
So, we can conclude that triangle P′Q′R′ is not a 270∘ rotation of triangle PQR
Envision Math 8th Grade Congruence And Similarity Topic 6.4 Key Concepts
Page 314 Exercise 10 Answer
To find: Why any rotation can be described by an angle between 20∘ and 360∘
Explanation:
Let’ start at any point of the coordinate system and slowly start to move around it. Once we have moved a quarter of the way around the circle, we will have a 90∘ rotation. Then, when we keep moving, we will pass 180∘ (or halfway) and later 270∘ (or three quarters).
Once we reach the end of the circle, we will have passed a full 360∘ around the circle. In another words, we will be right back where we started, at 0∘.
It means turning around until you point in the same position again.
So, when we rotated some figure 360∘ about the origin, we actually put the figure on the same position. Like we did not even change it.
Therefore, the rotation over 360∘ we can observe like rotation that is reduced by 360∘.
For example, a 420∘ rotation will be:
420∘ − 360∘ = 60∘ rotation
Because in the meantime, we will return to the starting position and from the starting position rotate for another 60∘.
Once we have moved a quarter of the way around the circle, we will have a 90∘ rotation. Then, when we keep moving, we will pass 180∘ (or halfway) and later 270∘ (or three quarters). Once we reach the end of the circle, we will have passed a full 360∘ around the circle.
In another words, we will be right back where we started, at 0∘ about the origin, we actually put the figure on the same position. Like we did not even change it. Hence, any rotation can be described by an angle between 0∘ and 360∘.
Envision Math Grade 8 Volume 1 Student Edition Solutions Chapter 6 Congruence And Similarity Exercise 6.3 Page 314 Exercise 11 Answer
Given:
A graph with a rectangle.
To find:
Rotated KLMN 270∘ about origin.
Use the concepts of rotation to solve the question.
To graph
The rotation for 270∘ of triangle PQR about origin we have to:
Draw the ray from the origin to vertex K.
Use a protractor to draw a 270∘ angle in counterclockwise direction.
Plot vertex K′, the same distance from the origin as vertex K.
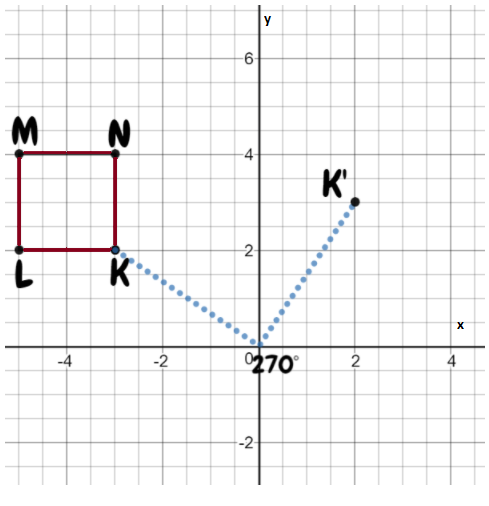
Repeat the procedure for other vertices.
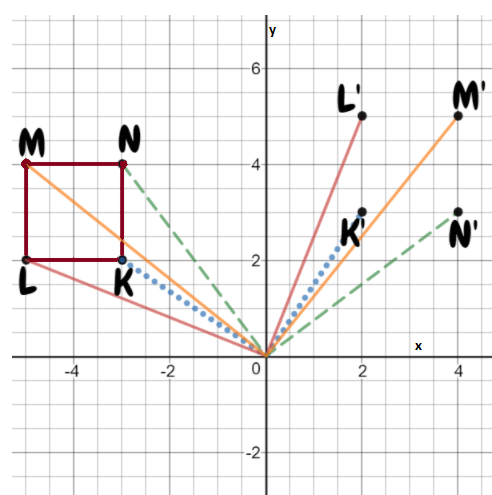
We now join the vertices,
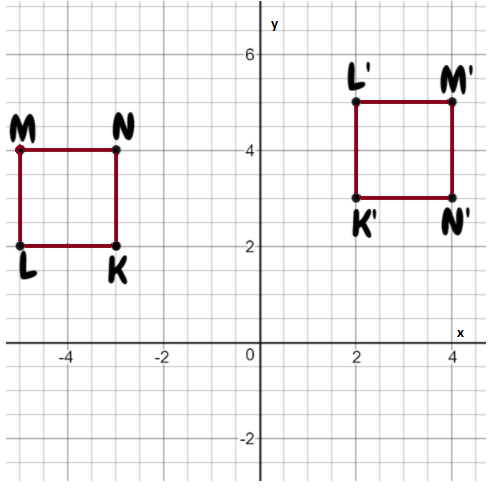
Therefore, the rotated rectangle is
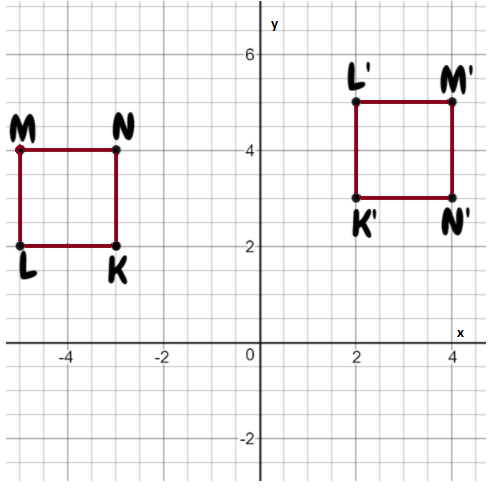
Envision Math Grade 8 Topic 6.4 Similarity Practice
Page 314 Exercise 12 Answer
Given:
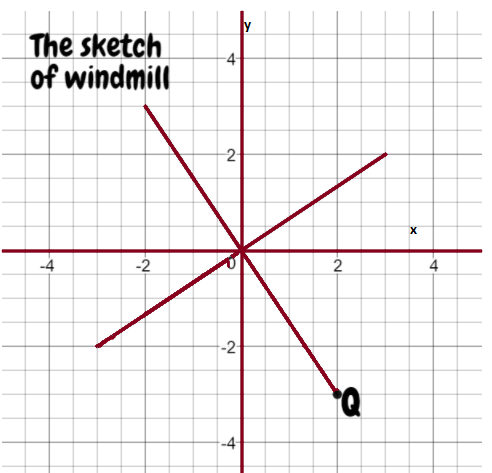
An architect is designing a new windmill with four sails. In her sketch, the sails’ center of rotation is the origin, (0,0), and the tip of one of the sails, point Q, has coordinates (2,−3). She wants to make another sketch that shows the windmill after the sails have rotated 270∘ about their center of rotation
To find: What would be the coordinates of Q′?
We use the concept of rotation.
Let’s drawn first the windmill with four sails. We have information that point Q, the tip of one of the sails, has coordinates (2,−3).
And if we know what kind of form windmills generally have, we can draw the remaining sails.
All four sails are equal in size and intersect at a right angle.
Notice that we can draw these sails so that the default sail we rotated three times for 90∘.
Therefore:
Draw the point Q and the ray from the origin to point Q.
(That will be the sketch of the given sail.)
Use a protractor to draw a 90∘ angle in counterclockwise direction.
Plot point Q1 the tip of other sail, the same distance from the origin as point Q.

We repeat he process,
Now, we have to graph of the sails about origin, their center of rotation to make another sketch that shows the windmill after the rotation of the sails. and the ray. angle in counterclockwise direction. ‘the same distance from the origin as point
the rotation for 270∘ In another words, we have to rotated each tip of the sails for 270∘
So, let’s rotated the first one:
Draw the point Q and a ray from the origin to point Q
(That will be the sketch of the given sail.)
Use a protractor to draw a 270∘ clockwise.
Plot point Q′ the same distance from origin as Q.
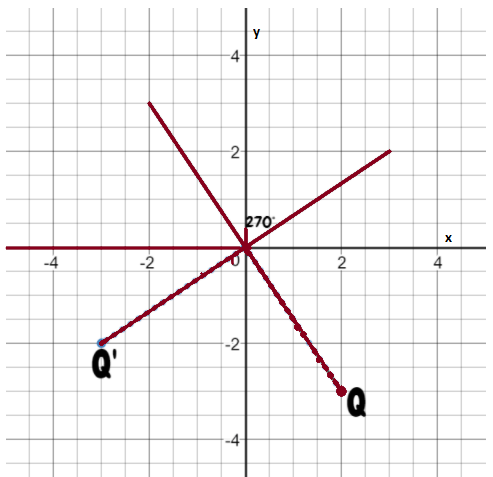
Notice that, the position of that sail, after rotation, is on the position of one of the existing sails before rotation.
This will be the case with other sails after rotation too.
Therefore, we can conclude that the sketch of the windmill will be the same as in the beginning (before rotation).
Only replacing each sail with some existing sail so that the angle between them is 270∘
We still have to specify the coordinates of point – and – coordinates of a point at rotation for, the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before second coordinate: Q′
There are some rules for x and y
In another words, when we rotate for 270∘, the coordinates of the vertices of the resulting image are in reverse order and we have to put minus before second coordinate:
(x,y) → (y,−x)
The coordinates will shift from
Q(2,−3) → Q′(−3,−2)
Envision Math Grade 8 Chapter 6.4 Lesson Overview
Page 314 Exercise 13 Answer
Given:
Rotation about origin maps ΔTRI to ΔT′R′I′.
To find:
Which graph shows an angle you could measure to find the angle of rotation about the origin?
Use the concept of rotation to solve the question.
Looking at the all pictures we can immediately say that graph at B is not graph that show an angle of rotation about origin, because there are two rays from one vertex. Moreover, the one of the ray is not even through the origin.
Then, notice that image at C and D are rays between unmatched vertices. And we measure the angle between corresponding vertices to find out the angle of rotation.
The graph at A shows an angle we could measure to find the angle of rotation about origin.
Result
The graph at A shows an angle we could measure to find the angle of rotation about origin.
The graph at A shows an angle we could measure to find the angle of rotation about origin.
Given:
Rotation about origin maps ΔTRI to ΔT′R′I′
To find:
What is the angle of rotation about axis?
To find the angle of rotation about origin, we have to find the number of degrees that figure rotates.
We have already rays from the origin through point R and point R′.
So, use a protractor to determine the angle formed by rays.

Hence the angle of rotation is 180∘
Since the angle of rotation is 180∘.
Hence the rest of the options are incorrect.
Correct option is (B) 180∘
