Envision Math Accelerated Grade 7 Volume 1 Chapter 2 Real Numbers
Question. Sofia wrote a decimal as a fraction. Nora said that her method and answer was incorrect but Sofia disagrees and says that this is the method she learned. Find the Nora or Sofia Correct.
Given:
Sofia wrote a decimal as a fraction
Nora said that her method and answer was incorrect
But Sofia disagrees and says that this is the method she learned.
To find Is Nora or Sofia correct.
The given Sofia answer is:
0.1211211121112
x = 0.12
100(x) = 100(0.12)
100x = 12.12
99x = 12
x = \(\frac{12}{99}\)
Nora says that her method and answer was incorrect. Actually, Sofia’s method and answer is incorrect as Nora said.
The correct method is:
0.1211211121112
x = 0.12
100(x) = 100(0.12)
100 x = 12.12
Divide both sides by 100
\(\frac{100 x}{100}\)=\(\frac{12.12}{100}\)
x = 0.1212
Finally, we concluded that Sofia’s method and answer is incorrect.
To find Is what is another nonterminating decimal number that cannot be written as a fraction.
0.1211211121112
The given Sofia answer is in the rational fraction.
Any real number that cannot be written in fraction form is an irrational number.
These numbers include non-terminating, non-repeating decimals.
Finally, we concluded that irrational fractions is another nonterminating decimal number that cannot be written as a fraction.
Question. Difference of rational and irrational numbers can be explained.
The difference of rational numbers and irrational numbers can be explained below
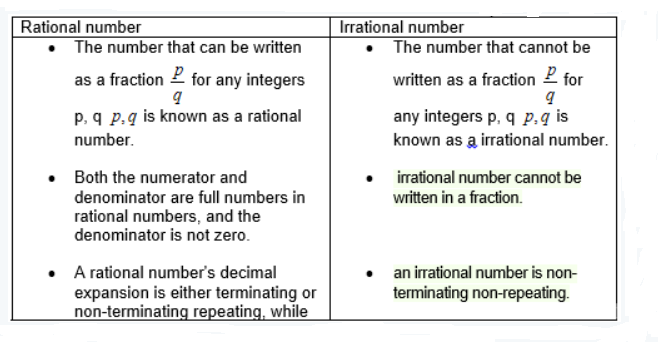
Finally, we concluded an irrational number is different from a rational number.
Question. Classify each number as a rational or irrational number.
Given:
π = 3.565565556..
0.04053661 −17
0.76 3.275
To classify each number as a rational or irrational number
π− Irrational number
0.04053661 − Rational number
0.76767676 − Irrational number
3.565565556.. − Rational number
−17 − Rational number
3.275 − Irrational number
Finally, we classified each number as a rational or irrational number.
Question. Classify each number as a rational or irrational number and explain.
Given:
\(\begin{array}{llll}
\frac{2}{3} & \sqrt{25} & -0.7 \overline{5} & \sqrt{2}
\end{array} 7,548,123\)
To classify each number as a rational or irrational number and explain
Given:
\(\frac{2}{3}\) − Is a rational number as it can be expressed in the form of \(\frac{p}{q}\)
\(\sqrt{25}\)− The number is a perfect square so \(\sqrt{25}\) = 5 is a rational number
\( − 0.7 \overline{5}\) − Is an irrational number, the decimal expansion does not repeat or terminate
\(\sqrt{2}\) − The number is not a perfect square so \(\sqrt{2}\) is an irrational number
7,548,123 − Is an irrational number because the expansion does not repeat or terminate
Finally, we explained and classified each number as a rational or irrational number.
Yes, Jen is correct
The number 4.567 as irrational because it does not repeat.
Finally, we concluded that Jen is correct and the number the number 4.567 as irrational because it does not repeat.
An irrational number differs from a rational number in the sense that it cannot be stated in the style of \(\frac{a}{b}\) , where a and b are both integers and b ≠ 0.
Furthermore, unlike a rational number, which might be recurring or terminating, an irrational number is non-repeating and non-terminating in decimals.
A square root of a not-perfect square is also shown by an irrational number.
Finally, we concluded that an irrational number is different from a rational number as.
The irrational number cannot be written in the form of \(\frac{a}{b}\)
Is non-repeating and non-terminating decimal.
It’s a square root of a not-perfect square.
It is not possible for a number to be both rational and irrational number.
This is because a rational number can be written in the form of \(\frac{p}{q}\) and rational number.
Cannot be written in the form of \(\frac{p}{q}\).
Finally, we concluded that a number cannot be both rational and irrational.
The given decimal is an Irrational number.
This is because the given decimal cannot be written in the form of \(\frac{a}{b}\)
Furthermore, the given decimal is a non-repeating and non-terminating decimal.
Finally, we concluded that the number 65.4349224…. is an irrational number.
Question. Find the number \(\sqrt{2500}\) rational or irrational? Explain.
Given:
Is the number \(\sqrt{2500}\) rational or irrational? Explain.
To find Is the number\(\sqrt{2500}\) rational or irrational? Explain.
The given square root is a rational number.
This is because the given number 2500 is a perfect square
50 × 50 = 2500
⇒ \(\sqrt{2500}\)
= 50
The result of the square root is 50 which can be classified as an integer, Whole number, and natural number.
Finally, we concluded that the number \(\sqrt{2500}\) is a rational number.
Question. Classify each number as rational or irrational.
Given :
\(4. 2\overline{7} \) ,0.375,0.232342345…,\(\sqrt{62},-\frac{13}{1}\)
To Classify each number as rational or irrational.
An irrational number is different from a rational number in terms that the irrational number.
It cannot be written in the form of \(\frac{a}{b}\), Where a and b are both integers and b≠0.
A rational number is recurring or terminating, an irrational number is non-repeating and non-terminating in decimals.
A square root of a not-perfect square is also shown by an irrational number.
According to that, The table for the given numbers as rational and irrational numbers.
⇒ \(\begin{array}{|l|l|}
\hline \text { Rational } & \text { Irrational } \\
\hline 4.2 \overline{7} & 0.232342345 \ldots \\
\hline 0.375 & \sqrt{62} \\
\hline-\frac{13}{1} & \\
\hline
\end{array}\)
Finally, we concluded that The table for the given numbers as rational and irrational numbers.
⇒ \(\begin{array}{|l|l|}
\hline \text { Rational } & \text { Irrational } \\
\hline 4.2 \overline{7} & 0.232342345 \ldots \\
\hline 0.375 & \sqrt{62} \\
\hline-\frac{13}{1} & \\
\hline
\end{array}\)
Question. Find 5.787787778…. a rational number? Explain.
Given:
Is 5.787787778…. a rational number? Explain
To find Is 5.787787778….a rational number? Explain.
The given decimal 5.787787778…. is an Irrational number.
This is because the given decimal cannot be written in the form of \(\frac{a}{b}\).
Furthermore, the given decimal is a non-repeating and non-terminating decimal.
Finally, we concluded that 5.787787778…. …is an irrational number.
Question. Find the number \(\sqrt{42}\) rational or irrational? Explain.
Given:
Is the number \(\sqrt{42}\) rational or irrational? Explain.
To find Is the number \(\sqrt{42}\) rational or irrational? Explain.
The given \(\sqrt{42}\) is an irrational number.
This is because the given number 42 is not a perfect square.
Therefore, the result of the given square root will be a non-terminating decimal.
Finally, we concluded that the number \(\sqrt{42}\) is irrational.
Question. Find which card shows irrational numbers.
Given:
- 10
- \(\frac{6}{5}\)
- π
- \(\frac{11}{4}\)
- 8.25635…
- \(6. \overline{31}\)
- -7
To find Which card shows irrational numbers?
From the given cards, the irrational numbers are π and 8.25635…
These two cards are said to be irrational because they cannot be written in the form of \(\frac{a}{b}\) and are non-repeating and non-terminating decimals.
Finally, we concluded that C)π and D) 8.25635…. are the irrational numbers
Given:
⇒ \(7. \overline{27}, \frac{5}{9}, \sqrt{15}, \sqrt{196}\)
To Circle the irrational number in the list.
An irrational number is different from a rational number in terms that the irrational number cannot be written in the form of \(\frac{a}{b}\) , Where a and b are both integers and b ≠ 0.
A rational number is recurring or terminating, an irrational number is non-repeating and non-terminating in decimals.
A square root of a not-perfect square is also shown by an irrational number.
According to that, From the given list the irrational number is \(\sqrt{15}\) .
15 is not a perfect square.
Therefore, the result of the square root of 15 would be a non-terminating and non-repeating decimal.
Which is an irrational number.
Finally, we concluded that \(\sqrt{15}\) is the irrational number in the given list.
⇒ \(\sqrt{76+n}\) and.\(\sqrt{2 n+26}\)
Question. Find which numbers are rational.
Given:
5.737737773…,26, \(\sqrt{45}\) \(-\frac{3}{2}\) ,0,9
To find which numbers are rational.
Given:
5.737737773…,26, \(\sqrt{45}\) ,\(-\frac{3}{2}\) ,0,9 26, \(-\frac{3}{2}\) ,0,9.
These are rational numbers because a rational number can be written in the form of \(\frac{a}{b}\).
Finally we concluded 26,\(-\frac{3}{2}\) ,0,9 is rational numbers.
Question. Find the which numbers are irrational.
Given:
5.737737773…,26, \(\sqrt{45}\) ,\(-\frac{3}{2}\) ,0,9
To find which numbers are irrational.
Given:
5.737737773…,26,\(\sqrt{45}\) ,\(-\frac{3}{2}\),0,9 5.737737773…, \(\sqrt{45}\).
These are irrational numbers because irrational numbers cannot be written in the form of \(\frac{p}{q}\).
Finally we concluded that 5.737737773…, \(\sqrt{45}\) are irrational numbers.
Deena says that 9.565565556… is a rational number, no it is wrong because 9.565565556… is an irrational number and it has a repeating value.
Finally, we concluded that Deena’s statement is wrong and 9.565565556… is an irrational number.
The given decimal form \(\frac{13}{3}\) is a rational number because it can be expressed in the form of.
⇒ \(\frac{p}{q}\) and it is the fraction of two integers, where the numerator is 13 and the denominator is 3.
Finally, we concluded that the decimal form of \(\frac{13}{3}\) a rational number.
The given rational numbers are 2.888… and 2.999…
The irrational number that is between the two rational numbers is 2.889888… and 2.998999…
Finally, we concluded that the irrational number between two rational numbers is 2.889888… and 2.998999…
Question. Find the smallest value of n that will make each number rational given expression is \(\sqrt{76+n}\) and.\(\sqrt{2 n+26}\)
Given:
The given expression is \(\sqrt{76+n}\) and.\(\sqrt{2 n+26}\)
To find the smallest value of \[n\]that will make each number rational.
Given:
⇒ \(\sqrt{76+n}\) and .\(\sqrt{2 n+26}\)
Substitute n = 5 in the above equation
⇒ \(\sqrt{76+n}\)
⇒ \( = \sqrt{81}\)
⇒ \( = \frac{9}{\sqrt{2(5)+26}}\)
⇒ \( = \sqrt{36}\)
=6
Finally, we concluded the smallest value of n is n = 5.
Question. Differentiate the rational and irrational \(\frac{8}{5}\),π,0,\( = \sqrt{1}\),4.46466…,−6,\(=\sqrt{2}\)
Given:
⇒ \(\frac{8}{5}\),π,0,\( = \sqrt{1}\),4.46466…,−6,\(=\sqrt{2}\)
To differentiate the rational and irrational
⇒ \(\begin{array}{|l|l|}
\hline \text { rational } & \text { irrational } \\
\hline \frac{8}{5} & \pi \\
\hline 0 & \sqrt{1} \\
\hline-6 & 4.46466 \ldots, \\
\hline & \sqrt{2} \\
\hline
\end{array}\)
Finally we concluded the rational are \(\frac{8}{5}\) ,0,6 and irrational are π,\(=\sqrt{1}\) ,4.46466 \(=\sqrt{2}\)