Envision Algebra 1 Assessment Readiness Workbook Student Edition Chapter 4

Envision Algebra 1 Assessment Readiness Workbook Chapter 4

Page 52 Exercise 1 Answer

In the question, five numbers are given as,

1. \(\frac{1}{7}\)

2. √3

3. √15

4. √25

5. \(\frac{2}{3}\)

It is required to find which of the given numbers are irrational.

To find this, consider each number and check whether it can be reduced to any ratio between an integer p and a natural number q. If it is possible, the number is not irrational.

Consider the number \(\frac{1}{7}\) and check whether it can be reduced to any ratio between an integer p and a natural number q.

The number \(\frac{1}{7}\) is in the form \(\frac{p}{q}\).

So, it is rational.

\(\frac{1}{7}\) is rational.

Consider the number √3 and check whether it can be reduced to any ratio between an integer p and a natural number q.

√3 cannot be reduced to any ratio between an integer p and a natural number q.

So, √3 is irrational.

Consider the number √15 and check whether it can be reduced to any ratio between an integer p and a natural number q.

√15 = √3 × √5, both terms are irrational since they cannot be reduced to any ratio between an integer p and a natural number q.

So,√15 is irrational.

Consider the number √25 and check whether it can be reduced to any ratio between an integer p and a natural number q.

√25 = 5

5 can be written in \(\frac{p}{q}\) form as \(\frac{5}{1}\).

So, √25 is rational.

Consider the number \(\frac{2}{3}\) and check whether it can be reduced to any ratio between an integer p and a natural number q.

The number \(\frac{2}{3}\) is in the form \(\frac{2}{3}\).

So, it is rational.

\(\frac{2}{3}\) is rational.

By considering each number and checking whether it can be reduced to any ratio between an integer p and a natural number q, it is found that numbers (B)√3 and  (C) √15 are irrational.

Page 52 Exercise 2 Answer

In the question, \(\frac{(3)^{-4}(7)^{-2}}{(3)^2(7)^{-5}}\) is given. And four forms are as

1. \(\frac{7^7}{3^6}\)

2. \(\frac{7^3}{3^6}\)

3. \(\frac{3^2}{7^3}\)

4. \(\frac{3^6}{7^7}\)

It is required to rewrite \(\frac{(3)^{-4}(7)^{-2}}{(3)^2(7)^{-5}}\) using positive exponents.

Given \(\frac{(3)^{-4}(7)^{-2}}{(3)^2(7)^{-5}}\)

Exponential formula \(a-b=\frac{1}{a^b}\)

Apply the exponential formula \(a-b=\frac{1}{a^6} \text { to } \frac{(3)^{-4}(7)^{-2}}{(3)^2(7)^{-5}}\)

⇒ \(\frac{(3)^{-4}(7)^{-2}}{(3)^2(7)^{-5}}=\frac{(7)^5}{(7)^2(3)^2(3)^4}\)

Apply the exponential formula \(\frac{a^m}{a^n}=a^{m-n} \text { and } a^m a^n=a^{m+n} \text { to } \frac{(7)^5}{(7)^5(3)^2(3)^4}\)

⇒ \(\begin{aligned}
\frac{(7)^5}{(7)^2(3)^2(3)^4} & =\frac{(7)^{5-2}}{(3)^{2+4}} \\
& =\frac{(7)^3}{(3)^6}
\end{aligned}\)

Using exponential equations \(\frac{1}{a^b}\), \(\frac{a^m}{a^n}\) = am-n and a^maⁿ= a^m+n,

⇒ \(\frac{(3)^{-4}(7)^{-2}}{(3)^2(7)^{-5}}\) can be rewritten as \(\frac{(7)^{3}}{(3)^{6}}\)

Page 52 Exercise 3 Answer

In the question, an equation is given as ∣2x−3∣ − 4 = 3 and four solutions are given as

1. −3

2. −2

3. 3

4. 5

5. 6

It is required to find the solution of the given equation.

To find this, first find the modulus value of ∣2x−3∣. Then two values are obtained. Take these two values as two cases and find the value of x from both cases.

Given |2x-3|-4=3
|2x-3|-4=3
|2x-3|=3+4
|2x-3|=7

So, talking modulus 2x-3=±7

Take the value 2x-3 as 7 and find the value of x

2x-3=7
2x=7+3
2x=10

Divide 2 on both sides

⇒ \(\frac{2 x}{2}=\frac{10}{2}\)

Take the value 2x-3 as-7 find the value of x

2x-3=-7
2x=-7+3
2x=-4

Divide 2 on both sides

\(\frac{2 x}{2}=\frac{-4}{2}\)

x=-2

By using the equation ∣x∣ = ±x, the two solutions obtained are

(B) x = −2 and

(D)x = 5

Page 52 Exercise 4 Answer

In the question, it is given that a line passes through two points (4,−1) and (−4,3).

Also, four equations are given as

1. y = \(\frac{1}{2} x-1\)

2. y = 2x – 1

3. y = \(\frac{-1}{2} x-1\)

4. y = -2x -1

It is required to find which equation represents a line perpendicular to a line that passes through (4,−1) and (−4,3).

To solve this, first find the slope of the line passes through (4,−1) and (−4,3) using the equation m = \(\frac{y_2-y_1}{x_2-x_1}\) and then use this slope to find the slope of the line perpendicular to the line passes through (4, -1) and (-4, 3) using the equation m₁ = \(\frac{-1}{m}\).

The slope of the line passes through (4,-1) and (4,3) using the equation.

m= \(\begin{aligned}
& m=\frac{y_2-y_1}{x_2-x_1} \\
& m=\frac{3-(-1)}{-4(-4)} \\
& m=\frac{3+1}{-4-(4)}
\end{aligned}\)

Reducing \(\frac{4}{-8}=\frac{-1}{2}\)

The slope of line passing through (4,-1) and (-4,3) is \(\frac{-1}{2}[\)

Slope of the line passing through (4,−1) and (−4,3) is m = \(\frac{-1}{2}\).

Find the slope m₁ of the line perpendicular to the line having slope m using the equation m₁= \(\frac{-1}{m}\).

m₁ = \(-\frac{1}{\left(-\frac{1}{2}\right)}\)

= 2

Find the equation of the line with slope 2 from the given options.

Since the slope of the line is 2, the equation satisfying is y = 2x − 1.

The slope of the line passing through(4,−1) and (−4,3) using the equation m = \(\frac{y_2-y_1}{x_2-x_1}\) is found as m = \(\frac{-1}{2}\).

The slope of the line perpendicular to the line having slope m using the equation m₁ = \(-\frac{1}{\left(-\frac{1}{2}\right)}\), is found as m₁ = 2.

Since the slope of the line is 2, the equation satisfying is (B) y = 2x − 1.

Page 52 Exercise 6 Answer

In the question, an expression (6x⁴y⁶)² is given. Also, four options are given as

1. 6x⁶y⁸

2. 12x⁸y¹²

3. 36x⁸y¹²

4. 36x⁶y⁸

It is required to simplify (6x⁴y⁶)² and to find which of the four options is right.

To solve this, use the equation (abc)ⁿ = aⁿbⁿcⁿ to the given expression and simplify it using mathematical operations.

Given (6x⁴y⁶)²

Apply the equation (abc)ⁿ = aⁿbⁿcⁿ to (6x⁴y⁶)²

(6x⁴y⁶)² =(6)².(x⁴)².(y⁶)²
(6x⁴y⁶)²=36x⁸y¹²

So, (6x⁴y⁶)²=36x⁸y¹²

Using the exponential equations (abc)ⁿ = aⁿbⁿcⁿ and

(ab)c= abc, (6x⁴y⁶)² can be simplified as 36x⁸y¹².

So (C) 36x⁸y¹² is the correct option.

Page 53 Exercise 7 Answer

In the question, a function is given as f(x) = ∣−x+7∣.

Also, four piecewise functions are given as

1. g(x) = \(\left\{\begin{array}{l}
-x+7, \text { if } x \leq-7 \\
x-7 \text {,if } x>-7
\end{array}\right.\)

1. g(x) = \(\left\{\begin{array}{l}
-x+7 \text {, if } x \leq 7 \\
x-7, \text { if } x>7
\end{array}\right.\)

1. g(x) = \(\left\{\begin{array}{l}
-x+7 \text {, if } x \geq 0 \\
x-7 \text {, if } x<0
\end{array}\right.\)

1. g(x) = \(\left\{\begin{array}{l}
-x+7 \text {, if } x \geq 7 \\
x-7 \text {, if } x<7
\end{array}\right.\)

It is required to identify the piecewise function that has the same graph as the function f(x) = ∣−x+7∣.

To solve this, the function will break when the term in the modulus becomes zero.

Find the value of x when the modulus becomes 0 and find the function value to the left and right of the x value.

Find the value of x when the modulus becomes 0.

Given f(x)=|-x+7|

Find the value of when the models become o

=-x+7=0
x=7

so, the function breaks art x=7
find the function value when x<7.

​if x<7
f(x)=-(-x+7
f(x)=x-7
i.e., f(x)=x-7,if x<7

Find the function value when x ≥ 7.

f(x) = −x+7.

i.e., f(x) = −x+7,if x ≥ 7.

The piecewise function having the same graph as f(x) = ∣−x+7∣ is

1. g(x) = \(\left\{\begin{array}{l}
-x+7 \text {, if } x \geq 7 \\
x-7 \text {, if } x<7
\end{array}\right.\)

Page 53 Exercise 8 Answer

In the question, a function f(x) = 2^x is given.

It is required to state which type of function is f(x) = 2^x

The general equation of the exponential function is f(x) = a^x

Comparing the given function with the general equation of the exponential function f(x) = a^x, it is found that

f(x) = 2^x is an exponential function with the value of a 2.

By Comparing the given function with the general equation of the exponential function f(x) = a^x, it is found that f(x) = 2^x is an exponential function with the value of a is 2.

Page 53 Exercise 9 Answer

In question four scores of a student are given as 89, 98, 93, and 97 and the average of five scores is given as 94.

It is required to find what score must the student earn on the fifth test to have an average score of 94.

To find this, let the score of the fifth subject be x. Substitute this in the equation of average score and find x.

Let x be the fifth score and substitute it in the equation of the average score.

​Average score \(=\frac{\text { total score }}{\text { number of Scores }}\)

⇒ \(94=\frac{89+98+93+97+x}{5}\)

⇒ \(94=\frac{377+x}{5}\)

94×5=377+x

Simplify the above equation and find x

470=377+x
x=470-377
x=93

So, the fifth score is 93.

Letting the fifth score as x and substituting it in the equation of average score, the fifth score is found as 93 to get an average of 94.

Page 53 Exercise 10 Answer

In the question, a graph is given.

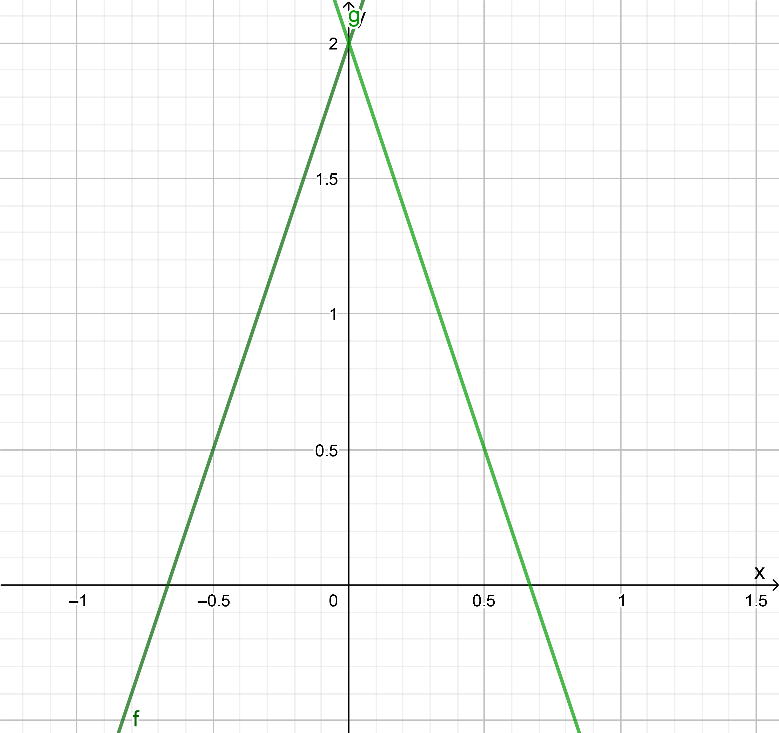
Four equations are given as

y = −∣3x∣ + 2

y = ∣3x+2∣

y = −3∣x+2∣

y = ∣3x∣ + 2

It is required to find the correct equation of the graph.

To find this, first find the coordinates from the given graph and find the equation of the line using the two-point form of a line (y – y₁) = \(\left(\frac{y_2-y_1}{x_2-x_1}\right)\left(x-x_1\right)\).

Find the coordinates from the given graph.
The coordinates are (0,2), (\(\frac{2}{3}\), 0) and (\(\frac{-2}{3}\), 0).

Find the equation of the line passing through (0,2) and (\(\frac{2}{3}\), 0) using two-point form of the line.

⇒ \(\left(y-y_1\right)=\left(\frac{y_2-y_1}{x_2-x_1}\right)\left(x-x_1\right)\)

⇒ \((y-2)=\left(\frac{0-2}{\frac{2}{3}-0}\right)(x-0)\)

⇒ \((y-2)=\frac{-2}{\frac{2}{3}} x\)

(y-2)=-3x

Find the equation of the line passing through (0,2)(0,2) and (\(\frac{-2}{3}\), 0) using the two-point form of the line.

⇒ \(\left(y-y_1\right)=\left(\frac{y_2-y_1}{x_2-x_1}\right)\left(x-x_1\right)\)

⇒ \((y-2)=\left(\frac{0-2}{\frac{-2}{3}-0}\right)(x-0)\)

⇒ \((y-2)=\frac{-2}{\frac{-2}{3}} x\)

(y-2)=3x

Write the two equations in the modulus form.

The equations are (y−2) = −3x and

(y−2) = 3x

−3x ≤ y−2 ≤ 3x

So, the resultant equation can be written as y = ∣3x∣+2.

By finding the coordinates from the given graph and applying a two-point form of the line (y – y₁) = \(\left(\frac{y_2-y_1}{x_2-x_1}\right)\left(x-x_1\right)\), the equation of the graph is found as y = |3x|+2.

So, the correct equation is (D) y = |3x|+2.

Page 53 Exercise 11 Answer

In the question, a scatter point graph is given with four options.

1. y = x + 2

2. y = −x + 2

3. y = 2x + 2

4. y = −2x + 2

 

It is required to find the best estimation of the equation of the fit line from given options.

To solve the question, draw a line that approximately divides the scatter points in half. Now find the equation of this line and compare it with the given options.

Draw a best-fit line of the scatter points.

The line should be drawn in this way so that it approximately divides the number of points in half on both sides of the line.

Find the equation of the line.

Extend the line so that it touches the x-axis. Now two points on the line are (0,2) and (−1,0).

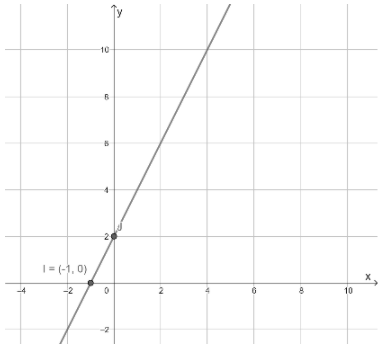
The slope of the line is

\(\frac{0-2}{-1-0}=\frac{-2}{-1}\)

= 2

And the y-intercept is 2.

Thus, the equation of the line is y = 2x + 2.

Therefore, option C) y = 2x + 2 is the best-estimated equation for the given scatter points which was solved using the general equation of line and slope of the line.

Page 43 Exercise 12 Answer

In the question, six numbers \(\frac{-1}{4}\), -1, √3. √-8, \(\frac{1}{2}\), 3 are given.

It is required to arrange the numbers from least to greatest.

To solve the question, convert all numbers in decimal format. Then arrange the number from least to greatest.

Arrange the numbers from least to greatest.

Numbers are \(\frac{-1}{4}\), -1, √3, √-8, \(\frac{1}{2}\), 3.

Using a calculator, write numbers as decimals, -0.25, -1, 1.732, -2.828, 0.5, 3.

Now arrange in ascending order −2.828, −1, −0.25, 0.5, 1.732,3

Thus, the numbers order from least to greatest are −√8, -1, \(\frac{-1}{4}\), \(\frac{1}{2}\), √3, 3.

Thus, the numbers order from least to greatest are −√8, -1, \(\frac{-1}{4}\), \(\frac{1}{2}\), √3, 3 which was solved by first converting numbers into decimal and then arranging.

Page 54 Exercise 13 Answer

In the question, a dot plot of data is given.

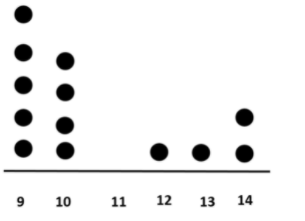
It is required to find the mean and median of the data shown in the above figure to the nearest hundredth.

To solve the question, make a table of given data. Then find the mean and median using suitable formulas and mathematical operations.

Make a table of the given data.

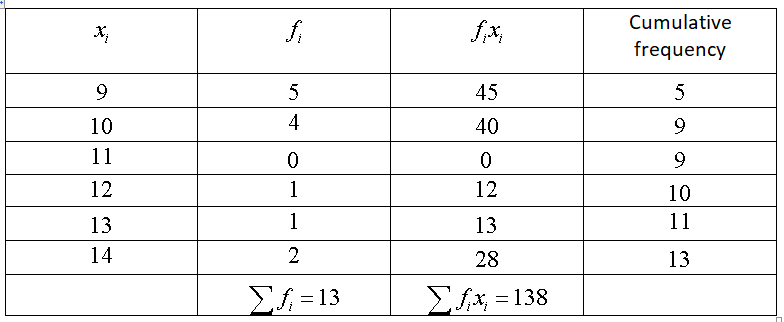
Find the mean of the data.

Mean \(\bar{x}=\frac{\sum f_i x_i}{\sum f_i}\)

⇒ \(\bar{x}=\frac{138}{13}\)

⇒\(\bar{x}=11.5\)

Thus, the mean of the data nearest to the hundredth is 11.50.

Find the median of the data.

Since the sum of the frequencies n is 13, which is an odd number.

Then the median is at the position

⇒ \(\left(\frac{13+1}{2}\right)^{t h}=7^{t h}\).

The number in the 7^th position is 10.

Therefore, the median nearest to the hundredth is 10.00.

Therefore, the mean of the data nearest to the hundredth is $11.50$ and the median nearest to the hundredth is $10.00$. They are different as the mean gives the average value of data and the median gives the middle number of data which were solved using formulas of mean and median.

Page 54 Exercise 14 Answer

In the question, the area of the rectangle is represented by a quadratic equation, 12x² + x − 6, and the width of the rectangle is represented as 3x−2.

It is required to find the width of the rectangle.

To solve the question, find the roots of the quadratic equations using quadratic formulas. Then write the equation as a product of its factors using its roots.

Find the roots of the quadratic equation.

Given equation is 12x²-x+6

⇒ \(x=\frac{-1 \pm \sqrt{1^2-4(12)(6)}}{2(12)}\)

⇒ \(\begin{aligned}
& x=\frac{-1 \pm \sqrt{1+288}}{24} \\
& x=-1 \pm 17
\end{aligned}\)

Solving further to calculate roots

⇒ \(\begin{aligned}
& x=\frac{-1+17}{24} \\
& x=\frac{16}{24} \\
& x=\frac{2}{3}
\end{aligned}\)

Solving further to calculate another root

⇒ \(\begin{aligned}
& x=\frac{-1-17}{24} \\
& x=\frac{-18}{24} \\
& x=\frac{-3}{4}
\end{aligned}\)

Solve further take x= \(\frac{2}{3}\)

Multiply by 3 and then subtract by 2

3x-2=0

⇒ \(x=\frac{-3}{4}\)

Multiply by 4 and then add by 3 4x+3=0.

Find the length of the square.

Now, the quadratic equation can be factored as

12x² + x − 6 = (4x+3)(3x−2)

On comparing with the formula,

A = l × b

So, the length is 4x+3 units.

Thus, the length of the rectangle is 4x+3 units which was solved using the quadratic formula and then finding factors of the equation.

Page 54 Exercise 15 Answer

In the question, a linear inequality, 2x+4y < 4, is given.

It is required to graph the inequality.

To solve the question, rearrange the equation so that on the left side there is a y variable, and everything else on the right. Then plot the inequality as a simple linear equation. Then shade the graph above the line for a ‘greater than’ inequality and below the line for a ‘less than’ inequality.

Rearrange the equation.

It should be done so that on the left side there is a y variable, and everything else on the right.

Given inequality is 2x+4y<4

Subtract 2x from both sides

4y<4-2x

Divide by 4 on both sides

⇒ \(\begin{aligned}
\frac{4 y}{4} & <\frac{4}{4}-\frac{2 x}{4} \\
y & <1-\frac{1}{2} x
\end{aligned}\)

A simple linear equation of the above inequality can be written as y = 1 – \(\frac{1}{2} x\).

Plot the equation on the graph.

y = 1 – \(\frac{1}{2} x\)

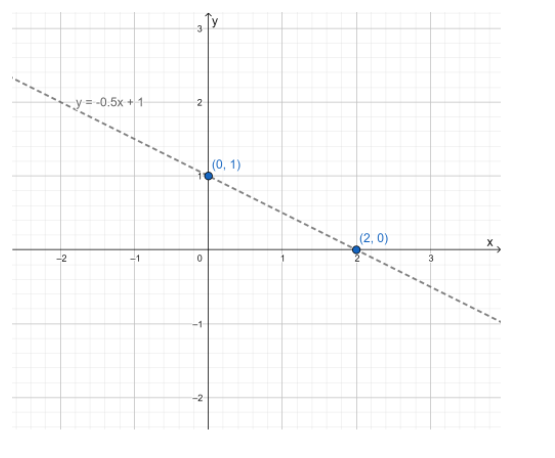
The line in the above graph is dashed as the inequality 4y < 4−2x contains < not ≤.

Solve further.

As the inequality, 4y < 4 − 2x, contains less than sign, the shaded area is

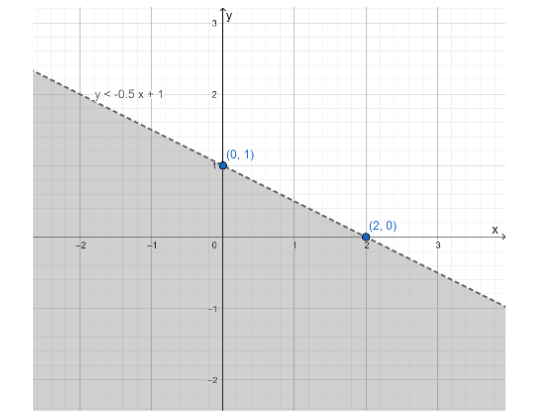
Thus, the graph of the linear inequality 2x+4y < 4 is sketched which was solved by first plotting a simple equation and then shading the region.

 

Page 54 Exercise 16 Answer

In the question, a function f(x) = x² − 4x + 5 is given.

It is required to graph the function.

To solve the question, convert the given equation into a general equation of the parabola.

Convert the given equation into a general equation of the parabola.

Given function can be written as

y = x² − 4x + 5

Now add and subtract the square of half of the coefficient of x in the equation.
​
Given function f(x) = x²-4x+5
Given function can be written as

y=x²-4x+5+2²-2²

y=x²-4x+4+5-4
y=x²-4x+4+5-4
y=x²-4x+4+1

Solve further to get it in general form

y= x²-2(2) x+2²+1
y=(x-2)²+1

Find the value of the vertex of the parabola.

y = (x−2)² + 1

By comparing it with the standard equation, the vertex (h,k) of the parabola is (2,1).

As a > 1, then the graph opens up.

Now plot the graph.

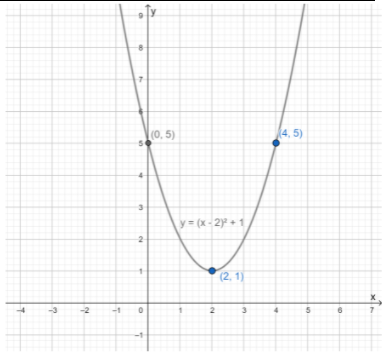
Therefore, a graph of the function f(x) = x² − 4x + 5 is sketched which was solved using the standard equation of parabola.

Page 55 Exercise 17 Answer

In the question, it is given that a ball is thrown directly upwards from a height of 2 feet and velocity is 16 feet per second, and equation h = −16t² + 16t + 2.

It is required to find the time it takes for a ball to reach its maximum height and maximum height of the ball.

To solve the question, find the slope of the given equation and equate the obtained equation to zero to find the time t. Then substitute the obtained value of time in the given equation to find the maximum height.

Find the time that the ball takes to reach maximum height.

Given equation is h=-16t2+16t+2

⇒ \(\frac{d h}{d t}=-32 t+16\)

At maximum height \(\frac{d h}{d t}=0\)

-32t+16=0

Solve further
Subtract 16 from both sides
-32t=-16

Solve further
Subtract 16 from both sides

-32t=-16

Divide both sides by -32

⇒ \(\begin{gathered}
\frac{-32 t}{-32}=\frac{-16}{-32} \\
t=\frac{1}{2}
\end{gathered}\)

Thus, the time required to go to maximum height is 0.5 seconds.

Put t=0.5 in the equation

h=-16t2+16t+2
h=-16(0.5)2+16(0.5)+2
h=-16(0.25)+8+2
h=-4+10

Solve further

h=-4+10
h=6

Thus, the time required to go to maximum height is 0.5 second

The initial height is 2 feet.

So, the maximum height is

6 + 2 = 8 feet.

A. The ball takes 0.5 sec to reach its maximum height.

B. Maximum height reached is 8 feet which was solved using the concept of slope and first derivative.

Page 55 Exercise 18 Answer

In the question, a table is given.

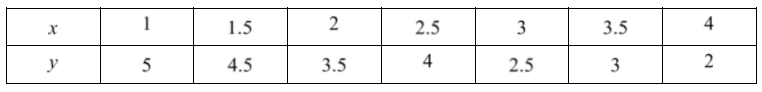
It is required to plot the data and find the estimation of the equation of the best-fit line.

To solve the question, plot the scatter points on a graph. Then draw a line that approximately divides the scatter points in half. Now find the equation of this line.

Plot the scatter points on a graph using a given table.

 

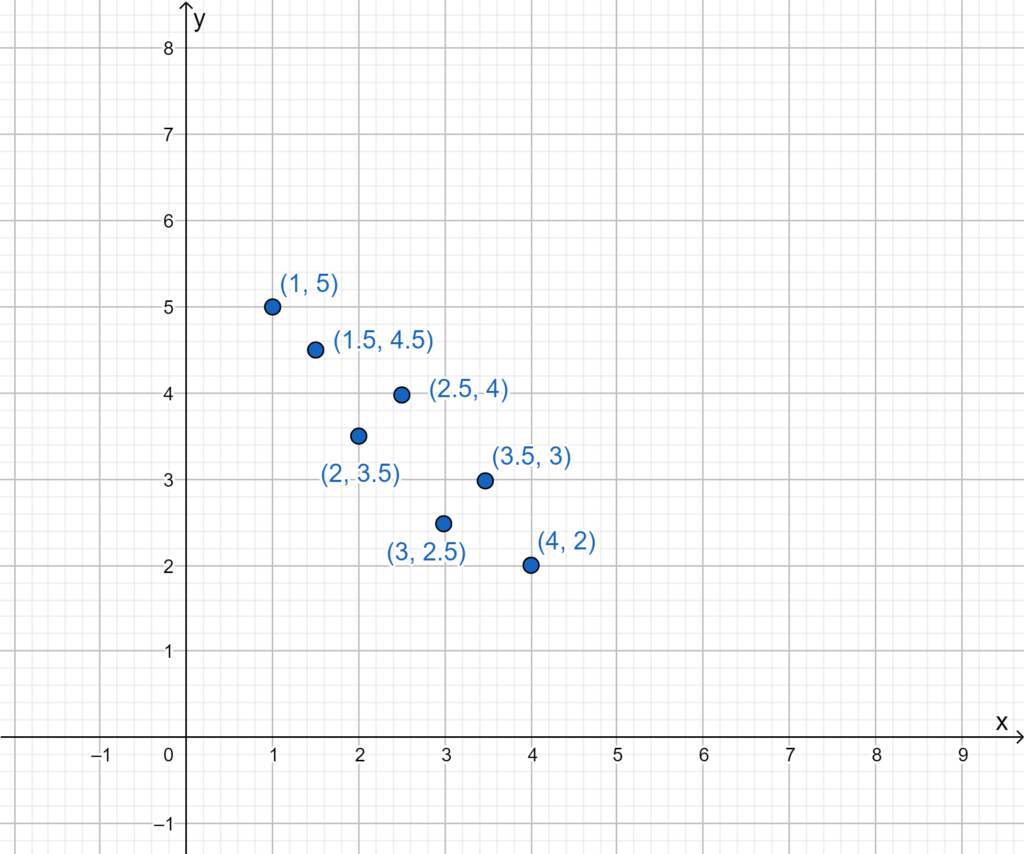
Draw a best-fit line of the scatter points.

The line should be drawn in this way so that it approximately divides the number of points in half on both sides of the line.

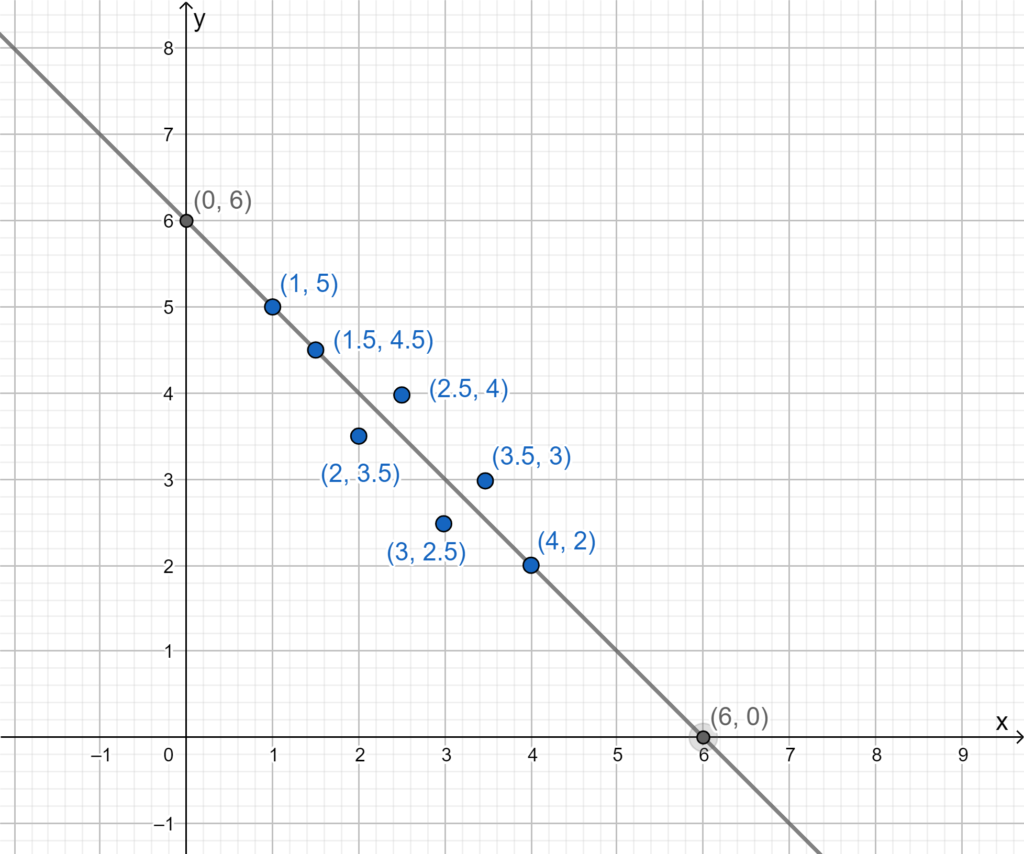
Find the equation of the line.

Extend the line so that it touches the x-axis. Now two points on the line are (0,6) and (6,0).

Slope of the line is

\(\frac{0-6}{6-0}=\frac{-6}{6}\)

= -1

And the y-intercept is 6.

Thus, the equation of the line is y = −x + 6.

Therefore, the graph of scatter points is plotted and y = -x + 6 is the best-estimated equation for the given scatter points which was solved using a general equation of the line and slope of the line.

Page 56 Exercise 19 Answer

In the question,  it is given that the length of the hypotenuse of the right triangle is 25cm and the measure of one leg is 20 cm.

It is required to find the length of the other leg.

To solve the question, put the value of the lengths of the leg and hypotenuse in the formula of Pythagoras’ theorem. Then solve using appropriate mathematical operations.

Find the length of the leg of the right triangle.

Put b as 20 and c as 25 in the Pythagoras theorem formula.

​a²+b²=c²
put b=20 and c=25

a²+20²=25²
a²+400=625

Subtract 400 from both sides

a²+400-400=625-400

a²=225

Take square root on both sides

⇒ \(\begin{aligned}
\sqrt{a^2} & =\sqrt{225} \\
a & =\sqrt{25^2} \\
a & = \pm 15
\end{aligned}\)

But measuring length cannot be negative. Thus, the measure of another leg of the right triangle is 15 units.

Thus, the measure of another leg of the right triangle is 15 units which was solved using Pythagoras theorem.

Page 56 Exercise 20 Answer

In the question, a quadratic equation 5x² − 18x + 9 = −3 is given.

1. 0.37

2. 0.6

3. 0.88

4. 2.72

5. 3

6. 3.23

It is required to choose the correct solutions of the equation from the given options.

To solve the question, convert the given quadratic equation into the form ax² + bx + c = 0. Then put values of a, b, and c in the quadratic formula to find the solutions.

Convert quadratic equations into general form.

Given 5×2-18x+9=-3
Add 3 on both sides
5×2-18x+9+3=-3+3
5×2-18x+12=0

Compared with the general equation

a=5,b=-18,c=12

⇒ \(x=\frac{-(-18) \pm \sqrt{(-18)^2-4(5)(12)}}{2(5)}\)

⇒ \(\begin{aligned}
& x=\frac{18 \pm \sqrt{324-240}}{10} \\
& x=\frac{18 \pm \sqrt{84}}{10}
\end{aligned}\)

use to calculate to evaluate

⇒ \(x=\frac{18 \pm 9.16}{10}\)

Solve further

⇒ \(\begin{aligned}
& x=\frac{18+9.16}{10} \\
& x=2.716 \\
& x=\frac{18-9 \cdot 16}{10} \\
& x=0.884
\end{aligned}\)

Thus, the solutions of the equation to the nearest hundredth are 2.72 and 0.88.

Thus, solutions of the equation to the nearest hundredth are C) 2.72 and D) 0.88 which were solved using a quadratic formula.

Page 56 Exercise 21 Answer

In the question it is given two equations namely, y = 3x + 3 and y = 3x.

It is required to conclude that how the graph of the equation y = 3x + 3 can be described as the transformation of the graph of equation y = 3x. To solve the question start with writing the given equations as f(x) and g(x) respectively.

Then, write the value of g(x) in terms of f(x) and compare it with the formula of vertical shift to get the required answer.

Write the first equation as f(x) and g(x) as the second equation.
​
​f(x) = 3x + 3

g(x) = 3x

Write f(x) in terms of g(x).

​f(x) = 3x + 3

f(x) = g(x) + 3
​
Now, rewrite the expression and find the value of g(x) in terms of f(x) by subtracting 3 from both sides of the equation.
​
​g(x) + 3 − 3 = f(x) − 3

g(x) = f(x) − 3

Use the formula that vertical shift depends upon the value of k.

g(x) = f(x) + k, which means the graph is shifted k units upwards.

g(x) = f(x) − k, which means the graph is shifted k units downwards.

From the above-calculated equation, it is clear that for the given equations g(x) = f(x) − 3, the shift is 3 units downwards.

The transformation of graph y = 3x + 3 as y = 3x can be described by a 3-unit downwards translation, so the correct option is C) translation 3 units down which was solved using the formula for the vertical shift.

Page 56 Exercise 22 Answer

In the question, it is given an equality as −2x + 5 < 1.

It is required to graph the solution of the inequality.

To solve the question, start with writing the given inequality and solve it using basic mathematical operations.

Finally, plot the obtained answer on the graph.

Write the given inequality.

Given inequality -2x+5<1

Add 2x to both sides

-2x+2x+5<1+2x

5<1+2x

Subtract 1 from both sides

5-1<1+2x-1

4<2x

Divide 2 on both sides

⇒ \(\frac{4}{2}<\frac{2 x}{2}\)

2<x

So the solution of the inequality lies on the right-hand side of the value 2 on the graph.

Plot the graph.

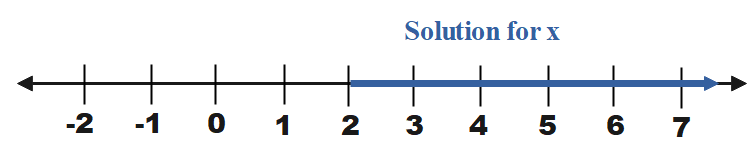
The graph for the given inequality i.e. −2x + 5 < 1 is as shown and the solution for the equation is given by x > 2.

Page 56 Exercise 24 Answer

In the question it is given that $500 has been deposited in an account that gives 2% interest compounded monthly.

It is required to use the formula A = \(P\left(1+\frac{r}{n}\right)^{n t}\) to determine how much money will be in the account after 5 years. To solve the question start with writing the given information in the question. Then substitute the given values in the formula. Simplify the formed equation using basic mathematical operations and a calculator to get the required answer.

According to the given

p=500
r=2%
n=12
t=5 years

Now substitute the given values into the formula

A= \(P\left(1+\frac{r}{n}\right)^{n t}\)

A= \(500\left(1+\frac{2}{12}\right)^{12(5)}\)

A= \(500\left(1+\frac{1}{6}\right)^{60}\)

A= \(500\left(\frac{6+1}{6}\right)^{60}\)

A=\(500\left(\frac{7}{6}\right)^{60}\)

A+500(10394.59)
A=51,97,295

So the amount calculated is $51,97,295.

When $500 is deposited as a principle in a bank with 2% interest compounded monthly, then after 5 years the amount accumulated in the account is calculated to be $51,97,295 which was solved using the formula A = \(P\left(1+\frac{r}{n}\right)^{n t}\)

Page 57 Exercise 25 Answer

In the question it is given two equations namely, y = \(\sqrt[3]{\frac{x}{6}}\) and y = \(\sqrt[3]{x}\).

It is required to conclude how the graph of the equation y = \(\sqrt[3]{\frac{x}{6}}\) can be described as the transformation of the graph of the parent function y = \(\sqrt[3]{x}\).

To solve the question start with writing the given equations as f(x) and g(x) respectively. Then, write the value of g(x) in terms of f(x) and compare it with the formula of the horizontal shift to get the required answer.

Write the parent equation as f(x) and g(x) as the second equation.

f(x) = \(\sqrt[3]{x}\)

g(x) = \(\sqrt[3]{\frac{x}{6}}\)

Now, rewrite the expression and find the value of g(x) interms of f(x) by substituting the value of x.

g(x) = \(\sqrt[3]{\frac{x}{6}}\)

g(x) = \(\sqrt[3]{\frac{1 x}{6}}\)

g(x) = \(f\left(\frac{x}{6}\right)\)

g(x) = \(f\left(\frac{1}{6} x\right)\)

Use the formula that for a parent function f(x). A new function g(x) = a⋅f(x), where a is a constant, results in a horizontal compression or vertical stretch of the function f(x).

a > 1, means the graph is horizontally stretched.

0 < a < 1, means the graph is horizontally compressed.

From the above-calculated equation, it is clear that for the given equations g(x) = \(f\left(\frac{1}{6} x\right)\).

so, a = \(\frac{4}{2}\)

Clearly. 0 < a < 1, so there is a horizontal compression by a factor of \(\frac{1}{a}\).

\(\frac{1}{a}\) = \(\frac{1}{\frac{1}{6}}\)

\(\frac{1}{a}\) = 6

Hence for the given equations of two graphs, the transformation from the parent function is horizontal compression by a factor of 6.

The transformation of graph \(\sqrt[3]{x}\) as y = \(\sqrt[3]{\frac{x}{6}}\) can be described by a horizontal compression by a factor of 6, so the correct option is B) horizontal compression by a factor of 6, which was solved using the formula for horizontal compression and stretch.

Page 57 Exercise 26 Answer

In the question, it is given a system of equations as y = −2x − 4 and y = −4x − 2.

It is required to find the solution of the system of these equations.

To solve the question, start by writing the given system of equations and substituting the value of y from one equation to another. Then, simplify the expression using basic mathematical operations until the value of x is obtained. Then substitute the value of x in any of the given equations to get the required answer.

​Given a system of equations

y=-2x-4
y=-4x-2
-4x-2=-2x-4

Add 4x on both sides

-4x-2+4x=-2x+4x-4
2x=2

divide 2 on both sides

⇒ \(\frac{2 x}{x}=\frac{2}{x}\)

x=1

Substitute this calculated values of x

y=-2(1)-4
y=-2-4
y=-6

So, the solution for the given system of equations is (x,y) = (1,−6).

The given system of equations i.e. y = −2x − 4 and y = −4x − 2 results in a solution value of x as 1 and y as −6 which was solved using basic mathematical operations.

Page 57 Exercise 27 Answer

In the question, a graph is given as

Also, four equations are given as

a. y = \(\sqrt{x+5}\) − 3

b. y = \(\sqrt{x-3}\) + 5

c. y = \(\sqrt{x-5}\) + 3

d. y = \(\sqrt{x+3}\) + 5

It is required to state which of the given options is an equation for the transformation of the graph of the parent function y = √x shown in the given graph.

To solve this, find the origin of the given graph and find the equation of the curve using the shifted origin.

Find the origin of the curve from the given graph and find the equation of the curve using the shifted origin.

The given graph is

From the graph origin of the curve is (−5,−3).

So, the equation of the curve is

⇒ \(\begin{aligned}
& y-(-3)=\sqrt{x-(-5)} \\
& y+3=\sqrt{x+5} \\
& y-(-3)=\sqrt{x-(-5)}
\end{aligned}\)

⇒ \(\begin{aligned}
& y+3=\sqrt{x+5} \\
& y=\sqrt{x+5}-3
\end{aligned}\)

So, option (A) is the correct option.

Option (A) y = \(\sqrt{x+5}\) – 3 is an equation for the transformation of the graph of the parent function y = √x shown in the given graph.

Page 57 Exercise 28 Answer

In the question, it is given that the formula for the volume of a cylinder in cubic feet is V = πr²h where r is the radius of the base in feet and h is the height in feet. Also, it is given that its volume is 1078πft³ and its height is 22 feet.

It is required to find the diameter of the cylinder.

To solve this, substitute the given volume and height in the equation V = πr²h and find r.

Then using this r, find the diameter d = 2r.

v=πr2h-1
v=1078π
h=22

Substitute the value in 1

v=πr2h
1078π=π.r2.22
Divide 22 on both sides

⇒ \(\begin{aligned}
& \frac{22 r^2}{22}=\frac{1078}{22} \\
& r^2=49
\end{aligned}\)

Square root on both sides

⇒ \(\begin{aligned}
\sqrt{r^2} & =\sqrt{49} \\
r & = \pm \sqrt{7^2} \\
r & = \pm 7
\end{aligned}\)

Since the radius is a length, it is positive

So,r=7

Diameter d=2r
d=2(7)
d=14

So, the diameter is 14ft.

By substituting the volume and height in the equation of volume, the radius of the cylinder is found as r = 7, and using this radius diameter is found as 14ft.

Page 57 Exercise 30 Answer

In the question, four pairs of points and four slopes are given as follows

(3, 1) and (5, -7) \(\frac{1}{4}\)

(10, 14) and (-2, 11) 4

(-11, 9) and (-12, 5) \(\frac{-1}{4}\)

(-2, 7) and (-10, 9) -4

It is required to match each pair of points to the slopes.

To match this, find the slope of each line using the formula m = \(\frac{y_2-y_1}{x_2-x_1}\) and match them according to the calculated slope.

Find the slope m1 of the line passing through (3,1) and (5,7)

⇒ \(\begin{aligned}
& m=\frac{y_2-y_1}{x_2-x_1} \\
& m_1=\frac{-7-1}{5-3} \\
& m_1=\frac{-8}{2} \Rightarrow m_1=-4
\end{aligned}\)

Find the slope m2 of the line passing through (10,14) and (-2,11)

⇒ \(\begin{aligned}
& m=\frac{y_2-y_1}{x_2-x_1} \\
& m=\frac{11-14}{-2-10} \\
& m_2=\frac{-3}{-12} \Rightarrow m_2=\frac{1}{4}
\end{aligned}\)

Find the slope m3 of the line passing through (-11,9) and (-12,5)

⇒  \(\begin{aligned}
& m=\frac{y_2-y_1}{x_2-x_1} \\
& m_3=\frac{5-9}{-12+11} \\
& m_3=\frac{-4}{-1} \Rightarrow m_3=4
\end{aligned}\)

Find the slope of the line passing through (-2,7) and (-10,9)

⇒ \(\begin{aligned}
& m=\frac{y_2-y_1}{x_2-x_1} \\
& m_4=\frac{9-7}{-10+2} \\
& m_4=\frac{2}{-8} \\
& m_4=\frac{-1}{4}
\end{aligned}\)

Match the points and the calculated slopes.

(3, 1) and (5, -7) -4

(10, 14) and (-2, 11) \(\frac{1}{4}\)

(-11, 9) and (-12, 5) 4

(-2, 7) and (-10, 9) \(\frac{-1}{4}\)

Page 58 Exercise 31 Answer

In the question, it is given that the ages of the members of a beach clean-up club are 16, 19, 22, 25, 31, 33, 38 and 42.

Also, it is given that a new member who is $19$ years old joins the club.

It is required to describe how the age of the new member will affect the mean, median, mode, and range of the ages of the club.

To solve this, first find the mean, median, mode, and range of the ages without the age of the new member. Then find the mean, median, mode, and range of the ages including the age of the new member. Compare the obtained answers in both cases to describe how the age of the new member will affect the mean, median, mode, and range.

Find the mean of the ages without the age of the new member.

Mean = \(\frac{16+19+22+25+31+33+38+42}{8}\)

Mean= \(\frac{226}{8}\)

Mean= 28.25

Find the median ages without age of new member

The middle numbers are 25 and 31

Median= \(\frac{25+31}{2}\)

Median= \(\frac{56}{2}\)

Median=28

Find the mode of the ages without the age of the new member.

Mode is the highest occurring number. Here every number occurs only once.

So, no mode exists.

Find the range of the ages without the age of the new member.
​
​Range = 42 − 16

= 26

Find the mean of the ages with the age of the new member.

mean = \(\frac{16+19+19+22+25+31+33+38+42}{9}\)

Mean= \(\frac{245}{9}\)

Mean= 27.22

Find the median of the ages with the age of the new member.

The middle number is 25.

Median = 25

Find the mode of the ages with the age of the new member.
​
​Mode = highest occurring number

= 19

Find the range of the ages with the age of the new member.
​
​Range = 42 − 16

= 26

The addition of new members with 19 years old will reduce the mean as 19 is small relative to the given data.

Median becomes a single number as there are an odd number of terms.

Mode is possible when the new member is added as there are two numbers of the same value.

The range will be the same as the highest and lowest numbers are the same.

Page 58 Exercise 32 Answer

In the question, an expression 8x⁵ − 26x⁴ + 6x³ is given.

It is required to find the factored form of 8x⁵ − 26x⁴ + 6x³ and to explain it.

To solve this, first take 2x³ common from the given expression. Then factorize the remaining function.

Given expression 8x⁵ − 26x⁴ + 6x³

Take 2x³ common from the expression

8×5-263=2×3(4×2-13x+3)

Factorise 4×2-13x+3

4×2-13x+3=4×2-12x-x+3
=4x(x-3)-1(x-3)

Take(x-3) common from the above equation
4x(x-3)-1(x-3)=(4x-1)(x-3)

take (x-3) common from the above equation

By taking 2x³ common and factorizing the remaining term, the factorization of 8x⁵ − 26x⁴ + 6x³ is found as 2x³(x−3)(4x−3).

Page 58 Exercise 33 Answer

In the question, it is given that the measure of angle A of an obtuse triangle is two times the measure of angle B, while the measure of angle C is 28° less than the measure of angle B.

It is required to explain what are the degree measures of the three angles.

To find this, take ∠A as 2∠B and ∠C as ∠B−28 in ∠A + ∠B + ∠C = 180° and find ∠B. Using the value of ∠B find the other two angles.

∠A+∠B+∠C=180°

Substitute ∠A=2∠B
∠C=∠B-28°

2∠B+∠B+∠B-28°=180°
4∠B=180°+28°
4∠B=208°

Divide 4 on both sides

⇒ \(\frac{4 \angle B}{4}=\frac{208^{\circ}}{4}\)

∠B=52°

Find ∠A by substituting the value of ∠B as 52°

∠A=2∠B
∠A=2(52)°
∠A=104°

Find ∠C by substituting the value of ∠B is 52°

∠C=∠B-28°
∠C=52°-28°
∠C= 24°

By taking ∠A as 2∠B and ∠C as ∠B − 28 in ∠A+∠B+∠C = 180°, the values of the three angles are found as

∠A=104°

∠B = 52°

∠C = 24°

Page 58 Exercise 34 Answer

In the question, a function f(x) = 9x² + 15x − 6 is given.

It is required to find what are the zeros of the function f(x) = 9x² + 15x − 6.

To find the zeros, put f(x) = 0 and solve using mathematical operations.

Put 9x² + 5x − 6 = 0 and simplify to find the zeros.

Given function f(x)=9x²+15x-6

⇒ \(\begin{aligned}
& 9 x^2+15 x-6=0 \\
& 9 x^2+18 x-3 x-6=0 \\
& 9 x(x+2)-3(x+2)=0 \\
& (9 x-3)(x+2)=0
\end{aligned}\)

​Take 3 common from (9x-3)

(9x-3)(x+2)=0
3(3x-1)(x2)=0
(3x-1(x+2)=0

Here(3x-1) is o or (x+2) is o by the  property, if ab=0

Put(3x-1)=0 and find x

3x-1=0
3x=1
⇒ \(x=\frac{1}{3}\)

Put (x+2)=0 and find x
x+2=0
x=-2

By taking 9x² + 15x − 6 = 0, the zeros of the function are found as x = \(\frac{1}{3}\) and x = −2.

Page 59 Exercise 35 Answer

In the question, it is given that Dakota has a combination of 18 dimes and quarters for a total of $2.70.

It is required to write a system of equations used to determine the number of each type of coin Dakota has and to determine how many coins of each type Dakota has.

To solve this, let the number of dimes as x and the number of quarters as y. The given condition is Dakota has a combination of 18 dimes and quarters. Convert the condition in terms of $. Then solve the two equations to find x.

Convert the given condition Dakota has a combination of $18$ dimes and quarters to equation form.

x+y=18
Put 1dime=$0.1
1quarter=$0.25 given condition

x+y=18
0.1+0.25y=2.7
x+2.5y=27

Solve the two equations x+y=18 and

x+2.5y=27
x.x+2.5y-y=27-18
1.5y=9
y=6

Substitute the value of y as 6

x+y=18
x+6=18
x=18-6
x=12

The system of equations used to determine the number of each type of coin Dakota has been x + y = 18.

The number of dimes is 12 and the number of quarters is 6.

Page 59 Exercise 36 Answer

In the question, a piecewise-defined function is given as

f(x) = \(\left\{\begin{array}{l}
4 x+7, \text { if } x<-2 \\
-x, \text { if }-2<x \leq 2 \\
1, \text { if } x>2
\end{array}\right.\)

It is required to graph the given piecewise function.

To plot this first take y = f(x) and find the coordinates by substituting different numbers in the range to the specified value of the function.

​Put x as -3 in the function y=4x+7

y=4(-3)+7
y=-12+7
y=-5

So, the coordinates are (-3,-5)

Put x as -4 in the function y=4x+7

y=4(-4)+7
y=-16+7
y=-9

So, the coordinates are (-4,9)

Put x as -1 in the function y=-x
y=-x
y=-(-1)
y=1

So, the coordinates are (-1,1)

Put x as 2 in the function y=x
y=-x
y=-2
so, the coordinates is (2,-2)

Put x as 3 in the function y = 1.

The coordinate is (3,1).

Put x as 4 in the function y = 1.

The coordinate is (4,1).

Plot the calculated point in the coordinate plane and draw the graph.

The points are plotted on the coordinate plane as

In the question, a function

f(x) = \(\left\{\begin{array}{l}
4 x+7, \text { if } x<-2 \\
-x, \text { if }-2<x \leq 2 \\
1, \text { if } x>2
\end{array}\right.\) is given.

It is required to find the domain and range of the function.

To solve the question, use the fact that the domain of all the polynomials is (−∞,∞).

Find the range for each interval separately and then take their union.

Find the domain of the function.

As it is given that

f(x) = \(\left\{\begin{array}{l}
4 x+7, \text { if } x<-2 \\
-x, \text { if }-2<x \leq 2 \\
1, \text { if } x>2
\end{array}\right.\)

Now, the function f(x) has a polynomial at every interval. So, the domain of the function is (−∞,∞).

Find the range of the function.

For x < −2, f(x) = 4x + 7

Also, ​

​f(−2) = 4(−2) + 7

= −1
​
Thus the range of the function is (−∞,−1) when x < −2.

For −2 < x ≤ 2, f(x) = −x

Thus the range of the function is [−2,2] for −2 < x ≤ 2.

Solve further to get the range of function f(x).

For x > 2, f(x) = 1.

So, the range of the function for the interval x > 2 is 1.

Now, the range of the function f(x),
​
​R = (−∞,−1)∪[−2,2)∪{1}

= (−∞,2)

Thus, the range of the function f(x) is (−∞,2).

Therefore, the domain of the function f(x) is (−∞,∞) and the range is (−∞,2) which were solved according to definitions of domain and range.

In the question, a function

f(x) = \(\left\{\begin{array}{l}
4 x+7, \text { if } x<-2 \\
-x, \text { if }-2<x \leq 2 \\
1, \text { if } x>2
\end{array}\right.\) is given.

It is required to find out on which interval function is increasing or decreasing.

To solve the question, for each interval find the first derivative of the function. If f′(x) < 0, then the f(x) is increasing on that interval. And if f′(x) > 0, then the f(x) decreases on that interval.

Find the intervals where function is increasing or decreasing.

For x < −2, f(x) = 4x + 7

​f′(x) = 4

f′(x) > 0
​
So, f(x) is decreasing on the interval (−∞,−2).

Solve further for more intervals.

For −2 < x ≤ 2, f(x) = −x

​f′(x) = −1

f′(x) < 0
​
So, f(x) is increasing on the interval [−2,2].

Solve further for more intervals.

For x > 2, f(x) = 1.

f′(x) = 0

Since f′(x) = 0 the function is constant in the interval (2,∞).

Therefore, function f(x) is decreasing on the interval (−∞,−2) and increasing on the interval [−2,2] which were solved by calculating the first derivative of the function at each interval.

Page 60 Exercise 1 Answer

In the question, it is given that a person named Kayden is considering three different ways to rent movies and video games.

a. Rent movies and game disks for $2 each.

b. Unlimited games and movies to any device for $20 per month.

c. Rent movies and game disks for a $10 monthly fee plus $2.5 per disk.

It is required to write the functions that give the cost to rent x movies or disks per month for each plan, that is A(x), B(x), and C(x). Then graph the functions.

To solve the question, analyze the given data carefully and write functions accordingly for each plan. Then graph the functions.

Write functions for each plan that give the cost to rent x movies or disks per month for each plan.

For plan A, the cost function is A(x)=2x,

For plan B, the cost function is B(x)=20,

For plan C, cost function is C(x) = 10 + 2.5x

Plot the above functions on the graph.

A(x) = 2x

C(x) = 10 + 2.5x

 

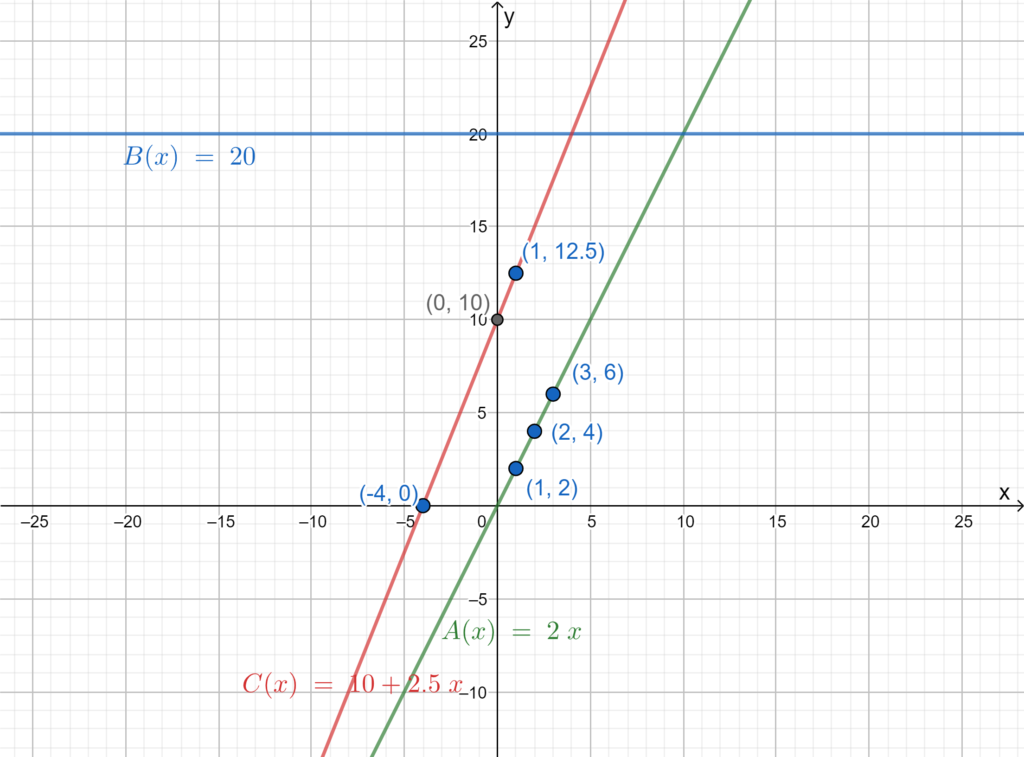
Thus, cost functions for

plan A is A(x) = 2x

plan B is B(x) = 20

plan C is C(x) = 10 + 2.5x,

which were solved by forming cost functions for the cost of x items.

The graph of the functions is

In the question, it is given that a person named Kayden is considering three different ways to rent movies and video games.

Rent movies and game disks for $2 each.

Unlimited games and movies to any device for $20 per month.

Rent movies and game disks for a $10 monthly fee plus $2.5 per disk.

Also from the result of the previous part, the costs functions for

plan A is A(x) = 2x

plan B is B(x) = 20

plan C is C(x) = 10 + 2.5x

It is required to tell under what conditions Plan B is better than plan A if only cost is considered.

To solve the question, analyze the given data carefully. Now plan B is better than A if the cost of B is less than A. Solve the inequality and get the conditions using suitable mathematical operations.

Analyze the given data carefully.

For plan A, the cost function is A(x) = 2x,

For plan B, the cost function is B(x) = 20,

Now, the Cost of plan B is less than plan A when 20 < 2x

Divide by 2 on both sides.

\(\frac{20}{2}<\frac{2 x}{2}\)

x > 0

Now, if the number of disks is more than 10, plan A will be more costly than plan B where cost is constant.

Thus, when the number of disks is more than 10, plan B is better than plan A.

Thus, when the number of disks is more than 10, plan B is better than plan A which was solved by analyzing data and using appropriate mathematical operations.

In the question, it is given that a person named Kayden is considering three different ways to rent movies and video games.

Rent movies and game disks for $2 each.

Unlimited games and movies to any device for $20 per month.

Rent movies and game disks for $10 monthly fee plus $2.5 per disk.

Also from the result of the previous part, the cost functions for

plan A is A(x) = 2x

plan B is B(x) = 20

plan C is C(x) = 10 + 2.5x

It is required to tell under what conditions plan C is better than plan B, if only cost is considered.

To solve the question, analyze the given data carefully. Now plan C is better than B if the cost of C is less than B. Solve the inequality and get the conditions using suitable mathematical operations.

Analyze the given data carefully.

For plan B, the cost function is B(x) = 20,

For plan C, cost function is C(x) = 10 + 2.5x

The cost of plan C is less than plan B if 10 + 2.5x < 20

B(x)=20
c(x)=10+2.5x
10+2.5x<20
subtract 10 on both sides

10-10+2.5x<20-10
2.5x<10

Divide both sides by 2.5

\(\begin{gathered}
\frac{2.5 x}{2.5}<\frac{10}{2.5} \\
x<4
\end{gathered}\)

Now, if the number of disks is less than 4, plan C is less costly than plan B.

Thus, when the number of disks is less than 4, plan C is better than plan C which was solved by analysing data and using appropriate mathematical operations.

In the question, it is given that a person named Kayden is considering three different ways to rent movies and video games.

Rent movies and game disks for $2 each.

Unlimited games and movies to any device for $20 per month.

Rent movies and game disks for $10 monthly fee plus $2.5 per disk.

Also from result of previous part, costs functions for

plan A is A(x) = 2x

plan B is B(x) = 20

plan C is C(x) = 10 + 2.5x

It is required to show that Plan A is always more cost effective than Plan C.

To solve the question, analyse given data carefully. Compare costs of two plans by subtracting cost of plan A from plan C. If result is positive that means cost of plan A is always less than C.

Compare costs of plan C and plan A.

For plan A, cost function is A(x) = 2x,

For plan C, cost function is C(x) = 10 + 2.5x

Find their differences.

​C(x) − A(x) = 10 + 2.5x − 2x

= 10 + 0.5x
​
Now, since x is the number of disks, thus it cannot be negative. This means the difference is always positive.

Solve further for conclusion.
​
​C(x) − A(x) > 0

C(x) > A(x)
​
Thus, the cost of plan A is always less than plan C.

Therefore, it is shown that plan A is always cost-effective than plan C which was solved by comparing two costs.

Proof: A(x)=2x
C(x)=10+2.5x
C(x)-A(x)=10+2.5x-2x
=10+0.5x
C(x)-A(x)>0
C(x)>A(x)

Hence showed

In the question, it is given that a person named Kayden is considering three different ways to rent movies and video games.

Rent movies and game disks for $2 each.

Unlimited games and movies to any device for $20 per month.

Rent movies and game disks for $10 monthly fee plus $2.5 per disk.

Also from result of part(a), costs functions for

plan A is A(x) = 2x

plan B is B(x) = 20

plan C is C(x) = 10 + 2.5x

From part (b), plan B is more cost-effective than plan A when the number of disks is more than 10.

As a result of part (d), plan A is always more cost-effective than plan C.

It is required to recommend a plan to Kayden and justify the answer.

To solve the question, analyze the given data and results of previous steps carefully.

Since plan A is always more cost-effective than plan C. Plan B is more cost-effective than plan A when the number of disks is more than 10. It is more likely that he needs less than 10 disks. So, plan A is recommended.

Therefore, plan A) Rent movies and game disks for $2 each, is recommended as it is more likely that Kayden needs less than 10 disks per month.