Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice
Page 269 Problem 1 Answer
Given- The standard form of a quadratic equation.
To find- An example for the standard form of a quadratic function and determine the x−intercepts, they−intercept, or the vertex of the graph, and the concavity of a parabola.
We can give an example of a quadratic equation in standard form, then describe the process to determine the x−intercept,y−intercept, the vertex, and the concavity of the parabola using the general quadratic equation in standard form.
The standard form of the quadratic function is of the form f(x)=ax2+bx+c, wherea,b,and c are real numbers with a≠0.
An example of a quadratic function in standard form isf(x)=3x2+6x−8
Here a=3
b=6
c=−8
To find the x−intercepts algebraically, we substitute y=0 in the equation and then solve for values of x.
In the same manner, to find for y−intercepts algebraically, we substitute x=0 in the equation and then solve for y.
To find the vertex of a quadratic equation,y=ax2+bx+c, we find the point{−b/2a,a(−b/2a)2+b(−b/2a)+x} by following these steps.
At first, we get the equation in the form y=ax2+bx+c.We calculate−b/2a.
This is the x−coordinate of the vertex.
To find they−coordinate of the vertex, we substitute x=−b/2a in the equation solve for y.
This is they−coordinate of the vertex.
Now, for determining the concavity of the function f(x)=ax2+bx+c, we will calculate its second derivative.
So,f(x)′′=2a
Thus, we have the concavity of the function f′′
(x)=2a, we can say that the sign off′′(x) directly depends on the sign of the coefficient a, as2 is a positive number.
If a will be positive,2a will be positive, whereas if a will be negative,2a will be negative.
Therefore, we can say that a directly relates with the concavity of the function, as if a is positive,f′′(x) will be positive and the function will be concave up. If a is negative,f′′(x) will be negative and the function will be concave down.

Hence, for a quadratic function f(x)=ax2+bx+c,Ifa>0, thenf(x) is concave upward of the parabola,
Ifa<0, thef(x) is concave downward of the parabola.
An example of a quadratic function in standard form is f(x)=3x2+6x−8
The x−intercept of the equation is the x−values when y=0.
They−intercept of the equation is they−values when x=0.
The vertex of the quadratic function f(x)=ax2+bx+c is the point{−b/2a,a(−b/2a)2+b(−b/2a)+c}
The concavity of parabola of the equation f(x)=ax2+bx+c is given by,
Ifa>0, the parabola is concave upward.
Ifa<0, the parabola is concave downward.
Carnegie Learning Algebra Ii Chapter 2 Exercise 2.1 Solutions
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 269 Problem 2 Answer
Given- The factored form of a quadratic equation.
To find- An example for the factored form of a quadratic function and determine the x−intercepts, the y−intercept, or the vertex of the graph, and the concavity of a parabola.
We can give an example of a quadratic equation in standard form, then describe the process to determine the x−intercept, y−intercept, the vertex, and the concavity of the parabola using the general quadratic equation in factored form.
The factored form of the equation for a quadratic equation isy=a(x−r)(x−s) a product of three factors.
The values a,r,s are values that also determine the shape and position of the parabola.
An example of a quadratic function in factored form isy=2(x−5)(x−7)
Here,a=2
r=5
s=7
To find the x−intercepts algebraically, we substitute y=0 in the equation and then solve for values of x.
In the same manner, to find for y−intercepts algebraically, we substitute y=0 in the equation and then solve for y.
To find vertex in factored form, we have to find the axis of symmetry and substitute the value of x and solve for y.
The axis of symmetry can be calculated given the formula,x=r+s/2, where r and s are zeroes of the equation.
Now, for determining the concavity of the function f(x)=a(x−r)(x−s) we will calculate its second derivative.
So,f′′(x)=2a
Thus, we have the concavity of the functionf′′ (x)=2a, we can say that the sign off′′(x)
directly depends on the sign of the coefficient a, as2 is a positive number.
If a will be positive,2a will be positive, whereas if a will be negative,2a will be negative.
Therefore, we can say that a directly relates to the concavity of the function, as if a is positive,f′′(x)
will be positive and the function will be concave up. If a is negative,f′′ (x) will be negative and the function will be concave down.
Hence, for a quadratic function f(x)=a(x−r)(x−s),
Ifa>0, then f(x) is concave upward of the parabola,
Ifa<0, then f(x) is concave downward of the parabola.
An example of a quadratic function in factored form isy=2(x−5)(x−7)
The x−intercept of the equation is the x−values when y=0.
They−intercept of the equation is they−values whenx=0.
To find vertex in factored form, we have to find the axis of symmetry and substitute the value of x=r+s/2 and solve for y.
The concavity of parabola of the equationf(x)=a(x−r)(x−s) is given by,
Ifa>0, the parabola is concave upward.
Ifa<0, the parabola is concave downward.
Page 269 Problem 3 Answer
Given- The vertex form of a quadratic equation.
To find- An example for the vertex form of a quadratic function and determine the x−intercepts, they−intercept, or the vertex of the graph, and the concavity of a parabola.
We can give an example of a quadratic equation in vertex form, then describe the process to determine the x−intercept, y−intercept, the vertex, and the concavity of the parabola using the general quadratic equation in factored form.
The vertex form of a quadratic equation isy=a(x−h)2+k
Here,y is the y−coordinate,x is the x−coordinate, and a is the constant that tells you whether the parabola is facing up (+a) or down(−a).
The coordinate(h,k) gives the vertex of the parabola.
An example of a quadratic function in factored form isy=5(x−6)2+8
Here,a=5
h=6
k=8
They−intercept is the point at which the parabola crosses they−axis.
The x−intercepts are the points at which the parabola crosses the x−axis.
The x−intercept is the value of x at y=0.
They−intercept is the value of y at x=0.
The vertex of the equation is given by(h,k), which can be calculated by computi
Now, for determining the concavity of the function f(x)=a(x−h)2+k we will calculate its second derivative.
So,f′′(x)=2a
Thus, we have the concavity of the function f′′(x)=2a, we can say that the sign off′′(x) directly depends on the sign of the coefficient a,as2 is a positive number.
If a will be positive,2a will be positive, where as if awill be negative,2a will be negative.
Therefore, we can say that a directly relates to the concavity of the function, as if a is positive,f′′(x)
will be positive and the function will be concave up.
If a is negative,f′′(x) will be negative and the function will be concave down.
Hence, for a quadratic function f(x)=a(x−h)2+k,
Ifa>0, then f(x) is concave upward of the parabola,
Ifa<0 An example of a quadratic function in factored form is y=5(x−6)2+8
The x−intercept of the equation is the x−values when y=0.
They−intercept of the equation is they−values when x=0.
The vertex of the equation is given by(h,k), which can be calculated by computing h=−b/2a, and then evaluating y ath to find k.
The concavity of parabola of the equationy=a(x−h)2+k is given by,
Ifa>0, the parabola is concave upward.
Ifa<0, the parabola is concave downward.
Skills Practice Exercise 2.1 Answers
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 269 Problem 4 Answer
Given- Concavity of a parabola of a quadratic function.
To find- To describe how to determine the concavity of a parabola.
We can determine the concavity of a parabola by calculating the second derivative of the quadratic function.
For a quadratic function f, we can determine the concavity by finding the second derivative.
The seconds derivative of the function is given by f′′=2a, where a is the coefficient of x2.
So,f′′ is directly related to the sign of a.
When a will be positive,f′′=2a is positive.
When a will be negative,f′′=2a is negative.
In any quadratic function f, if the second derivative f′′ is positive, then the function is concave up.
And, If the second derivative f′′ is negative, then the function is concave down.
Concavity of a parabola shows that the parabola is concave up when it bends up, and concave down when it bends down.
It can be determined by calculating the second derivative of the quadratic function f.
So,f′′=2a, where a is the coefficient of x2.
When a is positive,f′′=2a is positive and the parabola is concave up.
When a is negative,f′′ =2a is negative and the parabola is concave down.
Page 270 Problem 5 Answer
Given equations of four parabolas and a parabola plotted on the graph.
We need to match the parabola on the graph to its correct equation out of four equations given to us.
The given parabola on the graph : (Value of one smallest box on the x and y axes is 0.5 units)

Investigating each of the given equations:
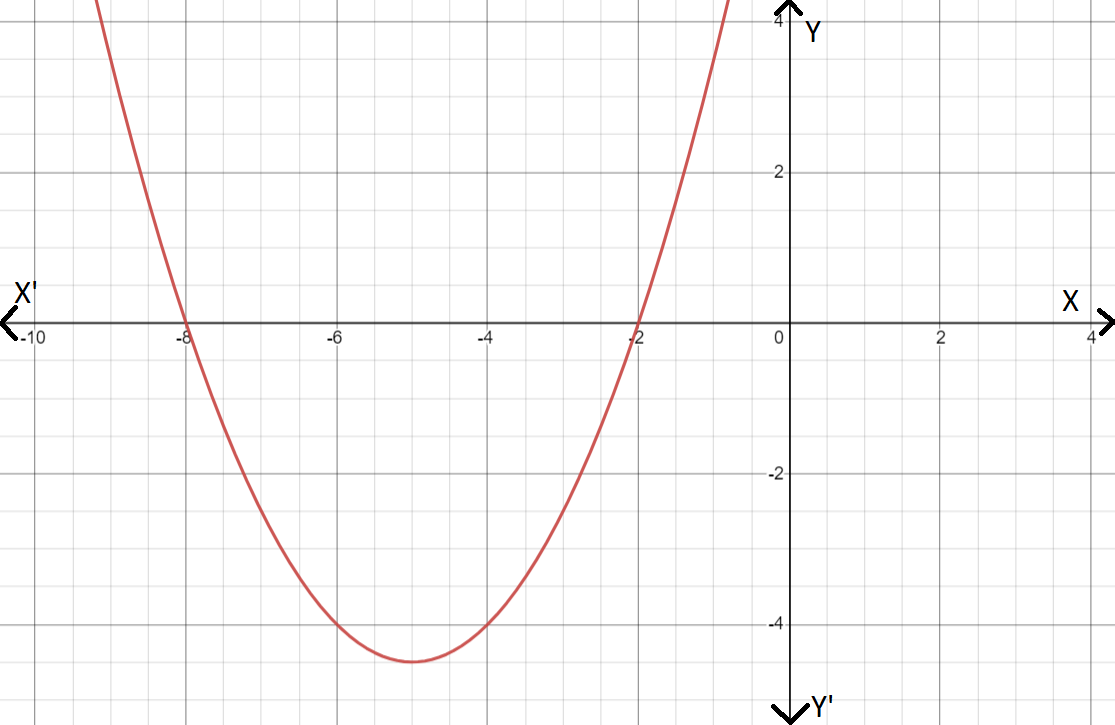
f(x)=6(x−2)(x−8): If we choose any point between −2 and −8, say choose x=−4
Now 6(x−2)(x−4)=288>0 at x=−4.
But according to the graph y=−4 at x=−4. Hence this function does not match the graph.
f(x)=−1/2(x+2)(x+8) : If we choose the point x=−4
Now −1/2(x+2)(x+8)=6>0 at x=−4.
But according to the graph y=−4 at x=−4. Hence this function does not match the graph.
f(x)=1/2(x+2)(x+8): Now choose the point x=−4
Now 1/2(x+2)(x+8)=−4<0
at x=−4.
According to the graph also y=−4 at x=−4. Thus it satisfies this condition but it may or may not be true.
f(x)=1/2(x−2)(x−8): The value of f(x) at x=−2 is 20≠0
But according to the graph, at x=−2, the value of the graph is 0
Hence this function does not match the graph.
Thus the correct option is f(x)=1/2(x+2)(x+8).
Circling the function that matches the graph is:
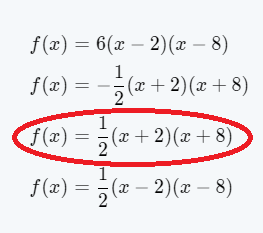
Page 270 Problem 6 Answer
Given equations of four parabolas and a parabola plotted on the graph.

We need to match the parabola on the graph to its correct equation out of four equations given to us.
Investigating the given function equations:
f(x)=−2x2−x−2: This function cannot cannot match the given graph as at x=0, f(x)=−2
but according to the graph y=7 at x=0.
Hence this function does not match the graph. (Value of one smallest box along x and y axes is 0.5units)
f(x)=2x2−x+7: The value of f(x)=8 at x=1.
But according to the graph the value of y at x=1 is some value around 4.
Hence this function does not match the given graph.
f(x)=−x2−2x+7: The value of f(x)
at x=1/2 is 23/4. But according to the graph , y=6
at x=1/2
Hence this function does not match the graph.
f(x)=−2x2−x+7: The value of f(x)=6 which is the same as the value of y in the graph corresponding to x=1/2.
Since no other function matches the graph but this function matches some points corresponding to the graph, hence this function matches the graph.
Circling the equation of the parabola to the graph:

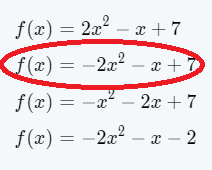
Algebra Ii Chapter 2 Skills Practice Solutions Exercise 2.1
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 270 Problem 7 Answer
Given equations of four parabolas and a parabola plotted on the graph.
We need to match the parabola on the graph to its correct equation out of four equations given to us.
From the graph it is clear that at x=4
we have y=2
In the function f(x)=4(x−2)2−2, the value of f(x)=14 at x=4. Hence this function does not match the graph.
f(x)=0.25(x−2)2+4: The value of f(x)=5 at x=4 and hence this function does not match the graph .In the function −0.25(x+4)2+2, the value of f(x)=−2 at x=4.
Hence this function also does not match the graph .But for the function f(x)=0.25(x−4)2+2, the values of f(x) correspond to the y values in the graph for all values of x
Thus circling the correct equation :

Page 270 Problem 8 Answer
Given equations of four parabolas and a parabola plotted on the graph.
We need to match the parabola on the graph to its correct equation out of four equations given to us.
From the graph it is clear that y=0
at x=−2,x=−5
For the functions: f(x)=−3(x+2)(x−5), the value of f(x)≠0 at x=−5
f(x)=3(x−2)(x−5), the value of f(x)≠0 at x=−2,x=−5
f(x)=−3(x−2)(x−5), the value of f(x)≠0 at x=−2,x=−5
But for the function f(x)=3(x+2)(x+5), the value of f(x) correspond to the y-value for all values of x.
Circling the equation that match with the graph given:
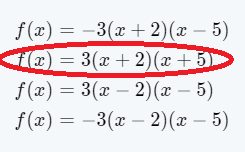
Page 271 Problem 9 Answer
Given equations of four parabolas and a parabola plotted on the graph.
We need to match the parabola on the graph to its correct equation out of four equations given to us.
The graph given:
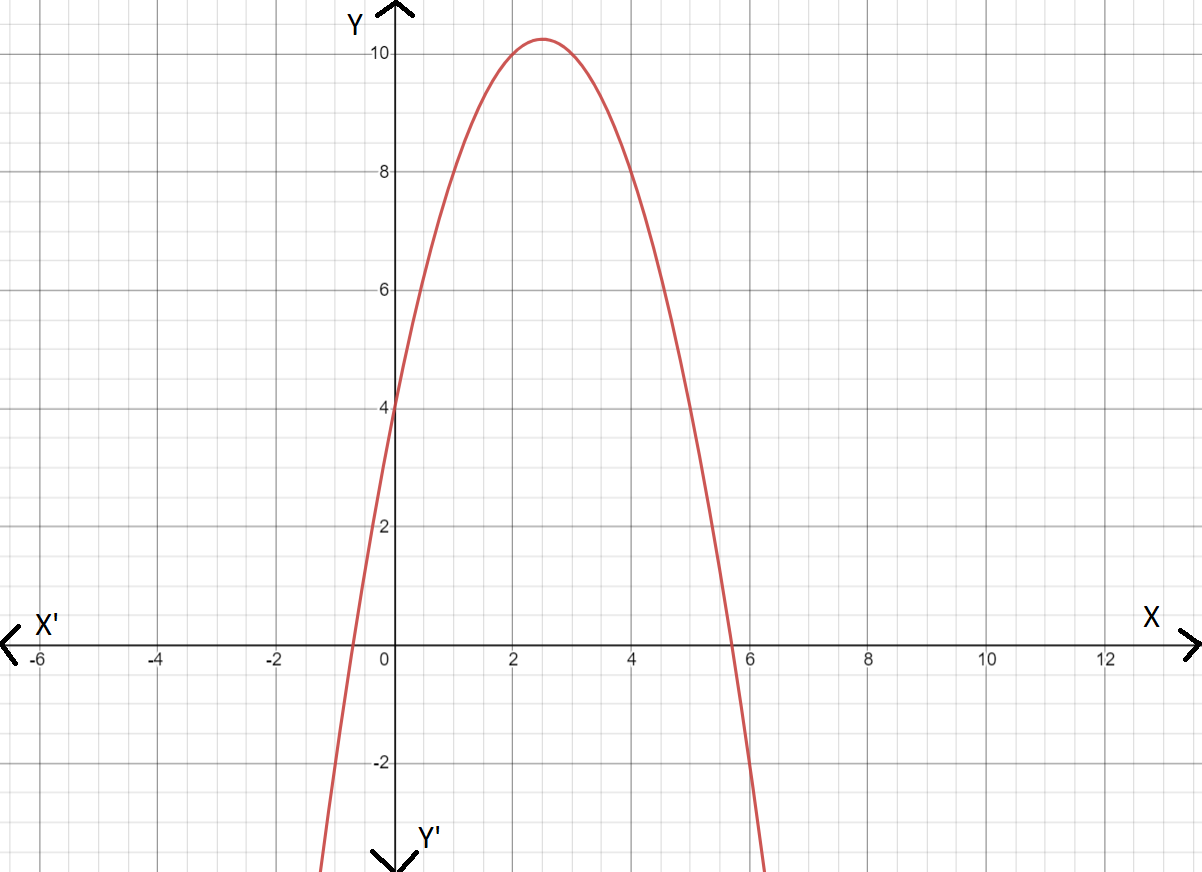
In the graph we can see that x=0
we find y=4
Thus the functions f(x)=x2+5x−4 and f(x)=−x2+5x+10 , at the point x=0
gives the value f(x)=−4 and f(x)=10 respectively.
Hence these functions does not match the graph.
Now if we put f(x)=0 in the equation f(x)=x2+5x+4, we find the corresponding values of x, where the graph cuts the x-axis.
⇒x2+5x+4=0
⇒(x+4)(x+1)=0
⇒x=−4
x=−1
But in the graph the parabola cuts the x-axis at both positive and negative points. Hence this function does not match the graph.
Thus the only option that remains: f(x)=−x2+5x+4 is the function that matches the graph.
Encircling the correct option we get:

Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 271 Problem 10 Answer
Given equations of four parabolas and a parabola plotted on the graph.
We need to match the parabola on the graph to its correct equation out of four equations given to us.
From the given graph we can see : y=0
at x=2
and y=2
at x=0
In the functions f(x)=1/2(x−2)2+2 and f(x)=1/2(x+2)2 , when we put x=2, the value of f(x)≠0
Hence these functions does not match the graph.
In the function f(x)=−1/2(x−2)2, the value of f(x)=−2 at x=0. Hence this also does not match the graph.
Hence the function f(x)=1/2(x−2)2 is the equation that matches the graph.
Encircling the correct option we get:

Page 271 Problem 11 Answer
Given the y-intercept value and the axis of symmetry .
To determine the most efficient form of the quadratic function satisfying these conditions.
The axis of symmetry of the quadratic function y=ax2+bx+c is given by −b/2a
Given axis of symmetry = −3/8
According to the problem:
−3/8=−b/2a
⇒b=3/4a (Multiplying both sides by 2a)
Now as the point (0,3) satisfies the equation :
3=0+0+c
⇒c=3
Hence the most efficient form obtained: y=ax2+3/4ax+3
The quadratic function obtained is : y=ax2/+3/4ax+3
Page 271 Problem 12 Answer
Given three points that lies on the quadratic function.
To find the most efficient form of the quadratic function with this data.
Since the point (−1,12)
lies on the function, hence it satisfies the equation:
12=a−b+c…(1)
The point (5,12) lies on the function, hence it satisfies the equation:
12=25a+5b+c…(2)
The point (−2,−2) lies on the function, hence it satisfies the equation:
−2=4a−2b+c….(3)
Now doing the operation on equations 4×(1)−(3):
50=−2b+3c….(4)
Now doing the operation 25×(1)−(2):
288=24c−30b….(5)
Now doing the operation 8×(4)−(5):
400−288=30b−16b
⇒112=14b (Combining like terms)
⇒b=8 (Dividing both sides by 14)
Thus putting value of b in the equation (4)
we get: 50=−2(8)+3c
⇒50=−16+3c
⇒66=3c (Adding 16 on both sides)
⇒22=c (Dividing both sides by 3)
Now putting back the value of c and b in the equation (1)
we get: 12=a−8+22
⇒12=a+14 (Combining like terms)
⇒a=12−14 (Subtracting 14 from both sides)
⇒a=−2 (Combining like terms)
Hence the quadratic function looks like: y=−2x2+8x+22
The most efficient form of the quadratic function is y=−2x2+8x+22
Carnegie Learning Skills Practice Exercise 2.1 Explained
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 272 Problem 13 Answer
To convert factored form f(x) = (x+5)(x+7) into standard form.
f(x) = (x+5)(x+7)
f(x) = x2+5x−7x−35
f(x) = x2−2x−35.
Therefore, the standard form of quadratic equation is f(x) = x2−2x−35.
Page 272 Problem 14 Answer
To convert factored form f(x) = (x+2)(x+9) into standard form.
f(x) = (x+2)(x+9)
f(x) = x2+9x+2x+18.
f(x) = x2+11x+18.
Therefore, the standard form of quadratic equation is f(x) = x2+11x+18.
Page 272 Problem 15 Answer
To convert factored form f(x) = 2(x-4)(x+1) into standard form.
f(x) = 2(x-4)(x+1)
f(x) = 2(x2+x−4x−4).
f(x) = 2(x2−3x−4).
f(x) = 2x2−6x−8.
Therefore, the standard form of quadratic equation is f(x) = 2x2−6x−8.
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 272 Exercise 1 Answer
To convert factored form f(x) = -3(x-1)(x-3) into standard form.
f(x) = -3(x-1)(x-3)
f(x) = -3(x2−3x−x+3)
f(x) = -3(x2−4x+3)
f(x) = -3x2 +12x−9.
f(x) = 3x2−12x+9.
Therefore, the standard form of quadratic equation is f(x) =
Page 272 Exercise 2 Answer
To convert vertex form f(x) = 1/3 (x+3)(x+7) into standard form.
f(x) = 1/3(x+3)(x+7)
f(x) = 1/3(x2+7x+3x+21)
f(x) = 1/3(x2+10x+21)
f(x) = x2/3+10x/3+7
Therefore, the standard form of quadratic equation is f(x) =
Page 272 Exercise 3 Answer
To convert factored form f(x) = −5/8(x−6)(x+2). into standard form.
f(x) = −5/8(x−6)(x+2)
f(x) = −5/8(x2+2x−6x−12).
f(x) = −5/8(x2−4x−12)
f(x) = −5/8x2+5/2x+15/2
f(x) = 5/8x2−5/2x−15/2
Therefore, the standard form of quadratic equation is f(x) = 5/8x2−5/2/x−15/2
Page 272 Exercise 4 Answer
To convert vertex form f(x) = 3(x-4)2 + 7. into standard form.
f(x) = 3(x−4)2+7
f(x) = 3(x2+16−8x)+7
Therefore, the standard form of quadratic equation is f(x) = 3x2−24x+55.
Chapter 2 Exercise 2.1 Skills Practice Guide
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 272 Exercise 5 Answer
To convert vertex form f(x) = −2(x+1)2−5.into standard form.
f(x) = −2(x2+1+2x)−5
f(x) = −2x2−2−4x−5
f(x) = −2x2 −4x−7
f(x) = 2x2+4x+7.
Therefore, the standard form of quadratic equation is f(x) = 2x2+4x+7.
Page 272 Exercise 6 Answer
Given that the quadratic function is,
f(x)=2(x+7/2)2−3/2
The given function is in vertex form.
Now we have to convert the vertex form into the standard form.
Consider the given function
f(x)=2(x+7/2)2−3/2
=2(x2+49/4+2(x)(7/2))−3/2
=2x2+49/4(2)+14x−3/2
=2x2+49/2+14x−3/2
=2x2+14x+46/2
=2x2+14x+23
The standard form of the equation f(x)=2(x+7/2)2−3/2 is 2x2+14x+23.
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 272 Exercise 7 Answer
Given that the quadratic function is,
f(x)=−(x−6)2+4
The given function is in vertex form.
Now we have to convert the vertex form into the standard form.
Consider the given function
f(x)=−(x−6)2+4
=−(x2+36−2(x)(6))+4
=−x2−36+12x+4
=−x2+12x−32
The standard form of the equation f(x)=−(x−6)2+4 is −x2+12x−32.
Page 272 Exercise 8 Answer
Given that the quadratic function is,
f(x)=−1/2(x−10)2−12
The given function is in vertex form.
Now we have to convert the vertex form into the standard form.
Consider the given function
f(x)=−1/2(x−10)2−12
=−1/2(x2+100−2(x)(10))−12
=−1/2x2−50+10x−12
=−1/2x2+10x−62
The standard form of the equation f(x)=−1/2(x−10)2−12 is −1/2 x2+10x−62.
Page 272 Exercise 9 Answer
Given that the quadratic function is,
f(x)=1/20(x+100)2+60
The given function is in vertex form.
Now we have to convert the vertex form into the standard form.
Consider the given function
f(x)=1/20(x+100)2+60
=1/20(x2+10000+2(x)(100))+60
=1/20x2+500+200x/20+60
=1/20x2+10x+560
The standard form of the equation f(x)=1/20(x+100)2+60 is 1/20x2+10x+560.
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 273 Exercise 10 Answer
Given that Cory is training his dog, Cocoa, for an agility competition.
Cocoa must jump through a hoop in the middle of a course. The center of the hoop is 8 feet from the starting pole.
The dog runs from the starting pole for 5 feet, jumps through the hoop, and lands 4 feet from the hoop.
When Cocoa is 1 foot from landing, Cory measures that she is 3 feet off the ground.
Now we have to write the function to represent Cocoa’s height in terms of her distance from the starting pole.
We will set a Cartesian coordinate system with the origin at the starting pole.
The height of Cocos jump will be a quadratic function written in factored form
h(x)=a(x−x1)(x−x2),
where x1 and x2 are the roots of the quadratic equation.
For the roots we know x1=5
x2=12 is the distance from the starting pole before and after the jump.
h(x)=a(x−5)(x−12)
Also, we know that 1 foot from landing Coco is 3 feet off the ground this means we have the following point of the graph (11,3) and if we replace these values in the above equation we get,
3=a(11−5)(11−12)
a(6)(−1)=3
a=−0.5
Thus the equation is : h(x)=−0.5(x−5)(x−12)
The function to represent Cocoa’s height in terms of her distance from the starting pole is h(x)=−0.5(x−5)(x−12).
Page 273 Exercise 11 Answer
Given that Sasha is training her dog, Bingo, to run across an arched ramp, which is in the shape of a parabola.
To help Bingo get across the ramp, Sasha places a treat on the ground where the arched ramp begins and one at the top of the ramp.
The treat at the top of the ramp is a horizontal distance of 2 feet from the first treat, and Bingo is 6 feet above the ground when he reaches the top of the ramp.
h=2
k=6
Now we have to write the function to represent Bingo’s height above the ground as he walks across the ramp in terms of his distance from the beginning of the ramp.
Now consider the general equation of the parabola,
f(x)=a(x−h)2+k
=a(x−2)2+6
Now at the point (0,0),
0=a(0−2)2+6
4a=−6
a=−6/4
a=−3/2
Therefore the function becomes,
f(x)=−3/2(x−2)2+6
The function to represent Bingo’s height above the ground as he walks across the ramp in terms of his distance from the beginning of the ramp is f(x)=−3/2(x−2)2+6.
How To Solve Skills Practice Exercise 2.1
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 274 Exercise 12 Answer
Given that Ella’s dog, Doug, is performing in a special tricks show.
Doug can fling a ball off his nose into a bucket 20 feet away.
Ella places the ball on Doug’s nose, which is 4 feet off the ground.
Doug flings the ball through the air into a bucket sitting on a 4-foot platform.
Halfway to the bucket, the ball is 10 feet in the air.
Here h=10
and k=10
Now we have to find the function to represent the height of the ball in terms of its distance from Doug.
Now consider the general equation of the parabola,
f(x)=a(x−h)2+k
=a(x−10)2+10
Now at the point (0,2),
2=a(0−10)2+10
2=100a+10
100a=−8
a=−8/100
a=−0.08
Therefore the function becomes,
f(x)=−0.08(x−10)2+10
The function to represent the height of the ball in terms of its distance from Doug is f(x)=−0.08(x−10)2+10.
Page 274 Exercise 13 Answer
Given that a spectator in the crowd throws a treat to one of the dogs in a competition.
The spectator throws the treat from the bleachers 19 feet above ground.
The treat amazingly flies 30 feet and just barely crosses over a hoop which is 7.5 feet tall.
The dog catches the treat 6 feet beyond the hoop when his mouth is 1 foot from the ground.
The equation is x2+7.5x+30.
Therefore, x2+7.5x+30.
Algebra Ii Chapter 2 Exercise 2.1 Answer Key
Carnegie Learning Algebra II Student Skills Practice 1st Edition Chapter 2 Exercise 2.1 Skills Practice Page 275 Exercise 14 Answer
Given that Hector’s dog, Ginger, competes in a waterfowl jump.
She jumps from the edge of the water, catches a toy duck at a horizontal distance of 10 feet from the edge of the water and a height of 2 feet above the water, and lands in the water at a horizontal distance of 15 feet from the edge of the water.
Here x1=0
and x2=15
Now we have to find the function to represent the height of Ginger’s jump in terms of her horizontal distance.
Consider the general function of factored form
f(x)=a(x−x1)(x−x2)
=a(x−0)(x−15)
=ax(x−15)
At the point (10,2),
2=a10(10−15)
2=10a(−5)−50a=2
a=−2/50
a=−1/25
Now consider the figure,

Therefore the function becomes,
f(x)=−1/25x(x−15)
The function to represent the height of Ginger’s jump in terms of her horizontal distance is −1/25x(x−15).
Page 272 Exercise 15 Answer
Given that 7 feet high with a speed of 18 feet per second.
The aim is to find the equation.
Assume that x per second.
P=7+18x
Therefore, the expression is 7+18x.
